Let ABCD be a square of side 2a. Find the coordinates of the vertices of this square when (i) A coincides with the origin and AB and AD are along OX and OY respectively. (ii) The centre of the square is at the origin and coordinate axes are parallel to the sides AB and AD respectively
To solve the problem, we will find the coordinates of the vertices of the square ABCD for both parts of the question step by step.
Part (i): A coincides with the origin and AB and AD are along OX and OY respectively.
1. Identify the position of point A: Since point A coincides with the origin, its coordinates are:
A(0,0)
2. Determine the coordinates of point B: Since AB is along the OX axis and the side length of the square is 2a, point B will be to the right of point A. Therefore, the coordinates of point B are:
B(2a,0)
3. Determine the coordinates of point D: Since AD is along the OY axis, point D will be above point A. Thus, the coordinates of point D are:
D(0,2a)
4. Determine the coordinates of point C: Point C is diagonally opposite to point A. Therefore, it will be at the coordinates:
C(2a,2a)
5. Summary of coordinates: The coordinates of the vertices of the square ABCD are:
- A(0,0)
- B(2a,0)
- C(2a,2a)
- D(0,2a)
Part (ii): The center of the square is at the origin and coordinate axes are parallel to the sides AB and AD respectively.
1. Identify the center of the square: The center of the square is at the origin, which means the coordinates of the center are:
O(0,0)
2. Determine the coordinates of point A: Since the side length of the square is 2a, each half will be a. Point A will be at:
A(−a,a)
3. Determine the coordinates of point B: Point B will be to the right of point A, thus its coordinates are:
B(a,a)
4. Determine the coordinates of point D: Point D will be above point A, thus its coordinates are:
D(−a,−a)
5. Determine the coordinates of point C: Point C will be below point B, thus its coordinates are:
C(a,−a)
6. Summary of coordinates: The coordinates of the vertices of the square ABCD are:
- A(−a,a)
- B(a,a)
- C(a,−a)
- D(−a,−a)
Final Summary of Coordinates:
- For part (i):
- A(0,0)
- B(2a,0)
- C(2a,2a)
- D(0,2a)
- For part (ii):
- A(−a,a)
- B(a,a)
- C(a,−a)
- D(−a,−a)
A(0,0)
B(2a,0)
D(0,2a)
C(2a,2a)
- A(0,0)
- B(2a,0)
- C(2a,2a)
- D(0,2a)
O(0,0)
A(−a,a)
B(a,a)
D(−a,−a)
C(a,−a)
- A(−a,a)
- B(a,a)
- C(a,−a)
- D(−a,−a)
- For part (i):
- A(0,0)
- B(2a,0)
- C(2a,2a)
- D(0,2a)
- A(−a,a)
- B(a,a)
- C(a,−a)
- D(−a,−a)
Topper's Solved these Questions
Similar Questions
Find the coordinates of the vertices of a square inscribed in the triangle with vertices A(0,0),B(2,1)andC(3,0), given that two of its vertices are on the side AC.
View SolutionFind the coordinates of the vertices of a square inscribed in the triangle with vertices A(0,0),B(3,0)andC(2,1); given that two of its vertices are on the side AB.
View Solution
Knowledge Check
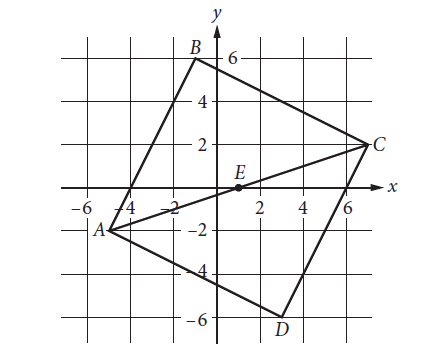
In the xy-plane above, ABCD is a square and point E is the center of the square. The coordinates of points C and E are (7, 2) and (1, 0), respectively. Which of the following is an equation of the line that passes through points B and D ?Ay=−3x−1By=−3(x−1)Cy=−13x+4Dy=−13x−1
The center of a square is at the origin and its one vertex is A(2,1). Find the coordinates of the other vertices of the square.
View SolutionThe center of a square is at the origin and its one vertex is A(2,1). Find the coordinates of the other vertices of the square.
View SolutionThe center of a square is at the origin and its one vertex is A(3,2). Find the coordinates of the other vertices of the square.
View SolutionLet the circumcentre of ΔABC is S(−1,0) and the midpoints of the sides AB and AC are E(1,−2) and F(−2,−1) respectively, then the coordinates of A are
View SolutionAB is a side of a regular hexagon ABCDEF and is of length a with A as the origin and AB and AE as the x-axis andy-axis respectively. Find the equation of lines AC,AF and BE
View SolutionAB is a side of a regular hexagon ABCDEF and is of length a with A as the origin and AB and AE as the x-axis andy-axis respectively. Find the equation of lines AC,AF and BE
View SolutionFind the area, in square centimetres, of a square whose side is (i) 2.6 cm (ii) 1.2 dm
View Solution
