Two lines AB and CD intersect at O. If ∠AOC+∠COB+∠BOD=2700 , find the measures of ∠AOC, ∠COB, ∠BOD and ∠DOA.
To solve the problem, we need to find the measures of angles ∠AOC, ∠COB, ∠BOD, and ∠DOA given that:
∠AOC+∠COB+∠BOD=270∘
1. Understand the Geometry:
We have two lines AB and CD intersecting at point O. This creates four angles around point O: ∠AOC, ∠COB, ∠BOD, and ∠DOA.
2. Use the Linear Pair Property:
Since AB and CD are intersecting lines, we know that:
∠AOC+∠BOC=180∘
This is because they form a linear pair.
3. Set Up the Equation:
We are given:
∠AOC+∠COB+∠BOD=270∘
We can substitute ∠BOC from the linear pair property into this equation. We know that:
∠BOC=180∘−∠AOC
4. Substitute and Rearrange:
Substitute ∠BOC into the equation:
∠AOC+(180∘−∠AOC)+∠BOD=270∘
This simplifies to:
180∘+∠BOD=270∘
5. Solve for ∠BOD:
Now, isolate ∠BOD:
∠BOD=270∘−180∘=90∘
6. Find ∠AOC:
Since ∠AOC=∠BOD (as they are opposite angles), we have:
∠AOC=90∘
7. Find ∠COB:
Now, use the linear pair property again:
∠AOC+∠BOC=180∘
Substitute ∠AOC:
90∘+∠BOC=180∘
Thus,
∠BOC=180∘−90∘=90∘
8. Find ∠DOA:
Since ∠AOD=∠BOC (again, as they are opposite angles), we have:
∠DOA=90∘
Summary of Angles:
- ∠AOC=90∘
- ∠COB=90∘
- ∠BOD=90∘
- ∠DOA=90∘
We have two lines AB and CD intersecting at point O. This creates four angles around point O: ∠AOC, ∠COB, ∠BOD, and ∠DOA.
Since AB and CD are intersecting lines, we know that:
∠AOC+∠BOC=180∘
This is because they form a linear pair.
We are given:
∠AOC+∠COB+∠BOD=270∘
We can substitute ∠BOC from the linear pair property into this equation. We know that:
∠BOC=180∘−∠AOC
Substitute ∠BOC into the equation:
∠AOC+(180∘−∠AOC)+∠BOD=270∘
This simplifies to:
180∘+∠BOD=270∘
Now, isolate ∠BOD:
∠BOD=270∘−180∘=90∘
Since ∠AOC=∠BOD (as they are opposite angles), we have:
∠AOC=90∘
Now, use the linear pair property again:
∠AOC+∠BOC=180∘
Substitute ∠AOC:
90∘+∠BOC=180∘
Thus,
∠BOC=180∘−90∘=90∘
Since ∠AOD=∠BOC (again, as they are opposite angles), we have:
∠DOA=90∘
- ∠AOC=90∘
- ∠COB=90∘
- ∠BOD=90∘
- ∠DOA=90∘
Topper's Solved these Questions
Similar Questions
Two straight lines AB and CD intersect one another at the point O. If ∠AOC+∠COB+∠BOD=2740, then ∠AOD=
View SolutionIn Figure, lines AB and CD intersect at O. If ∠AOC+ ∠BOE=700and ∠BOD=400, find ∠BOE and reflex ∠COE
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
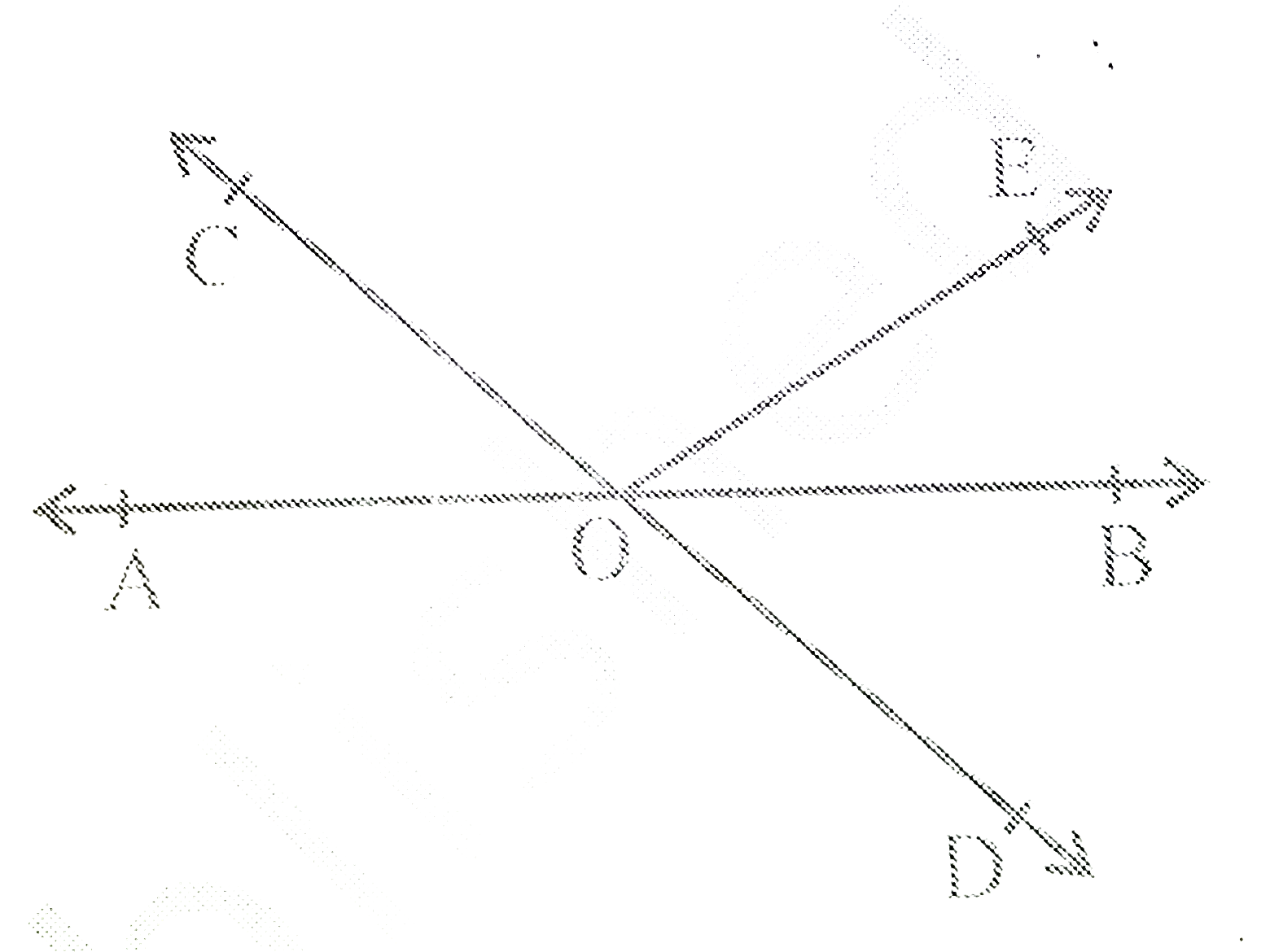
View SolutionIn Figure, find x. Further find ∠BOC, ∠COD and ∠AOD.
View SolutionIn Figure, lines AB, CD and EF intersect at O. Find the measures of ∠AOC, ∠COF, ∠DOE and ∠BOF .
View SolutionIn Fig.25, find ∠x. Further find ∠BOC, ∠COD and ∠AOD.
View SolutionAB and CD are diameters of a circle with centre O. If ∠OBD=500, find ∠AOC
View SolutionIn ABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 1200 , then find the measure of ∠A
View SolutionIn a parallelogram ABCD, the diagonals bisect each other at O. If ∠ABC=300, ∠BDC=100and ∠CAB=700, Find: ∠DAB, ∠ADC, ∠BCD, ∠AOD, ∠DOC, ∠BOC, ∠AOB, ∠ACD, ∠CAB, ∠ADB
View SolutionThe diagonals of a rectangle ABCD intersect in O. If ∠BOC=680, find ∠ODA.
View Solution
