A kite is flying at a height of 60m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60∘ . Find the length of the string assuming that there is no slack in the string.
To find the length of the string attached to the kite flying at a height of 60 m above the ground, we can use trigonometry. Here’s a step-by-step solution:
Step 1: Understand the Problem
We have a kite flying at a height (AB) of 60 m. The string (AC) makes an angle of 60 degrees with the ground (point C). We need to find the length of the string AC.
Step 2: Draw a Right Triangle
We can visualize the situation as a right triangle where:
- Point A is the kite,
- Point B is the point directly below the kite on the ground,
- Point C is the point on the ground where the string is tied.
Here, AB = 60 m (height of the kite), and angle CAB = 60 degrees.
Step 3: Use the Sine Function
In the right triangle ABC, we can use the sine function:
sin(θ)=OppositeHypotenuse
Here, θ=60∘, the opposite side is AB (60 m), and the hypotenuse is AC (the length of the string).
So, we can write:
sin(60∘)=ABAC
Substituting the known values:
sin(60∘)=60AC
Step 4: Substitute the Value of Sine
We know that:
sin(60∘)=√32
So, we can substitute this into our equation:
√32=60AC
Step 5: Cross-Multiply to Solve for AC
Cross-multiplying gives us:
√3⋅AC=2⋅60
√3⋅AC=120
Step 6: Isolate AC
Now, we can solve for AC:
AC=120√3
Step 7: Rationalize the Denominator
To rationalize the denominator, multiply the numerator and denominator by √3:
AC=120⋅√3√3⋅√3=120√33
AC=40√3
Step 8: Final Answer
Thus, the length of the string AC is:
Length of the string=40√3 m
We have a kite flying at a height (AB) of 60 m. The string (AC) makes an angle of 60 degrees with the ground (point C). We need to find the length of the string AC.
We can visualize the situation as a right triangle where:
- Point A is the kite,
- Point B is the point directly below the kite on the ground,
- Point C is the point on the ground where the string is tied.
In the right triangle ABC, we can use the sine function:
sin(θ)=OppositeHypotenuse
Here, θ=60∘, the opposite side is AB (60 m), and the hypotenuse is AC (the length of the string).
sin(60∘)=ABAC
Substituting the known values:
sin(60∘)=60AC
We know that:
sin(60∘)=√32
So, we can substitute this into our equation:
√32=60AC
Cross-multiplying gives us:
√3⋅AC=2⋅60
√3⋅AC=120
Now, we can solve for AC:
AC=120√3
To rationalize the denominator, multiply the numerator and denominator by √3:
AC=120⋅√3√3⋅√3=120√33
AC=40√3
Thus, the length of the string AC is:
Length of the string=40√3 m
Topper's Solved these Questions
Similar Questions
The string of a kite is 100 metres long and it makes an angle of 60o with the horizontal. Find the height of the kite, assuming that there is no slack in the string.
View SolutionA kite is flying at a height of 75 metres from the ground level, attached to a string inclined at 60∘ to the horizontal. Find the length of the string to the nearest metre.
View SolutionThe length of a string between a kite and a point on the ground is 90 metres. If the string makes an angle θ with the ground level such that tanθ=158, how high is the kite? Assume that there is no slack in the string.
View SolutionA kite is flying at a height of 30m from the ground. The length of string from the kite to the ground is 60m. Assuming that three is no slack in the string, the angle of elevation of the kite at the ground is: 450 (b) 300 (c) 600 (d) 900
View SolutionA kite is flying at a height of 30m from the ground. The length of string from the kite to the ground is 60m. Assuming that three is no slack in the string, the angle of elevation of the kite at the ground is: 450 (b) 300 (c) 600 (d) 900
View SolutionA kite is flying at a height of 30m from the ground. The length of string from the kite to the ground is 60m. Assuming that there is no slack in the string, the angle of elevation of the kite at the ground is: 450 (b) 300 (c) 600 (d) 900
View SolutionTwo masses A and B of 3kg and 2kg are connected by a long inextensible string which passes over a massless and frictionless pulley. Initially the height of both the masses from the ground is same and equal to 1 metre. When the masses are released. Mass A hits the ground and gets stuck to the ground. Consider the length of the string as large, so that the pulley does not obstruct the motion of masses A and B [g=10m/s2]
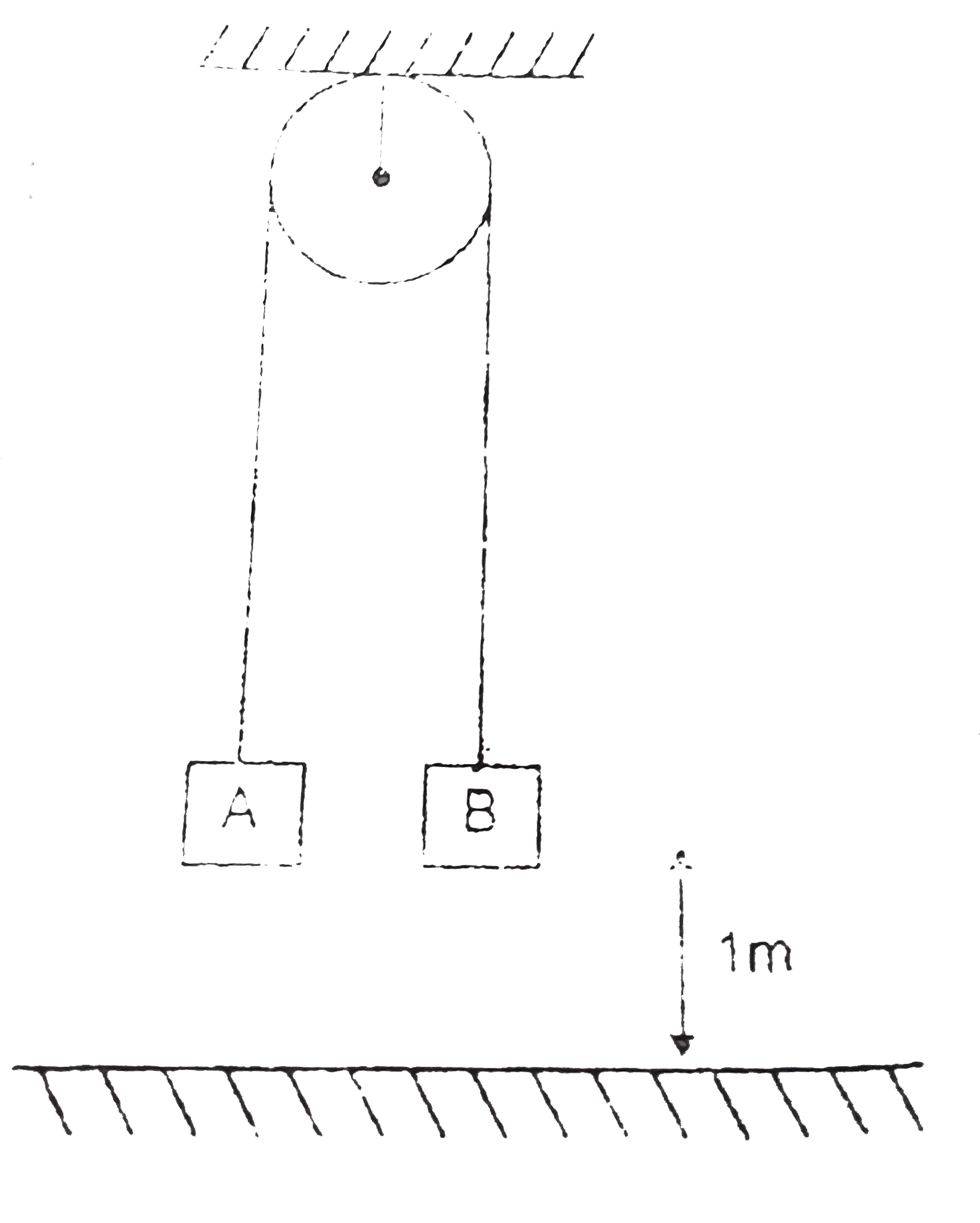
View SolutionA kite is attached to a string. Find the length of the string , when the height of the kite is 60 m and the string makes an angle 30∘ with the ground .
View SolutionThe angle of depression between a flying kite and 'a' point on the ground is 60 m. If the string makes 30∘.Find the height of the kite from the ground.
View SolutionAn aeroplane flying at a height 300 metre above the ground passes vertically above another plane at an instant when the angles of elevation of the two planes from the same point on the ground are 60∘ and 45∘ respectively. Then the height of the lower plane from the ground in metres is
View Solution