Figure, depicts an archery target marked with its five scoring areas from the centre outwards as Gold, Red, Blue Black and white. The diameter of the region representing Gold score is 21 cm and each of the other bands is 10.5 cm wide. Find the area of each of the five scoring regions.
To find the area of each of the five scoring regions on the archery target, we will follow these steps:
Step 1: Calculate the area of the Gold region.
- The diameter of the Gold region is given as 21 cm, so the radius R is:
R=212=10.5 cm
- The area A of a circle is given by the formula:
A=πR2
- Substituting the radius into the formula:
A=227×(10.5)2=227×110.25=346.5 cm2
Step 2: Calculate the area of the Red region.
- The radius of the Red region is the radius of the Gold region plus the width of the Red region:
Rred=10.5+10.5=21 cm
- The area of the Red region is the area of the outer circle minus the area of the inner circle (Gold region):
Ared=πR2red−πR2gold=π(212−(10.5)2)
- Calculating the areas:
Ared=227×(441−110.25)=227×330.75=1039.5 cm2
Step 3: Calculate the area of the Blue region.
- The radius of the Blue region is the radius of the Gold plus Red regions plus the width of the Blue region:
Rblue=21+10.5=31.5 cm
- The area of the Blue region:
Ablue=πR2blue−πR2red=π(31.52−212)
- Calculating the areas:
Ablue=227×(992.25−441)=227×551.25=1732.5 cm2
Step 4: Calculate the area of the Black region.
- The radius of the Black region:
Rblack=31.5+10.5=42 cm
- The area of the Black region:
Ablack=πR2black−πR2blue=π(422−31.52)
- Calculating the areas:
Ablack=227×(1764−992.25)=227×771.75=2425.5 cm2
Step 5: Calculate the area of the White region.
- The radius of the White region:
Rwhite=42+10.5=52.5 cm
- The area of the White region:
Awhite=πR2white−πR2black=π(52.52−422)
- Calculating the areas:
Awhite=227×(2756.25−1764)=227×992.25=3118.5 cm2
Summary of Areas:
- Area of Gold region: 346.5 cm2
- Area of Red region: 1039.5 cm2
- Area of Blue region: 1732.5 cm2
- Area of Black region: 2425.5 cm2
- Area of White region: 3118.5 cm2
- The diameter of the Gold region is given as 21 cm, so the radius R is:
R=212=10.5 cm
- The area A of a circle is given by the formula:
A=πR2
- Substituting the radius into the formula:
A=227×(10.5)2=227×110.25=346.5 cm2
- The radius of the Red region is the radius of the Gold region plus the width of the Red region:
Rred=10.5+10.5=21 cm
- The area of the Red region is the area of the outer circle minus the area of the inner circle (Gold region):
Ared=πR2red−πR2gold=π(212−(10.5)2)
- Calculating the areas:
Ared=227×(441−110.25)=227×330.75=1039.5 cm2
- The radius of the Blue region is the radius of the Gold plus Red regions plus the width of the Blue region:
Rblue=21+10.5=31.5 cm
- The area of the Blue region:
Ablue=πR2blue−πR2red=π(31.52−212)
- Calculating the areas:
Ablue=227×(992.25−441)=227×551.25=1732.5 cm2
- The radius of the Black region:
Rblack=31.5+10.5=42 cm
- The area of the Black region:
Ablack=πR2black−πR2blue=π(422−31.52)
- Calculating the areas:
Ablack=227×(1764−992.25)=227×771.75=2425.5 cm2
- The radius of the White region:
Rwhite=42+10.5=52.5 cm
- The area of the White region:
Awhite=πR2white−πR2black=π(52.52−422)
- Calculating the areas:
Awhite=227×(2756.25−1764)=227×992.25=3118.5 cm2
- Area of Gold region: 346.5 cm2
- Area of Red region: 1039.5 cm2
- Area of Blue region: 1732.5 cm2
- Area of Black region: 2425.5 cm2
- Area of White region: 3118.5 cm2
Topper's Solved these Questions
Similar Questions
Fig. 12.3 depicts an archery target marked with its five scoring areas from the centre outwards as Gold, Red, Blue, Black and White. The diameter of the region representing Gold score is 21 cm and each of the other bands is 10.5 cm wide. Find the area of each of the five scoring regions.
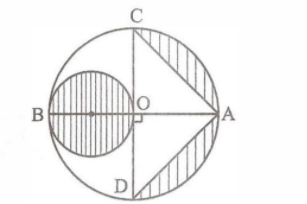
View SolutionIn the given figure, AB and CD are two diameters of circles ( with centre O) Perpendicular to each other and OD is the diameter of the smallest circle. If OA = 7cm, Find the area of the shaded region.
View SolutionIn the given figure, AB and CD are two diameters of circles ( with centre O) Perpendicular to each other and OD is the diameter of the smallest circle. If OA = 7cm, Find the area of the shaded region.
 View Solution
View SolutionIn figure arcs have been drawn with radii 14 cm each and with centres P, Q and R. Find the area of the shaded region .
 View Solution
View SolutionA wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as shown in Fig. . If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article.
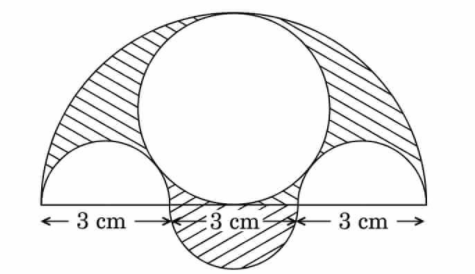
View SolutionThree semicircles each of diameter 3 cm, a circle of diameter 4·5 cm and a semicircle of radius 4·5 cm are drawn in the given figure. Find the area of the shaded region.
 View Solution
View SolutionThree semicircles each of diameter 3 cm, a circle of diameter 4·5 cm and a semicircle of radius 4·5 cm are drawn in the given figure. Find the area of the shaded region.
 View Solution
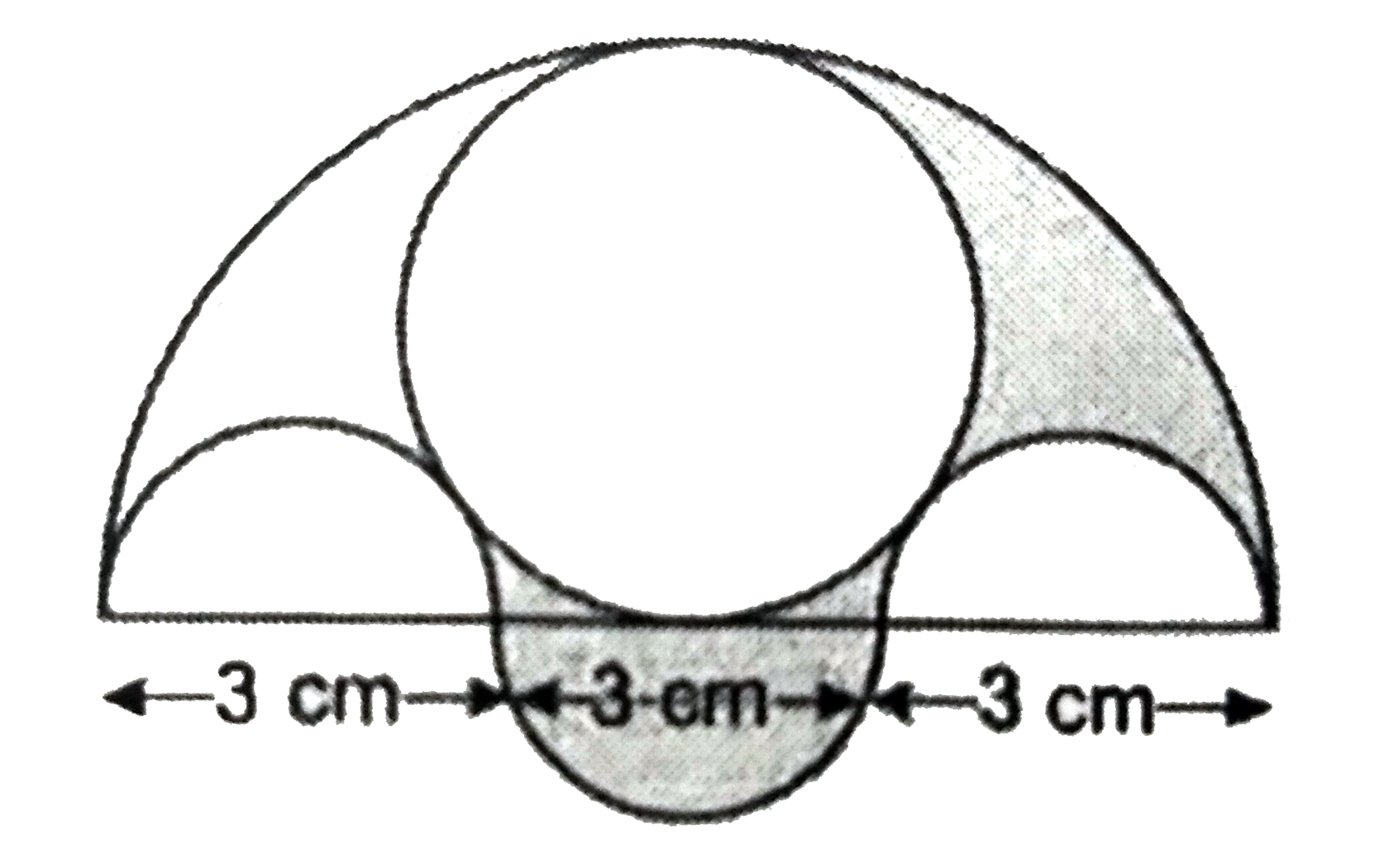
View SolutionThree semicircles each of diameter 3 cm, a circle of diameter 4.5 cm and a semicircle of radius 4.5 cm are drawn in the adjoining figure. Find the area of the shaded region.
 View Solution
View SolutionIf Figure - 4, AB and CD are two diameter of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. IF OA = 7 cm, then find the area of the shaded region.
 View Solution
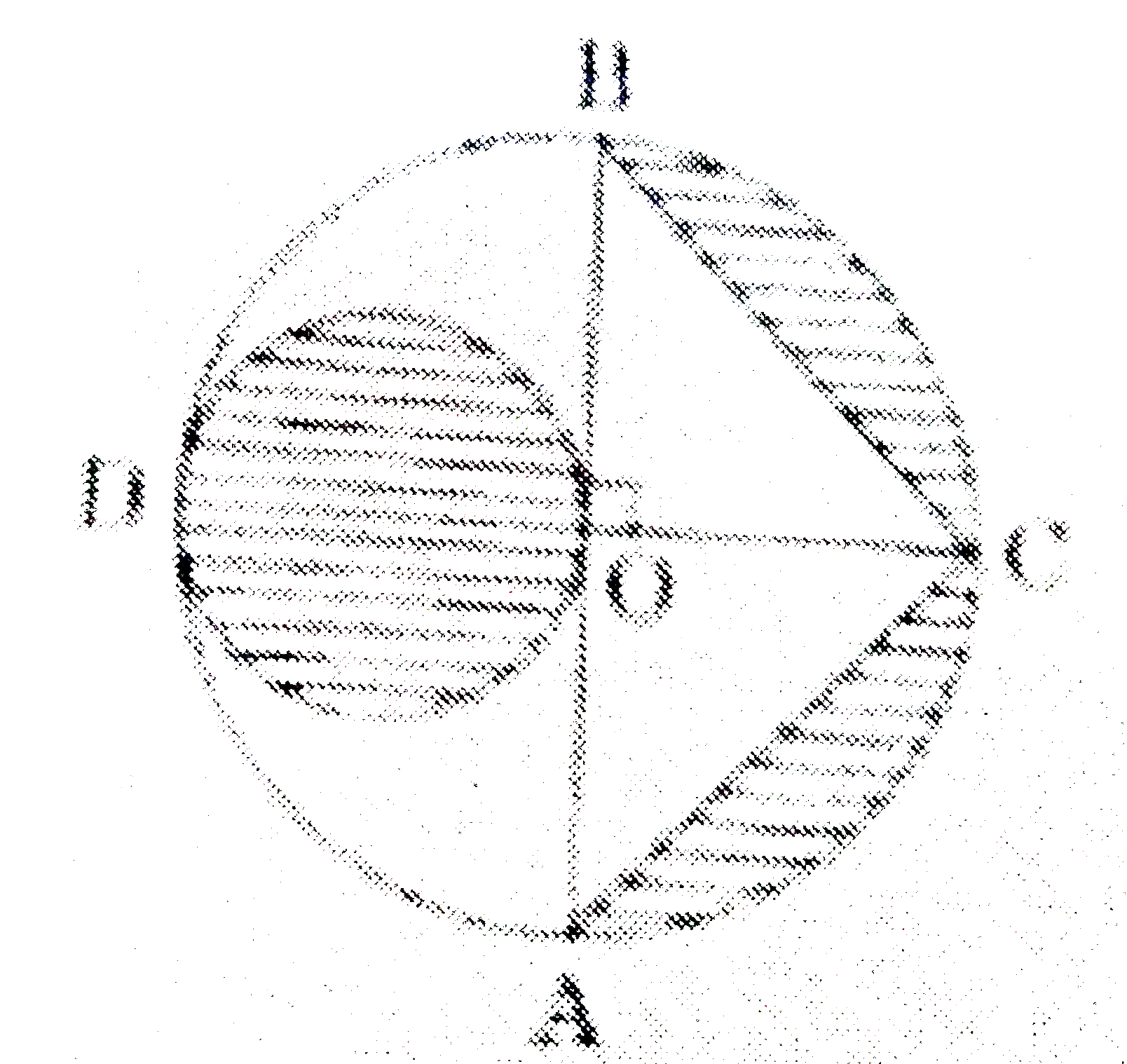
View SolutionIn Figure -4 AB, and CD are two diameters of a circle with centre O perpendiclualr to each other and OD is the diameter of the circle . If OA = 7 cm , then find the carea of the shaded region .
 View Solution
View Solution

