BC is a chord with centre O. A is a point on an arc BC . Prove that: ∠BAC−∠OBC= 90∘.
Step by step video & image solution for BC is a chord with centre O. A is a point on an arc BC . Prove that: ∠BAC−∠OBC= 90^@. by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
In ΔABC,D is any point on side BC. Prove that
AB+BC+CA>2AD
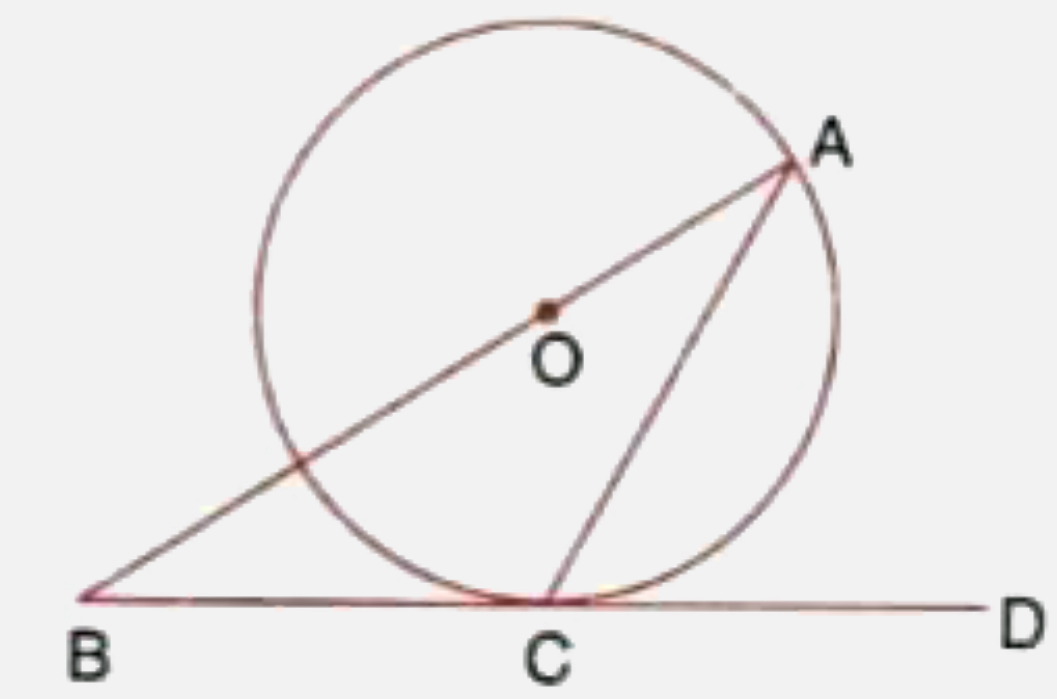
View SolutionThe given figure shows a circle with centre O and BCD is tangent to it at C. Show that
∠ACD+∠BAC=90∘
View SolutionTwo equal chord AB and CD of a circle with centre O, intersect each other at point P inside the circle.

Prove that:
BP=DP
View SolutionTwo tangent segments BC and BD are drawn to a circle with centre O and radius r such that ∠DBC=120∘. Prove that BO=2BC.
View SolutionBC is an equilateral triangle. A circle is drawn with centre A so that it cuts AB and AC at points M and N respectively. Prove that BN = CM
View SolutionIn the figure AB is the chord of a circle with centre O and DOC is a line segment such that BC=DO. If ∠C=20∘, find angle AOD.
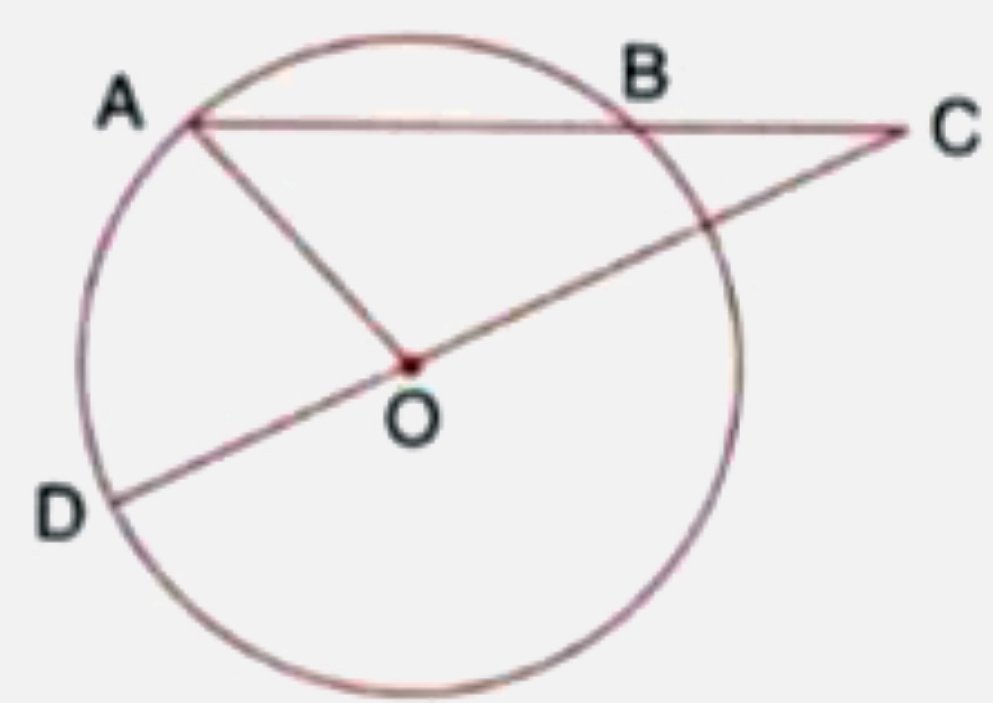
View SolutionChords AB and CD of a circle with centre O, intersect at a point E. If OE bisects angle AED, prove that chord AB = chord CD.
View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
View SolutionA ΔABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
View SolutionA Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
View Solution
