A beam is supported at the two ends and is uniformly loaded. The bending moment M at a distance x from one end is given by M=WL2x−W2x2 . Find the point at which M is maximum.
To find the point at which the bending moment M is maximum, we will follow these steps:
Step 1: Write down the expression for the bending moment
The bending moment M at a distance x from one end is given by:
M=WL2x−W2x2
Step 2: Differentiate the bending moment with respect to x
To find the maximum point, we need to differentiate M with respect to x:
dMdx=ddx(WL2x−W2x2)
Using the power rule of differentiation:
dMdx=WL2−Wx
Step 3: Set the derivative equal to zero
To find the critical points, we set the derivative equal to zero:
WL2−Wx=0
Solving for x:
Wx=WL2
x=L2
Step 4: Verify if it is a maximum
To confirm that this point is a maximum, we need to find the second derivative of M:
d2Mdx2=ddx(WL2−Wx)
Differentiating again:
d2Mdx2=−W
Since −W<0 (as W is a positive constant), this indicates that M is at a maximum at x=L2.
Conclusion
Thus, the bending moment M is maximum at the point:
x=L2
---
The bending moment M at a distance x from one end is given by:
M=WL2x−W2x2
To find the maximum point, we need to differentiate M with respect to x:
dMdx=ddx(WL2x−W2x2)
Using the power rule of differentiation:
dMdx=WL2−Wx
To find the critical points, we set the derivative equal to zero:
WL2−Wx=0
Solving for x:
Wx=WL2
x=L2
To confirm that this point is a maximum, we need to find the second derivative of M:
d2Mdx2=ddx(WL2−Wx)
Differentiating again:
d2Mdx2=−W
Since −W<0 (as W is a positive constant), this indicates that M is at a maximum at x=L2.
Thus, the bending moment M is maximum at the point:
x=L2
Topper's Solved these Questions
Similar Questions
A beam is supported at the two ends and is uniformly loaded. The bending moment M at a distance x from one end is given by M=Wx3−W3x3L2 . Find the point at which M is maximum.
View SolutionA beam is supported at the two ends and is uniformly loaded. The bending moment M at a distance x from one end is given by M=WL2x−W2x2 M=Wx3−W3x3L2 Find the point at which M is maximum in each case.
View SolutionA beam is supported at the two ends and is uniformly loaded. The bending moment M at a distance x from one end is given by (i)M=WL2x−W2x2 (ii) M=Wx3−W3x3L2 Find the point at which M is maximum in each case.
View SolutionThe density of a thin rod of length l varies with the distance x from one end as ρ=ρ0x2l2. Find the position of centre of mass of rod.
View SolutionThe electric foield at a point A on the perpendicular bisector of a uniformly charged wire of length l=3m and total charge q = 5 nC is x V/m. The distance of A from the centre of the wire is b = 2m. Find the value of x.
View SolutionA beam of weight W supports a block of weight W. The length of the beam is L. and weight is at a distance L4 from the left end of the beam. The beam rests on two rigid supports at its ends. Find the reactions of the supports.

View SolutionTwo uniform solid of masses m1 and m2 and radii r1 and r2 respectively, are connected at the ends of a uniform rod of length l and mass m. Find the moment of inertia of the system about an axis perpendicular to the rod and passing through a point at a distance of a from the centre of mass of the rod as shown in figure.

View SolutionA mass m is at a distance a from one end of a uniform rod of length l and mass M. Find the gravitational force on the mass due to the rod.

View SolutionA mass m is at a distance a from one end of a uniform rod of length l and mass M. Find the gravitational force on the mass due to tke rod.

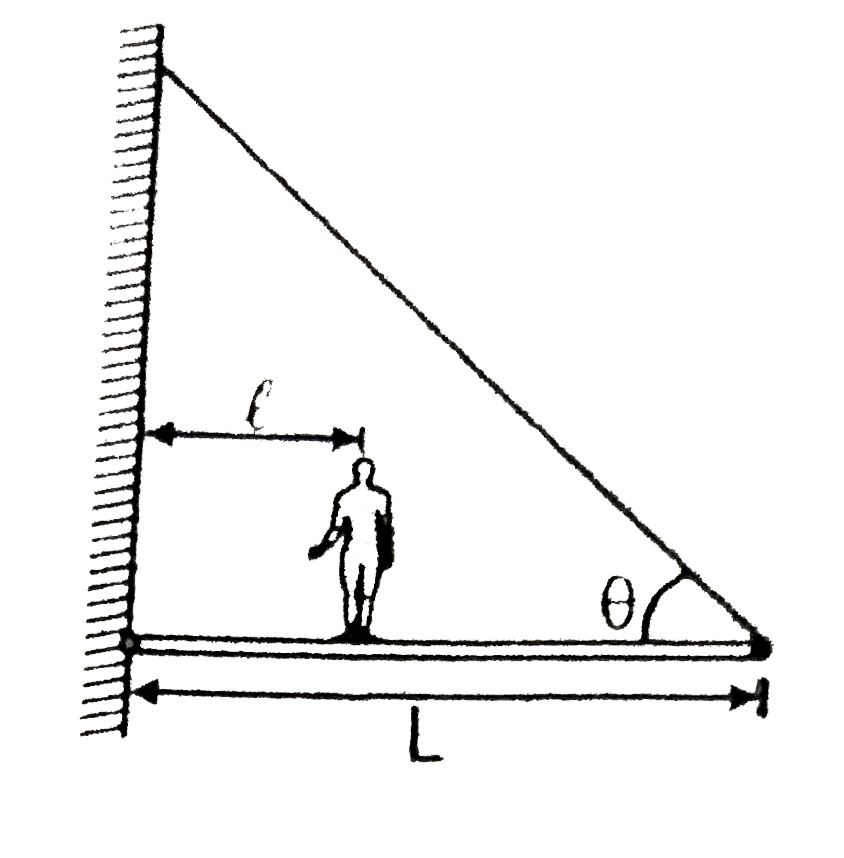
View SolutionA uniform horizontal beam of length L and mass M is attached to a wall by a pin connection. Its far end is supported by a cable that makes an angle θ with the horizontal . If a man of mass 'm' stands at a distance l from the wall , find the tension in the cable in equilibrium.
View Solution
