The electric foield at a point A on the perpendicular bisector of a uniformly charged wire of length l=3m and total charge q = 5 nC is x V/m. The distance of A from the centre of the wire is b = 2m. Find the value of x.
The correct Answer is:15
To find the electric field at point A on the perpendicular bisector of a uniformly charged wire, we can follow these steps:
Step 1: Understand the configuration
We have a uniformly charged wire of length l=3m and total charge q=5nC. The point A is located on the perpendicular bisector of the wire at a distance b=2m from the center of the wire.
Step 2: Set up the problem
The electric field due to a uniformly charged wire can be calculated by considering the contributions from each infinitesimal charge element along the wire. The wire can be treated as a line of charge.
Step 3: Identify the electric field components
At point A, the electric field contributions from the two ends of the wire will have horizontal components that add up and vertical components that cancel out due to symmetry.
Step 4: Calculate the distance from the charge element to point A
Let r be the distance from the midpoint of the wire to point A. The distance from the midpoint to either end of the wire is l2=32=1.5m. Therefore, we can use the Pythagorean theorem to find the distance R from a charge element at the midpoint to point A:
R=√(l2)2+b2=√(1.5)2+(2)2=√2.25+4=√6.25=2.5m
Step 5: Calculate the electric field due to one half of the wire
The electric field E due to a small charge dq at a distance R is given by:
dE=kdqR2
where k=9×109N m2/C2.
Step 6: Integrate over the length of the wire
Since the total charge q=5nC=5×10−9C, the linear charge density λ is:
λ=ql=5×10−93C/m
The total electric field at point A due to the entire wire can be calculated by integrating the contributions from each segment of the wire. However, due to symmetry, we can simplify the calculation by considering only one half of the wire and multiplying by 2.
Step 7: Calculate the horizontal component of the electric field
The horizontal component of the electric field Ex can be expressed as:
Ex=2⋅∫l20kdq⋅bR3R2
Substituting dq=λdx and integrating from 0 to 1.5m.
Step 8: Final calculation
After performing the integration and substituting the values, we find:
Ex=9V/m2=9V/m
Thus, the value of x is:
9
We have a uniformly charged wire of length l=3m and total charge q=5nC. The point A is located on the perpendicular bisector of the wire at a distance b=2m from the center of the wire.
The electric field due to a uniformly charged wire can be calculated by considering the contributions from each infinitesimal charge element along the wire. The wire can be treated as a line of charge.
At point A, the electric field contributions from the two ends of the wire will have horizontal components that add up and vertical components that cancel out due to symmetry.
Let r be the distance from the midpoint of the wire to point A. The distance from the midpoint to either end of the wire is l2=32=1.5m. Therefore, we can use the Pythagorean theorem to find the distance R from a charge element at the midpoint to point A:
R=√(l2)2+b2=√(1.5)2+(2)2=√2.25+4=√6.25=2.5m
The electric field E due to a small charge dq at a distance R is given by:
dE=kdqR2
where k=9×109N m2/C2.
Since the total charge q=5nC=5×10−9C, the linear charge density λ is:
λ=ql=5×10−93C/m
The horizontal component of the electric field Ex can be expressed as:
Ex=2⋅∫l20kdq⋅bR3R2
Substituting dq=λdx and integrating from 0 to 1.5m.
After performing the integration and substituting the values, we find:
Ex=9V/m2=9V/m
9
Topper's Solved these Questions
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Part - IV
Explore 9 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise - 3 Part - I
Explore 33 Videos
- ELECTROSTATICSBook:RESONANCE ENGLISHChapter:ELECTROSTATICSExercise:Exercise - 2 Part - I
Explore 21 Videos
- ELECTROMAGNETIC INDUCTIONBook:RESONANCE ENGLISHChapter:ELECTROMAGNETIC INDUCTIONExercise:A.l.P
Explore 19 Videos
- EXPERIMENTAL PHYSICSBook:RESONANCE ENGLISHChapter:EXPERIMENTAL PHYSICSExercise:PART -II
Explore 10 Videos
Similar Questions
Find the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionFind the electric field at a point P on the perpendicular bisector of a uniformly charged rod. The length of the rod is L, the charge on it is Q and the distance of P from the centre of the rod is a.
View SolutionElectric field at a point of distance r from a uniformly charged wire of infinite length having linear charge density λ is directly proportional to
View SolutionA semicircular ring of radius 0.5 m is uniformly charged with a total charge of 1.5×10−9 coul. The electric potential at the centre of this ring is :
View SolutionAt a point 20cm from the centre of a uniformly charged dielectric sphere of radius 10cm, the electric field is 100V/m. The electric field at 3cm from the centre of the sphere will be
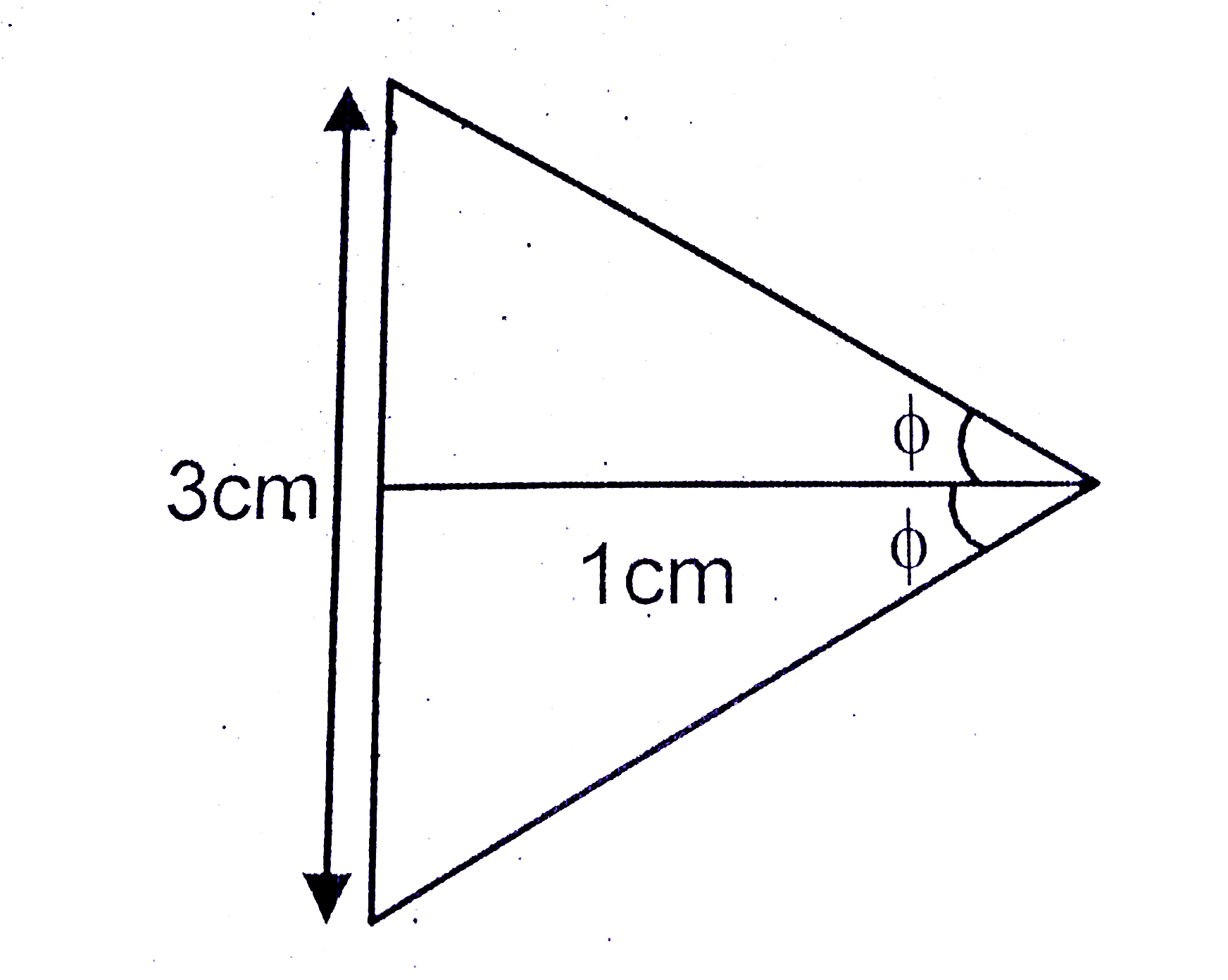
View SolutionA wire of length 3 cm has current 1 amp. Find magnetic field at a perpendicular distance a cm from centre of wire
View SolutionA charged disc of radius 3 m has charge density σ. Electric field at a distance of 4 m from its centre on axis is _______
View Solution(a) Using Gauss's law, derive an expression for the electric field intensity at any point outside a uniformly charged thin spherical shell of radius R and charge density σC/m2. Draw the field lines when the charge density of the sphere is (i) positive, (ii) negative.
(b) A uniformly charged conducting sphere of 2.5 m in diameter has a surface charge density of 100 μC/m2. Calculate the
(i) charge on the sphere
(ii) total electric flux passing through the sphere.
View SolutionA point charge Q is placed at the centre of a spherical conducting shell. A total charge of -q is placed on the shell. The magnitude of the electric field at point P1 at a distance R1 from the centre is X. The magnitude of the electric field due to induced charges at point P2 a distance R2 from the centre is Y. The values of X and Y are respectively.

View SolutionThe electric field due to a point charge at a distance 6 m from it is 630 N/C. The magnitude of the charge is
View Solution