Find the mean variance and standard deviation of the following probability distribution xi:a b pi:p q Where p+q=1.
To find the mean, variance, and standard deviation of the given probability distribution, we follow these steps:
Step 1: Define the Mean
The mean E(X) of a discrete random variable is calculated using the formula:
E(X)=∑xi⋅pi
where xi are the values of the random variable and pi are the corresponding probabilities.
Step 2: Calculate the Mean
Given:
- x1=a, p1=p
- x2=b, p2=q
Using the formula:
E(X)=a⋅p+b⋅q
Since p+q=1, we can express q as 1−p:
E(X)=a⋅p+b⋅(1−p)=a⋅p+b−b⋅p=(a−b)⋅p+b
Step 3: Define E(X2)
Next, we need to calculate E(X2):
E(X2)=∑x2i⋅pi
Calculating E(X2):
E(X2)=a2⋅p+b2⋅q
Substituting q:
E(X2)=a2⋅p+b2⋅(1−p)=a2⋅p+b2−b2⋅p=(a2−b2)⋅p+b2
Step 4: Calculate the Variance
The variance Var(X) is given by:
Var(X)=E(X2)−(E(X))2
Substituting the values we calculated:
Var(X)=((a2−b2)⋅p+b2)−((a−b)⋅p+b)2
Step 5: Expand and Simplify the Variance
Expanding (E(X))2:
(E(X))2=((a−b)⋅p+b)2=(a−b)2⋅p2+2(a−b)⋅p⋅b+b2
Now substituting this back into the variance formula:
Var(X)=((a2−b2)⋅p+b2)−((a−b)2⋅p2+2(a−b)⋅p⋅b+b2)
The b2 terms will cancel out:
Var(X)=(a2−b2)⋅p−(a−b)2⋅p2−2(a−b)⋅p⋅b
Step 6: Standard Deviation
The standard deviation SD(X) is the square root of the variance:
SD(X)=√Var(X)
Final Results
- Mean: E(X)=(a−b)⋅p+b
- Variance: Var(X)=(a2−b2)⋅p−(a−b)2⋅p2−2(a−b)⋅p⋅b
- Standard Deviation: SD(X)=√Var(X)
The mean E(X) of a discrete random variable is calculated using the formula:
E(X)=∑xi⋅pi
where xi are the values of the random variable and pi are the corresponding probabilities.
Given:
- x1=a, p1=p
- x2=b, p2=q
E(X)=a⋅p+b⋅q
Since p+q=1, we can express q as 1−p:
E(X)=a⋅p+b⋅(1−p)=a⋅p+b−b⋅p=(a−b)⋅p+b
Next, we need to calculate E(X2):
E(X2)=∑x2i⋅pi
Calculating E(X2):
E(X2)=a2⋅p+b2⋅q
Substituting q:
E(X2)=a2⋅p+b2⋅(1−p)=a2⋅p+b2−b2⋅p=(a2−b2)⋅p+b2
The variance Var(X) is given by:
Var(X)=E(X2)−(E(X))2
Substituting the values we calculated:
Var(X)=((a2−b2)⋅p+b2)−((a−b)⋅p+b)2
Expanding (E(X))2:
(E(X))2=((a−b)⋅p+b)2=(a−b)2⋅p2+2(a−b)⋅p⋅b+b2
Now substituting this back into the variance formula:
Var(X)=((a2−b2)⋅p+b2)−((a−b)2⋅p2+2(a−b)⋅p⋅b+b2)
The b2 terms will cancel out:
Var(X)=(a2−b2)⋅p−(a−b)2⋅p2−2(a−b)⋅p⋅b
The standard deviation SD(X) is the square root of the variance:
SD(X)=√Var(X)
- Mean: E(X)=(a−b)⋅p+b
- Variance: Var(X)=(a2−b2)⋅p−(a−b)2⋅p2−2(a−b)⋅p⋅b
- Standard Deviation: SD(X)=√Var(X)
Topper's Solved these Questions
Similar Questions
Find the variance and standard deviation of the following distribution

View SolutionFind the variance and standard deviation for the following distribution.

View SolutionFind the mean and standard deviation of the following probability distribution: xi:2 3 4 pi:0.2 0.5 0.3
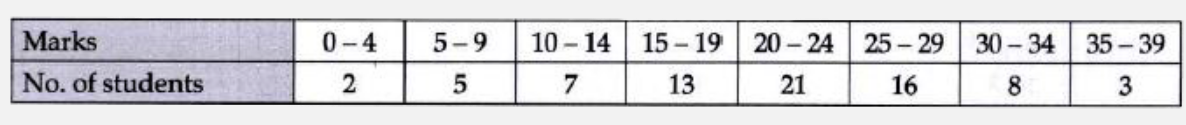
View SolutionFind the mean and standard deviation of the following frequency distribution :
View SolutionFind the mean and standard deviation of the following probability distribution: xi:−5 −4 1 2 pi:14 18 12 18
View SolutionFind the mean and standard deviation of the following probability distribution: xi: −1 0 1 2 3 pi: 0.3 0.1 0.1 0.3 0.2
View SolutionFind the mean and standard deviation of each of the following probability distribution: xi:1 3 4 5 pi:0.4 0.1 0.2 0.3
View SolutionFind the mean and standard deviation of the following probability distribution: xi: −2 −1 0 1 2 pi: 0.1 0.2 0.4 0.2 0.1
View SolutionFind the mean and standard deviation of the following probability distribution: xi: 1 2 3 4 pi: 0.4 0.3 0.2 0.1
View SolutionFind the mean and standard deviation of the following probability distribution:
xi:−3 −1 0 1 3
pi: 0.05 0.45 0.20 0.25 0.05
View Solution
