In the given figure, line l || m and a transversal n cuts them at P and Q respectively. If ∠1=750, find all other angles.
To solve the problem step by step, we start with the information given and apply the properties of angles formed by a transversal intersecting two parallel lines.
1. Identify the Given Information:
- We have two parallel lines: l and m.
- A transversal line n intersects these lines at points P and Q.
- We are given that ∠1=75∘.
2. Find ∠3:
- ∠1 and ∠3 are vertically opposite angles.
- Therefore, ∠3=∠1=75∘.
3. Find ∠2:
- ∠1 and ∠2 form a linear pair (they are on the same line).
- The sum of angles in a linear pair is 180∘.
- Thus, ∠1+∠2=180∘.
- Substituting the value of ∠1:
75∘+∠2=180∘
- Solving for ∠2:
∠2=180∘−75∘=105∘
4. Find ∠4:
- ∠2 and ∠4 are also vertically opposite angles.
- Therefore, ∠4=∠2=105∘.
5. Find ∠5:
- ∠1 and ∠5 are corresponding angles.
- Since l is parallel to m, corresponding angles are equal.
- Thus, ∠5=∠1=75∘.
6. Find ∠6:
- ∠2 and ∠6 are also corresponding angles.
- Therefore, ∠6=∠2=105∘.
7. Find ∠7:
- ∠3 and ∠7 are corresponding angles.
- Therefore, ∠7=∠3=75∘.
8. Find ∠8:
- ∠4 and ∠8 are corresponding angles.
- Therefore, ∠8=∠4=105∘.
Summary of Angles:
- ∠1=75∘
- ∠2=105∘
- ∠3=75∘
- ∠4=105∘
- ∠5=75∘
- ∠6=105∘
- ∠7=75∘
- ∠8=105∘
- We have two parallel lines: l and m.
- A transversal line n intersects these lines at points P and Q.
- We are given that ∠1=75∘.
- ∠1 and ∠3 are vertically opposite angles.
- Therefore, ∠3=∠1=75∘.
- ∠1 and ∠2 form a linear pair (they are on the same line).
- The sum of angles in a linear pair is 180∘.
- Thus, ∠1+∠2=180∘.
- Substituting the value of ∠1:
75∘+∠2=180∘
- Solving for ∠2:
∠2=180∘−75∘=105∘
- ∠2 and ∠4 are also vertically opposite angles.
- Therefore, ∠4=∠2=105∘.
- ∠1 and ∠5 are corresponding angles.
- Since l is parallel to m, corresponding angles are equal.
- Thus, ∠5=∠1=75∘.
- ∠2 and ∠6 are also corresponding angles.
- Therefore, ∠6=∠2=105∘.
- ∠3 and ∠7 are corresponding angles.
- Therefore, ∠7=∠3=75∘.
- ∠4 and ∠8 are corresponding angles.
- Therefore, ∠8=∠4=105∘.
- ∠1=75∘
- ∠2=105∘
- ∠3=75∘
- ∠4=105∘
- ∠5=75∘
- ∠6=105∘
- ∠7=75∘
- ∠8=105∘
Topper's Solved these Questions
Similar Questions
In the given figure, AB||CD and a transversal PQ cuts them at L and M respectively. If ∠QMD=1000, find all other angles.
View SolutionIn the given figure, AB and CD are parallel lines intersected by a transversal PQ at L and M respectively, If ∠CMQ=600 find all other angles in the figure.
View SolutionIn the given figure, line l || m and n is a transversal. If ∠1=400, find all the angles and check that all corresponding angles and alternate angles are equal.
View SolutionIn the given figure, AB and CD are parallel lines intersected by a transversal PQ at L and M respectively. If ∠LMD=350 find ∠PLA.
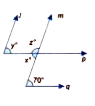
View SolutionIn the given figure l||mandp||q. Find the angle x,y, z
View SolutionIn Figure, AB and CD are parallel lines and transversal EF intersects them at P and Q respectively. If ∠APR=250, ∠RQC=300and ∠CQF=650, then
(a) x=550, y=400
(b) x=500, y=450
(c) x=600, y=350
(d) x=350, y=600
View SolutionIn the given figure, l||mandp||q. Find the angles x, y and z

View SolutionIn the adjoining figure, transversal n cuts parallel lines l and m. If ∠5=65∘, find all other angles

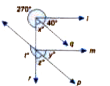
View SolutionIn the given figure, l||mandp||q. Find the angles x, y, z and t.
View SolutionIn Figure, RING is a parallelogram, if ∠R=700, find all other angles.
View Solution
