Find the dimensional formulae of
a the charge Q.
the potential V,
c. the capacitance C and
d the resistance R.
Some of the equations containing these quantities are <br>Q=It, U=VIt, Q=CV and V=RI,
where I denotes the electric current, t is time and U is energy.
To find the dimensional formulas for charge Q, potential V, capacitance C, and resistance R, we can use the provided equations and the known dimensional formulas for fundamental quantities.
1. Finding the Dimensional Formula for Charge Q:
- We use the equation Q=It, where I is electric current and t is time.
- The dimensional formula for electric current I is denoted as A (Ampere).
- The dimensional formula for time t is T.
- Therefore, the dimensional formula for charge Q is:
(Q)=(I)(t)=A⋅T=AT
2. Finding the Dimensional Formula for Potential V:
- We use the equation U=VIt, where U is energy.
- The dimensional formula for energy U can be derived from kinetic energy U=12mv2:
- The dimensional formula for mass m is M.
- The dimensional formula for velocity v is LT−1.
- Thus, the dimensional formula for energy U is:
(U)=M⋅(LT−1)2=ML2T−2
- Rearranging the equation U=VIt gives us:
V=UIt
- Substituting the dimensional formulas:
(V)=ML2T−2AT=ML2A−1T−3
3. Finding the Dimensional Formula for Capacitance C:
- We use the equation Q=CV.
- Rearranging gives:
C=QV
- We already found (Q)=AT and (V)=ML2A−1T−3.
- Therefore:
(C)=ATML2A−1T−3=AT⋅AML2T−3=A2T4ML2
- Rearranging gives:
(C)=M−1L−2T4A2
4. Finding the Dimensional Formula for Resistance R:
- We use the equation R=VI.
- Substituting the dimensional formulas:
(R)=ML2A−1T−3A=ML2A−2T−3
Summary of Dimensional Formulas:
- Charge Q: (Q)=AT
- Potential V: (V)=ML2A−1T−3
- Capacitance C: (C)=M−1L−2T4A2
- Resistance R: (R)=ML2A−2T−3
- We use the equation Q=It, where I is electric current and t is time.
- The dimensional formula for electric current I is denoted as A (Ampere).
- The dimensional formula for time t is T.
- Therefore, the dimensional formula for charge Q is:
(Q)=(I)(t)=A⋅T=AT
- We use the equation U=VIt, where U is energy.
- The dimensional formula for energy U can be derived from kinetic energy U=12mv2:
- The dimensional formula for mass m is M.
- The dimensional formula for velocity v is LT−1.
- Thus, the dimensional formula for energy U is:
(U)=M⋅(LT−1)2=ML2T−2
- Rearranging the equation U=VIt gives us:
V=UIt
- Substituting the dimensional formulas:
(V)=ML2T−2AT=ML2A−1T−3
- We use the equation Q=CV.
- Rearranging gives:
C=QV
- We already found (Q)=AT and (V)=ML2A−1T−3.
- Therefore:
(C)=ATML2A−1T−3=AT⋅AML2T−3=A2T4ML2
- Rearranging gives:
(C)=M−1L−2T4A2
- We use the equation R=VI.
- Substituting the dimensional formulas:
(R)=ML2A−1T−3A=ML2A−2T−3
- Charge Q: (Q)=AT
- Potential V: (V)=ML2A−1T−3
- Capacitance C: (C)=M−1L−2T4A2
- Resistance R: (R)=ML2A−2T−3
Topper's Solved these Questions
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Objective 1
6 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Objective 2
3 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- KINETIC THEORY OF GASESBOOK - HC VERMA ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - All Questions
106 Videos
Similar Questions
Find the dimensional formula of
(a) coefficient of viscosity η (b)charge q
(c ) potention V (d) capacitance C and
( e) resistance R
Some of the equations containing these quantities are
F=−ηA[ΔvΔl],q=It.U=VIt,q=CVandV=IR
where A denotes the area, v the velocity , l is the length ,I the electric current, t the time and U the energy .
View SolutionThe dimensional formula fo resistivity in terms of M,L,T and Q where Q stands for the dimensions of charge is
View SolutionIf C be the capacitance and V be the electric potential, then the dimensional formula of CV2 is
View SolutionObtain an expression for electric potential .V due to a point charge .Q at a distance r.
View SolutionFind the dimensional formulae of the following quantities:
a. the universal constant of gravitation G,
b. the surface tension S,
c. the thermal conductivity k and
d. the coeficient of viscosity η.
Some equation involving these quantities are
F=Gm1m2r2,S=ρgrh2,
Q=kA(θ2−θ1)tdandF=−ηAv2−v1x2−x1
where the symbols have their usual meanings.
View SolutionThe dimensionally correct expression for the resistance R among the following is
[P = electric power, l = electric current, t = time, V = volatge and E = electric energy]
View SolutionFind the equation a plane containing the line →r=t→a and perpendicular to the plane containing the line →r=u→band→r=v→c.
View SolutionFind the dimensions of
a. electric dipole moment p and
b. magnetic dipole moment M.
The defining equations are p=q.dandM=IA,
whre d is distance, A is area, q is charge and I is current.
View SolutionThe plates of a capacitor of capacitance C are given the charges Q1andQ2 as shown in figure. Now the switch is closed at t =0. Find the charges on plates after time t.
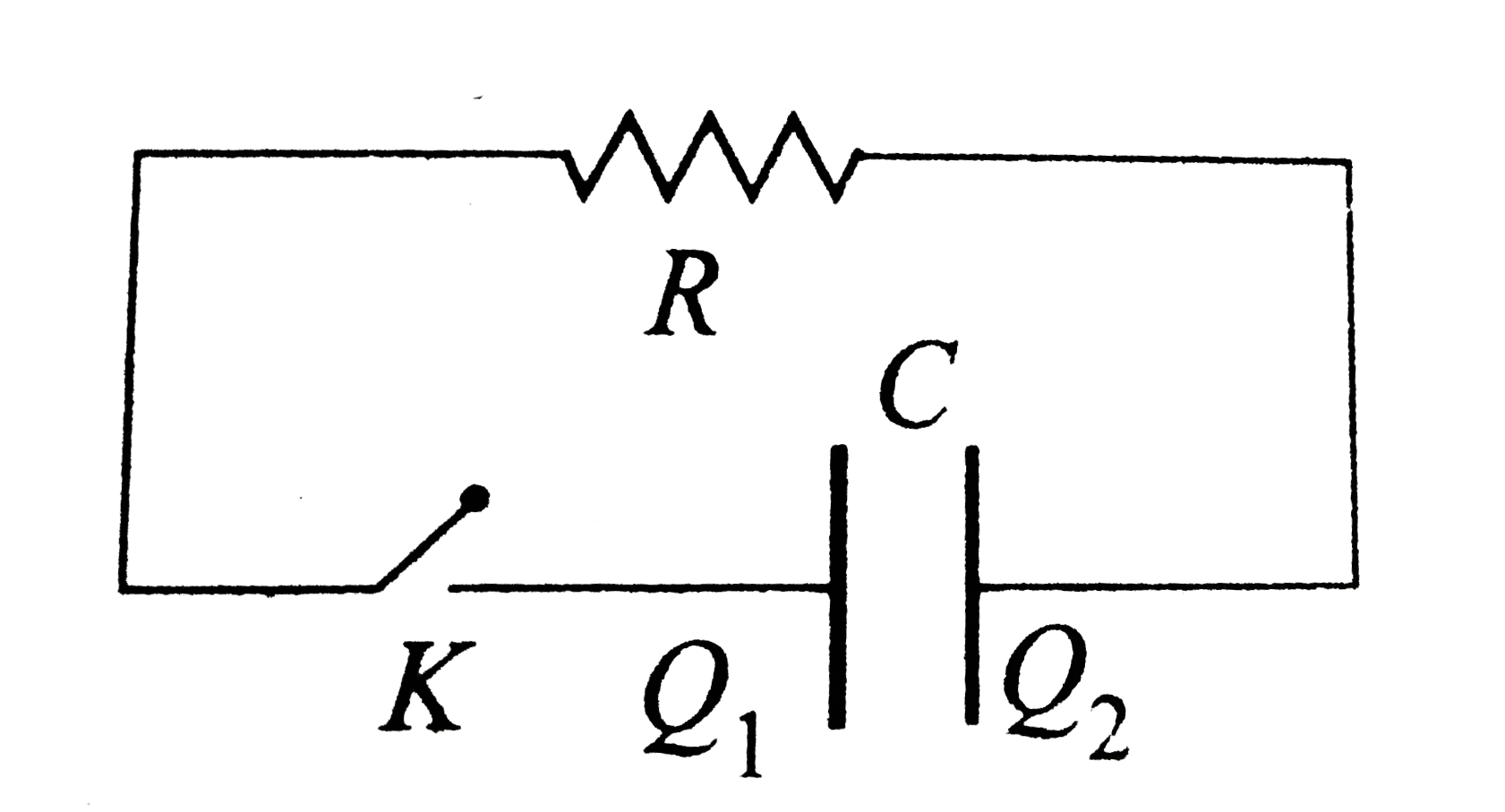
View SolutionFind potential difference across the plates AB if capacitance between the plates is C and area of each plate is equal and Q1,Q2(<Q1) are charges given to the plates
View Solution
