A body builder exerts a force of 150 N against a bullworker and compresses it by 20 cm. Caculate the spring constant of the spring in te bullworker.
To calculate the spring constant of the spring in the bullworker, we can use Hooke's Law, which states that the force exerted by a spring is directly proportional to the displacement or compression of the spring. The formula is given by:
F=kx
Where:
- F is the force applied (in Newtons),
- k is the spring constant (in Newtons per meter),
- x is the compression or extension of the spring (in meters).
1. Identify the Given Values:
- The force exerted by the bodybuilder, F=150N.
- The compression of the spring, x=20cm.
2. Convert the Compression to Meters:
- Since the standard unit for length in physics is meters, convert 20cm to meters:
x=20cm=20100m=0.2m
3. Rearrange Hooke’s Law to Solve for the Spring Constant k:
- From the formula F=kx, we can rearrange it to find k:
k=Fx
4. Substitute the Known Values into the Equation:
- Now, substitute F=150N and x=0.2m into the equation:
k=150N0.2m
5. Calculate the Spring Constant k:
- Performing the division:
k=1500.2=750N/m
Final Answer:
The spring constant of the spring in the bullworker is 750N/m.
---
- F is the force applied (in Newtons),
- k is the spring constant (in Newtons per meter),
- x is the compression or extension of the spring (in meters).
- The force exerted by the bodybuilder, F=150N.
- The compression of the spring, x=20cm.
- Since the standard unit for length in physics is meters, convert 20cm to meters:
x=20cm=20100m=0.2m
- From the formula F=kx, we can rearrange it to find k:
k=Fx
- Now, substitute F=150N and x=0.2m into the equation:
k=150N0.2m
- Performing the division:
k=1500.2=750N/m
The spring constant of the spring in the bullworker is 750N/m.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Questions for short Answer9videos
THE FORCES
- BOOK - HC VERMA ENGLISHCHAPTER - THE FORCESEXERCISE - Objective -27videos
THE FORCES
- BOOK - HC VERMA ENGLISHCHAPTER - SPECIFIC HEAT CAPACITIES OF GASESEXERCISE - All Questions75videos
SPECIFIC HEAT CAPACITIES OF GASES
- BOOK - HC VERMA ENGLISHCHAPTER - WAVE MOTION AND WAVES ON A STRINGEXERCISE - Question for short Answer7videos
WAVE MOTION AND WAVES ON A STRING
Similar Questions
Rajan exerts a force of 150 N in pulling a cart at a constant speed of 10 ms−1 .Calculate the power exerted.
View SolutionA block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
View SolutionA force of 25 N acts on a body of area of cross-section 20 cm2. Calculate the pressure exerted by the body in pascals.
View SolutionA block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s figure towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 N/m. Find the maximum compression of the spring.
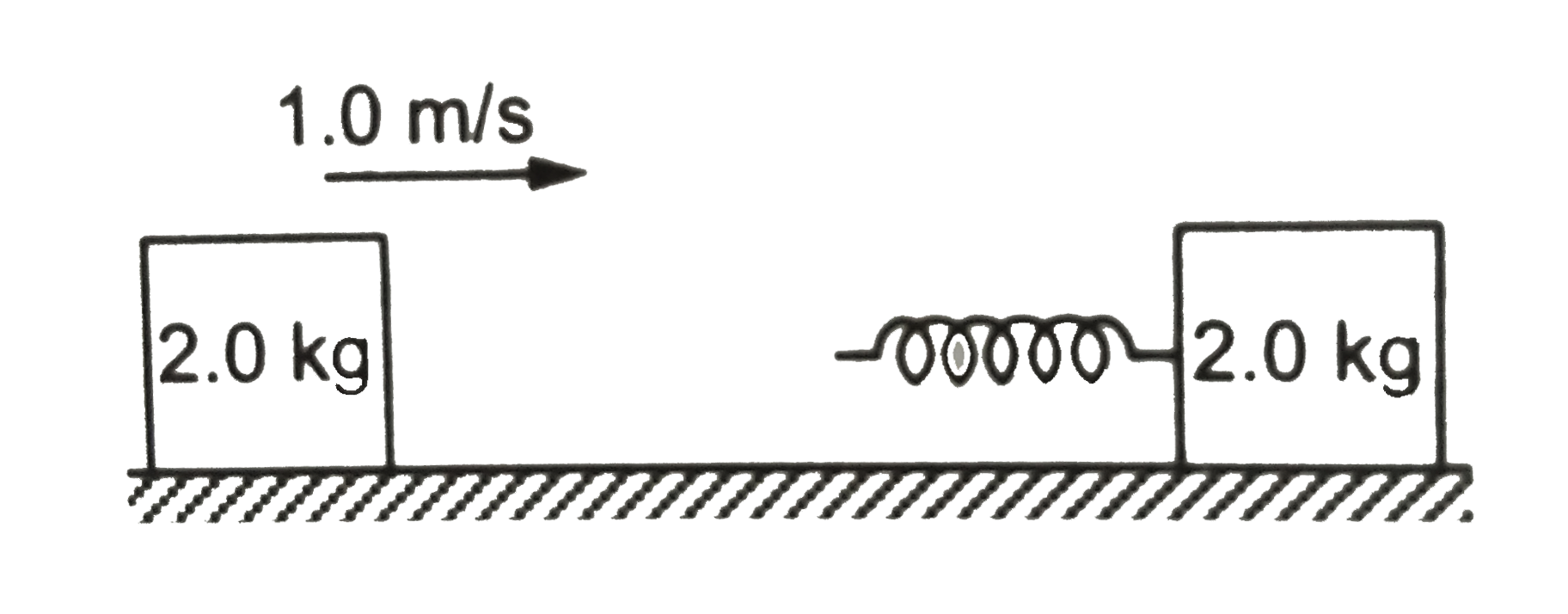 View Solution
View SolutionA block of mass m moving at a speed v compresses a spring through a distance x before its speed is halved. The spring constant of the spring is 6mv2nx2. Find value of n.
View SolutionA force of 10 N holds an ideal spring with a 20Nm spring constant in compression. The potential energy stored in the spring is
View SolutionA 16kg block moving on a frictionless horizontal surface with a velocity of 4m/s compresses an ideal spring and comes to rest. If the force constant of the spring be 100N/m, then how much is the spring commpressed ?
View Solution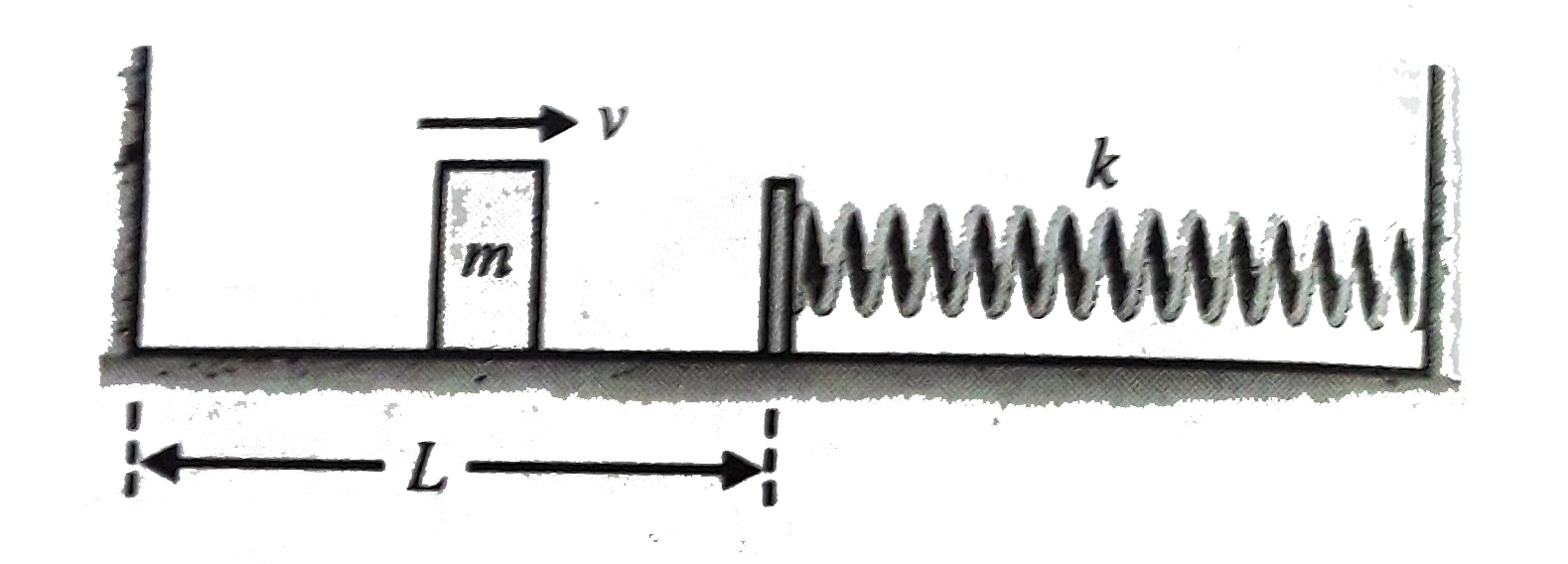
A spring having a spring constant k is fixed to a vertical wall as shown in Fig. A block of mass m moves with velocity v torards the spring from a parallel wall opposite to this wall. The mass hits the free end of the spring compressing it and is decelerated by the spring force and comes to rest and then turns the spring is decelerated by the spring force and comes to rest and then turns back till the spring acquires its natural length and contact with the spring is broken. In this process, it regains its angular speed in the opposite direction and makes a perfect elastice collision on the opposite left wall and starts moving with same speed as before towards right. The above processes are repeated and there is periodic oscillation.
Q. What is the time period of oscillation ?View SolutionA string 50 cm long is under a tension of 20N force. Caculate the frequency of fundamental mode given that mass of the string is 1 g.
View SolutionA string 50 cm long is under a tension of 20N force. Caculate the frequency of fundamental mode given that mass of the string is 1 g.
View Solution

