A 16kg block moving on a frictionless horizontal surface with a velocity of 4m/s compresses an ideal spring and comes to rest. If the force constant of the spring be 100N/m, then how much is the spring commpressed ?
1.7 m
1.6 m
1.4 m
1.8 m
The correct Answer is:B
To solve the problem, we will use the principle of conservation of energy. The kinetic energy of the block will be converted into the potential energy stored in the spring when the block comes to rest.
1. Identify the Given Values:
- Mass of the block, m=16kg
- Initial velocity of the block, v=4m/s
- Spring constant, k=100N/m
2. Calculate the Initial Kinetic Energy (KE):
The kinetic energy of the block when it is moving can be calculated using the formula:
KE=12mv2
Substituting the values:
KE=12×16kg×(4m/s)2=12×16×16=128J
3. Set Up the Equation for Potential Energy (PE) of the Spring:
When the block compresses the spring and comes to rest, all the kinetic energy is converted into potential energy stored in the spring:
PE=12kx2
Where x is the compression of the spring.
4. Equate the Kinetic Energy to the Potential Energy:
Since the block comes to rest, we can set the kinetic energy equal to the potential energy:
12kx2=KE
Substituting the values:
12×100N/m×x2=128J
5. Solve for x2:
50x2=128
x2=12850=2.56
6. Calculate x:
Taking the square root of both sides:
x=√2.56=1.6m
Final Answer:
The spring is compressed by 1.6m.
---
- Mass of the block, m=16kg
- Initial velocity of the block, v=4m/s
- Spring constant, k=100N/m
The kinetic energy of the block when it is moving can be calculated using the formula:
KE=12mv2
Substituting the values:
KE=12×16kg×(4m/s)2=12×16×16=128J
When the block compresses the spring and comes to rest, all the kinetic energy is converted into potential energy stored in the spring:
PE=12kx2
Where x is the compression of the spring.
Since the block comes to rest, we can set the kinetic energy equal to the potential energy:
12kx2=KE
Substituting the values:
12×100N/m×x2=128J
50x2=128
x2=12850=2.56
Taking the square root of both sides:
x=√2.56=1.6m
The spring is compressed by 1.6m.
Topper's Solved these Questions
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - IMPECCABLE
54 Videos
- WORK ENERGY AND POWER BOOK - VMC MODULES ENGLISHCHAPTER - WORK ENERGY AND POWER EXERCISE - ENABLE
50 Videos
- WAVE MOTIONBOOK - VMC MODULES ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE ADVANCED ARCHIVE LEVEL 2 (TRUE FALSE TYPE)
4 Videos
Similar Questions
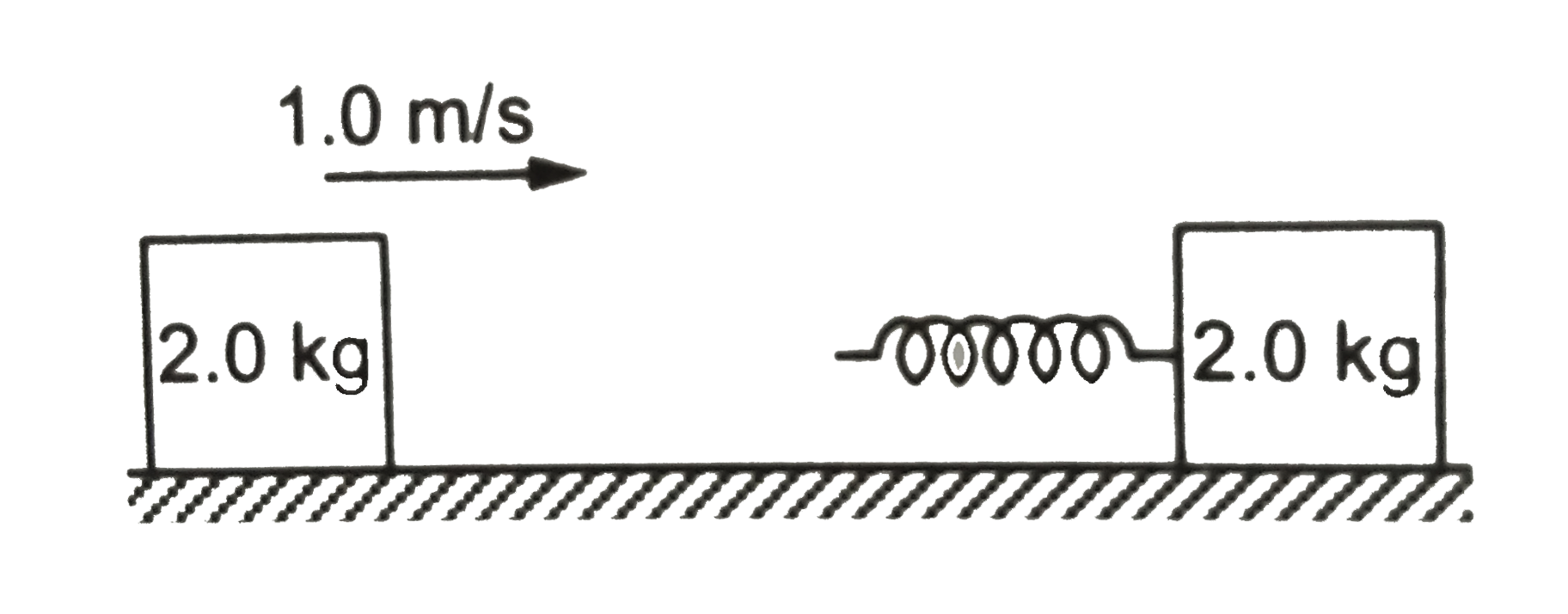
A block of mass 2.0 kg is moving on a frictionless horizontal surface with a velocity of 1.0 m/s figure towards another block of equal mass kept at rest. The spring constant of the spring fixed at one end is 100 N/m. Find the maximum compression of the spring.
View SolutionA solid sphere of mass 2 kg is rolling on a frictionless horizontal surface with velocity 6m/s. It collides on the free end of an ideal spring whose other end is fixed. The maximum compression produced in the spring will be
(Force constant of the spring = 36 N/m)
View SolutionA block of 200 g mass is dropped from a height of 2 m on to a spring and compress the spring to a distance of 50 cm. The force constant of the spring is
View SolutionA 3.0kg block slides on a frictionless horizontal surface, first moving to the left at 50m/s. It collides with a spring as it moves left, compresses the spring and is brought to rest momentarily. The body continues to be accelerated to the right by the force of compressed spring. Finally, the body moves to the right at 40m/s. The block remains in contact with the spring for 0.020s. What were the magnitude and direction of the impulse of the spring on the block? What was the spring's average force on the block?
View SolutionThe block of mass M moving on the frictionless horizontal surface collides with the spring constant k and compresses it by length L . The maximum momention of the block after collision is

View SolutionA plank of mass 5kg is placed on a frictionless horizontal plane. Further a block of mass 1kg is placed over the plank. A massless spring of natural length 2m is fixed to the plank by its one end. The other end of spring is compressed by the block by half of spring's natural length. They system is now released from the rest. What is the velocity of the plank when block leaves the plank? (The stiffness constant of spring is 100N/m)

View SolutionA planck of mass 5kg is placed on a frictionless horizontal plane. Further a block of mass 1kg is placed over the plank. A massless spring of natural length 2m is fixed to the plank by its one end. The other end of spring is compressed by the block by half of spring's natural length. They system is now released from the rest. What is the velocity of the plank when block leaves the plank? (The stiffness constant of spring is 100N/m)

View SolutionA planck of mass 5kg is placed on a frictionless horizontal plane. Further a block of mass 1kg is placed over the plank. A massless spring of natural length 2m is fixed to the plank by its one end. The other end of spring is compressed by the block by half of spring's natural length. They system is now released from the rest. What is the velocity of the plank when block leaves the plank? (The stiffness constant of spring is 100N/m)

View SolutionA block of mass m is initially moving to the right on a horizontal frictionless surface at a speed v. It then compresses a spring of spring constant k. At the instant when the kinetic energy of the block is equal to the potential energy of the spring, the spring is compressed a distance of :
View SolutionA block of mass m sliding n a smooth horizontal surface with velocity →v meets a long horizontal spring fixed ast one end and having spring constant k as shown n figure. Find the maximum compression of the spring. Will the velocity of the block be the same as v whenit comes back to the original position shown?

View Solution
