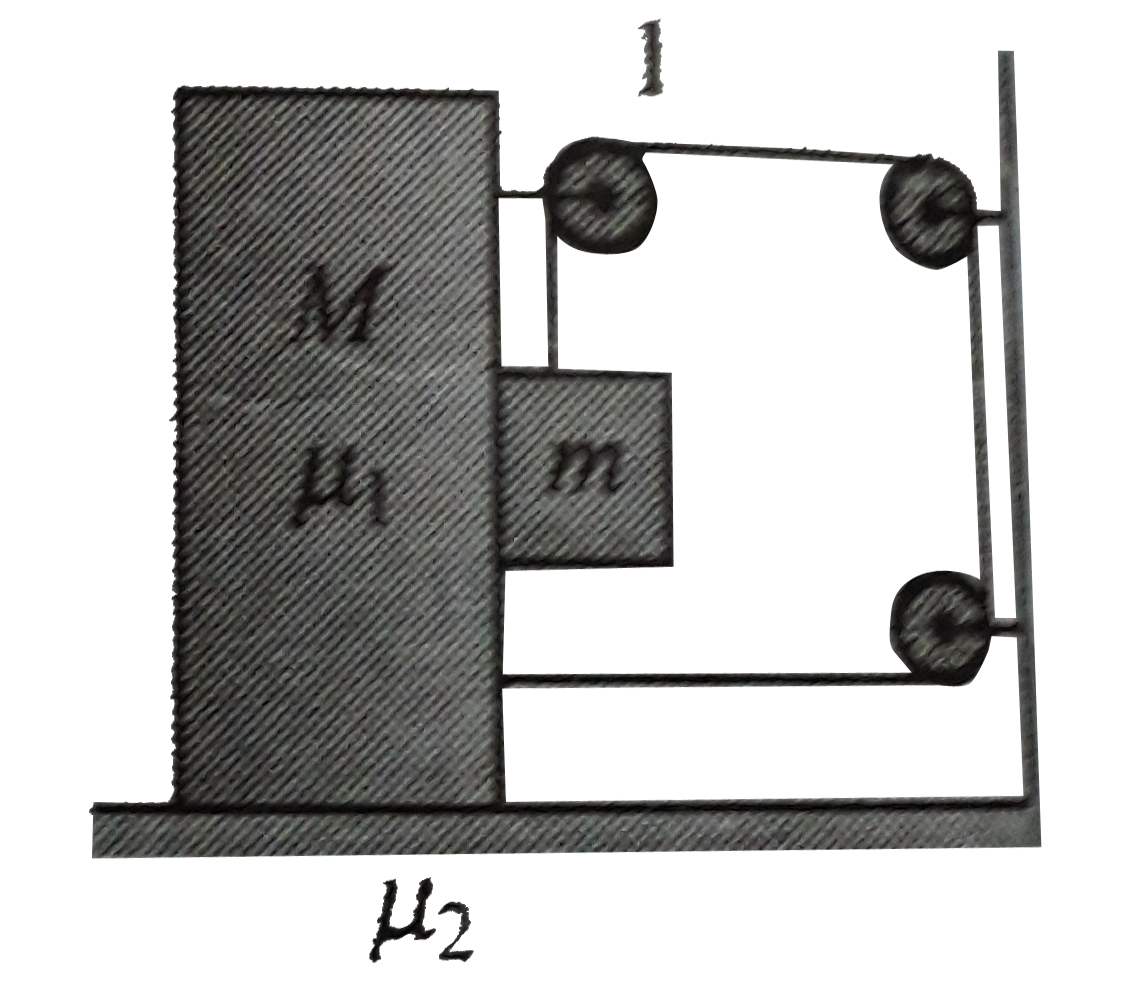
Find the acceleration of the block of mass M in the situation of figure. The coefficient of friction between the two blocks is μ1 and that between the bigger block and the ground is μ2.
The correct Answer is:A, B
Let the acceleration of the block M is a towards rilght. So the block m must to down with an acceleration 2a.
M it will also have acceleration a towards right. So it will experience two nertial forces as shown the free body diagram. From free body digram........1
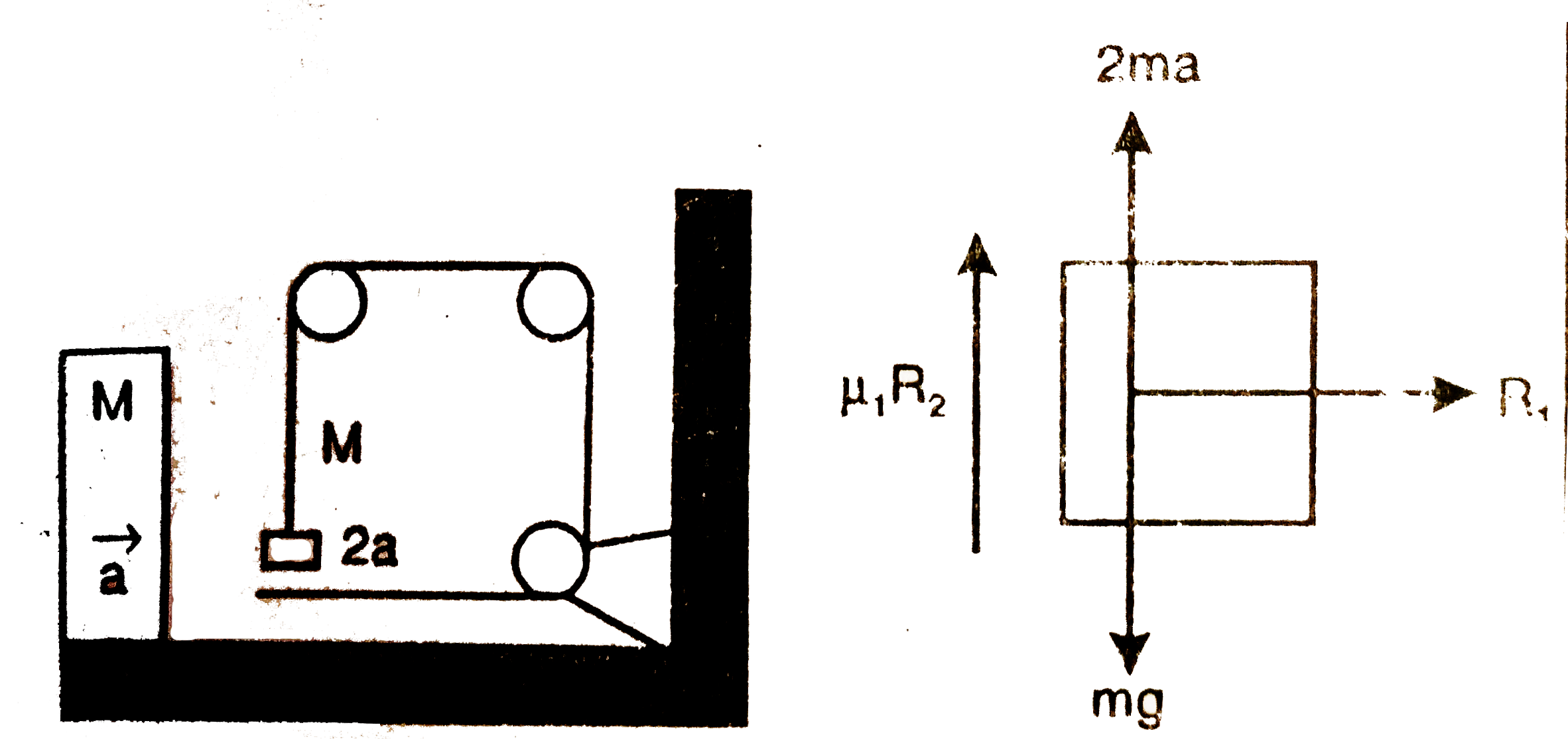
R1−ma=0
→R1=ma..........i
Again, 2ma+T−Mg+μ1R1=0
→T=Mg−(2+μ1)ma.........ii
From free body diagram -2,
T+μ1R1+Mg−R2=0
Putting the value of R1 from i
R2=T+μ1ma+mg
Putting teh value of T from ii,
R2=(Mg−2ma−μ1ma)=μ1ma+Mg+mg
R_2=Mg+mg-2ma.....iii<br>Aga∈omntheeebodydiagram2,<br>T+T-R-Ma-mu_2R_2=0<br>rarr 2T-Ma-ma-mu_2(Mg+mg-2ma)=0<br>Pu∈gthevaluesofR_1 and R_2omiandiii<br>2T=(M+m)a+mu_2(Mg+mg-2ma)....iv<br>Fromequationiiandivwehave<br>2T=2mg-2(2+mu_1)ma<br>=(M+m)a+mu_2(Mg+mg-2ma)<br>rarr 2mg-mu_2(M+m)g<br>=a[M+m-2mu_2m+4m+2mu_1m]<br>rarr a=([2m-m_2(M+m)]g)/(M+m[5+2(mu_1-mu_2)])
Topper's Solved these Questions
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Objective -2
5 Videos
- FLUID MECHANICSBOOK - HC VERMA ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - All Questions
90 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
Similar Questions
The block of mass M and are arrenged as the situation in fig is shown.The coefficient of friction between two block is μand that between the bigger block and the ground is μ find the acceleration of the block
View SolutionThe block of mass M and are arrenged as the situation in fig is shown.The coefficient of friction between two block is μand that between the bigger block and the ground is μ find the acceleration of the block .
View Solution
Knowledge Check
A block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?
 A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)g
A(M+m)(μ2−μ1)gB(M−m)(μ2−μ1)gC(M−m)(μ2+μ1)gD(M+m)(μ2+μ1)gA block of mass m2 is placed on a horizontal table and another block of mass m1 is placed on top is it. An increasing horizontal force F=αt is exerted on the upper block but the lowwer block never moves as a result. If the coefficient of friction between the blocks is μ1 coefficient of friction between the blocks is μ1 and that between the lower block and the table is μ2, then what is the maximum possible value of μ1/μ2 ?
Am2m1B1+m2m1Cm1m2D1+m1m2
A small block of mass m is projected on a larger block of mass 10m and length l with a velocity v as shown in the figure. The coefficient of friction between the two block is μ2 while that between the lower block and the ground is μ1. Given that μ2>11μ1.
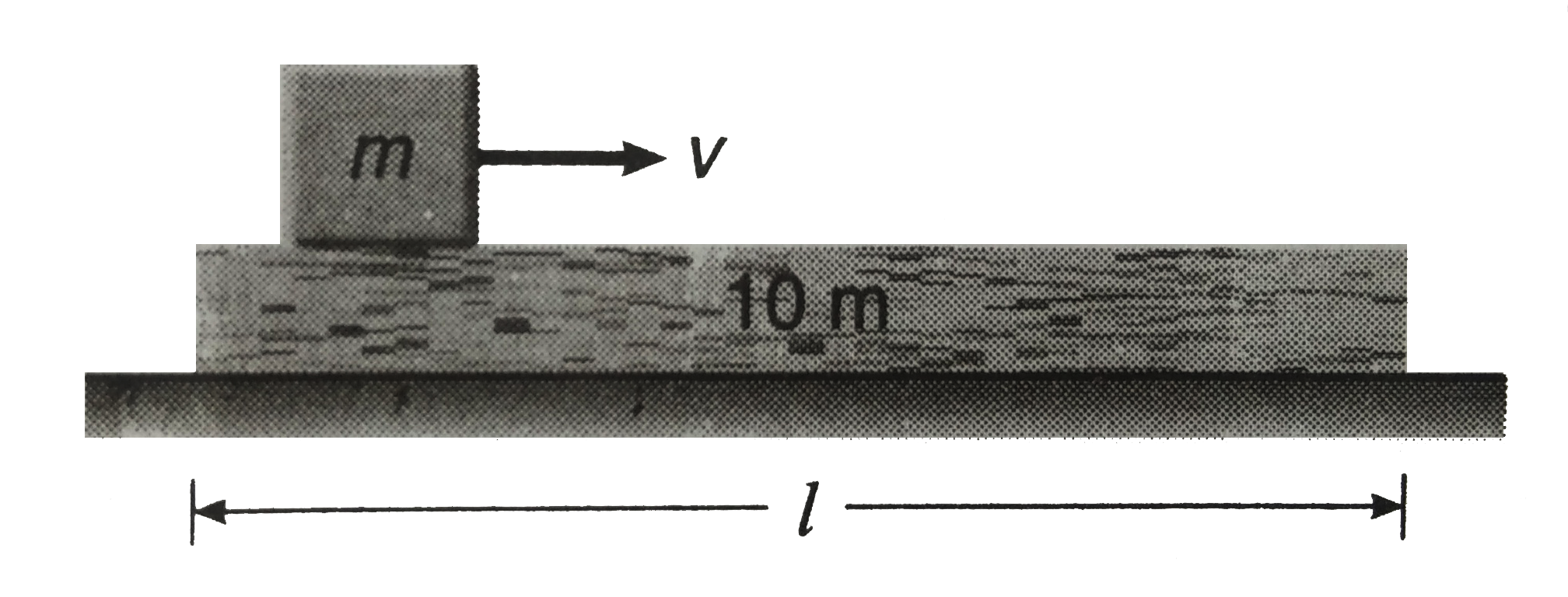
(a) Find the minimum value of v, such that the mass m falls off the block of mass 10m .
(b) If v has minimum value, find the time taken by block m to do so.
View SolutionA small block of mass m is projected on a larger block of mass 10m and length l with a velocity v as shown in the figure. The coefficient of friction between the two block is μ2 while that between the lower block and the ground is μ1. Given that μ2>11μ1.

(a) Find the minimum value of v, such that the mass m falls off the block of mass 10m .
(b) If v has minimum value, find the time taken by block m to do so.
View SolutionThe coefficient of friction between the block and the inclined plane is μ.
View SolutionConsider the situation as shown in the figgure. The coefficent of friction between the blocks is μ. Find the acceleration of each block.
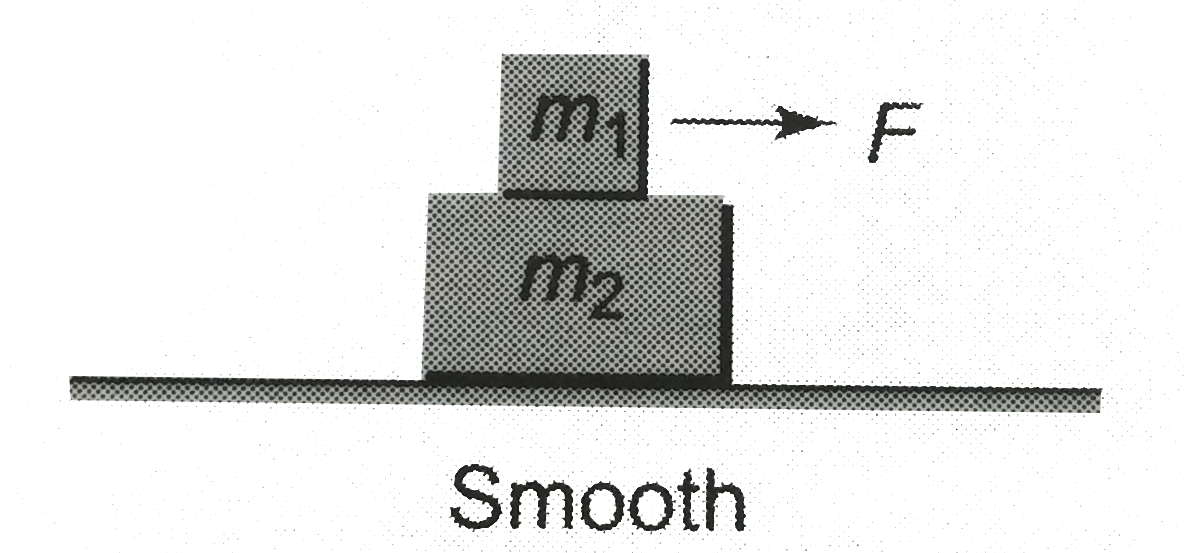
View SolutionA block of massm is placed on another block of mass M which itself is lying on a horizontal surface .The coefficient of friction between two blocks is μ1and that between the block of mass M and horizontal surfece is μ2 What maximum horizontal force can be applied to the lower block move without separation?

View SolutionTwo blocks of masses'm' and 'M' are arranged as shown in the figure. The coefficient of friction between the two blocks is ' μ ', where as between the lower block and the horizontal surface is zero. Find the force 'F' to be applied on the upper block, for the system to be under equilibrium ?

View Solution
