Inside a uniform spherical shell
the gravitational potential si zero
the gravitational field is zero
the gravitational potential is same everywhere
the gravitational field is same everywhere
The correct Answer is:B, C, D
To solve the question regarding the gravitational properties inside a uniform spherical shell, we will analyze the gravitational potential and gravitational field within the shell step by step.
1. Understanding the Concept of Gravitational Field and Potential:
- The gravitational field (E) at a point is defined as the force experienced by a unit mass placed at that point.
- The gravitational potential (V) at a point is defined as the work done in bringing a unit mass from infinity to that point.
2. Gravitational Field Inside a Uniform Spherical Shell:
- According to the shell theorem, the gravitational field inside a uniform spherical shell is zero. This means that if you are inside the shell, you will not experience any gravitational force due to the mass of the shell surrounding you.
3. Gravitational Potential Inside a Uniform Spherical Shell:
- The gravitational potential inside the shell is constant and is equal to the potential at the surface of the shell. However, it is not zero. The potential is the same at all points inside the shell.
4. Analyzing the Options:
- Option A: "The gravitational potential is zero." - This is incorrect because the gravitational potential inside the shell is not zero; it is constant and equal to the potential at the surface.
- Option B: "The gravitational field is zero." - This is correct, as established by the shell theorem.
- Option C: "The gravitational potential is the same everywhere." - This is also correct, as the potential is constant throughout the interior of the shell.
- Option D: "The gravitational field is the same everywhere." - This is misleading because while the gravitational field is zero everywhere inside the shell, it does not imply that it is "the same" in a non-zero sense; however, it is technically correct in the context of being zero everywhere.
5. Conclusion:
- The correct statements are B, C, and D. Therefore, the answer to the question is that options B, C, and D are true.
Final Answer:
The correct options are B, C, and D.
- The gravitational field (E) at a point is defined as the force experienced by a unit mass placed at that point.
- The gravitational potential (V) at a point is defined as the work done in bringing a unit mass from infinity to that point.
- According to the shell theorem, the gravitational field inside a uniform spherical shell is zero. This means that if you are inside the shell, you will not experience any gravitational force due to the mass of the shell surrounding you.
- The gravitational potential inside the shell is constant and is equal to the potential at the surface of the shell. However, it is not zero. The potential is the same at all points inside the shell.
- Option A: "The gravitational potential is zero." - This is incorrect because the gravitational potential inside the shell is not zero; it is constant and equal to the potential at the surface.
- Option B: "The gravitational field is zero." - This is correct, as established by the shell theorem.
- Option C: "The gravitational potential is the same everywhere." - This is also correct, as the potential is constant throughout the interior of the shell.
- Option D: "The gravitational field is the same everywhere." - This is misleading because while the gravitational field is zero everywhere inside the shell, it does not imply that it is "the same" in a non-zero sense; however, it is technically correct in the context of being zero everywhere.
- The correct statements are B, C, and D. Therefore, the answer to the question is that options B, C, and D are true.
The correct options are B, C, and D.
Topper's Solved these Questions
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercises
39 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Question for short Answers
18 Videos
- GRAVITATIONBOOK - HC VERMA ENGLISHCHAPTER - GRAVITATIONEXERCISE - Objective -1
16 Videos
- FRICTIONBOOK - HC VERMA ENGLISHCHAPTER - FRICTIONEXERCISE - Questions for short Answer
11 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 2
6 Videos
Similar Questions
A particle placed
1.inside a uniform spherical shell of mass M, but not at the center
2.inside a uniform spherical shell of mass M, at the center
3.outside a uniform spherical shell of mass M, a distance r from the center
4.outside a uniform solid sphere of mass M, a distance 2r from the center
If F1,F2,F3 and F4 are gravitational forces acting on the particle in four cases.
View SolutionInside a uniform shell
View SolutionFor a spherical shell
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the centre.
View SolutionA small area is removed from a uniform spherical shell of mass M and radius R. Then the gravitatioinal field intensity near the hollow portion is
View SolutionA particle of mass M is placed at the centre of a uniform spherical shell of equal mass and radius a. Find the gravitational potential at a point P at a distance a2 from the center.
View SolutionA small section of area ΔA is removed from a uniform spherical shell with surface mass density σ and radius R as shown in the figure . Find the magnitude of gravitational field intensity at point P due to the remaining mass .
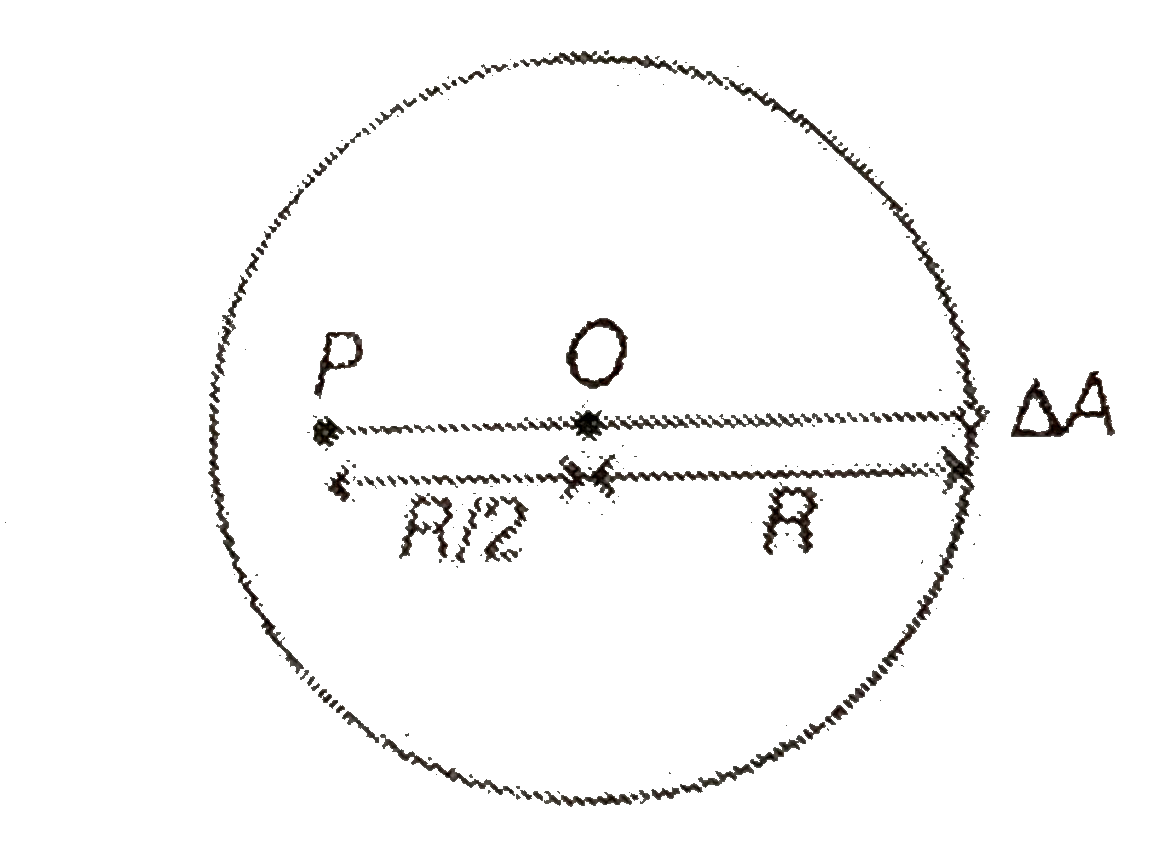
View SolutionA uniform metal sphere of radius a and mass M is surrounded by a thin uniform spherical shell of equal mass and redius 4a. The centre of the shell falls on the surface of the iner sphere
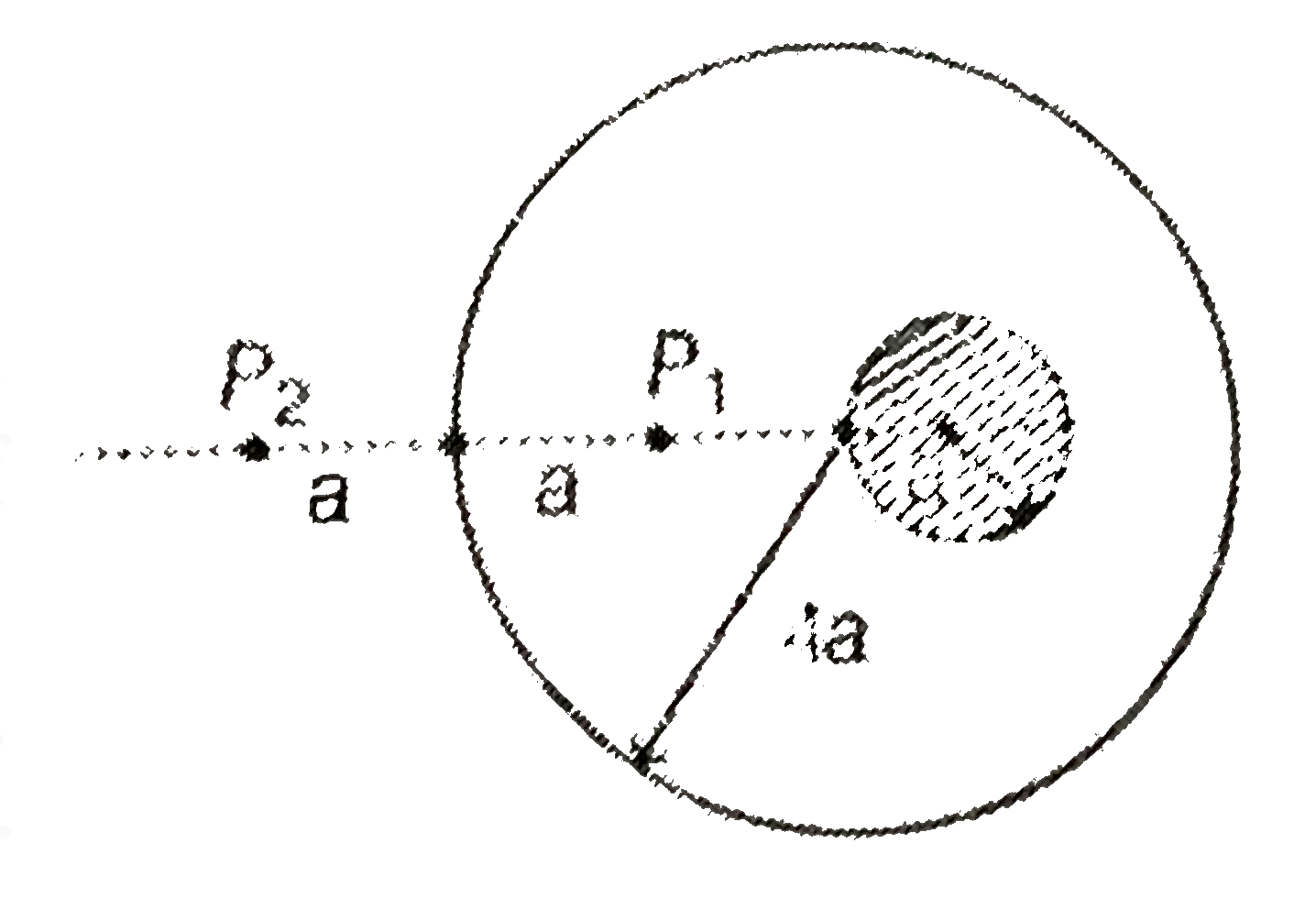
View SolutionConsider a thin uniform spherical layer of mass M and radius R. The potential energy of gravitational interaction of matter forming this shell is :
View Solution
