A bicovex lens has radii of cuvature 20 cm each. If the refractive index of the material of the lens is 1.5, what is its focal length?
To find the focal length of a biconvex lens with given radii of curvature and refractive index, we can use the lens maker's formula. Here’s a step-by-step solution:
Step 1: Identify the parameters
- The lens is biconvex, which means it has two convex surfaces.
- The radius of curvature (R) for both surfaces is given as 20 cm.
- The refractive index (μ) of the lens material is 1.5.
Step 2: Assign the radii of curvature
- For a biconvex lens:
- The first radius of curvature (R1) is positive: R1=+20cm
- The second radius of curvature (R2) is negative: R2=−20cm
Step 3: Write the lens maker's formula
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
Where:
- f is the focal length,
- μ is the refractive index,
- R1 and R2 are the radii of curvature.
Step 4: Substitute the values into the formula
Substituting the known values into the lens maker's formula:
1f=(1.5−1)(120−1−20)
Step 5: Simplify the equation
Calculating the terms:
1f=0.5(120+120)
1f=0.5(220)
1f=0.5×110
1f=0.510
1f=5100
Step 6: Calculate the focal length
Taking the reciprocal to find f:
f=1005=20cm
Conclusion
The focal length of the biconvex lens is 20cm.
---
- The lens is biconvex, which means it has two convex surfaces.
- The radius of curvature (R) for both surfaces is given as 20 cm.
- The refractive index (μ) of the lens material is 1.5.
- For a biconvex lens:
- The first radius of curvature (R1) is positive: R1=+20cm
- The second radius of curvature (R2) is negative: R2=−20cm
The lens maker's formula is given by:
1f=(μ−1)(1R1−1R2)
Where:
- f is the focal length,
- μ is the refractive index,
- R1 and R2 are the radii of curvature.
Substituting the known values into the lens maker's formula:
1f=(1.5−1)(120−1−20)
Calculating the terms:
1f=0.5(120+120)
1f=0.5(220)
1f=0.5×110
1f=0.510
1f=5100
Taking the reciprocal to find f:
f=1005=20cm
The focal length of the biconvex lens is 20cm.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercises
78 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question
7 Videos
- LIGHT WAVESBOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer
11 Videos
Similar Questions
A biconvex lens has radii of curvature 20 cm and 40cm. The refractive index of the material of the lens is 1.5. An object is placed 40cm in front of the lens. Calculate the psition of the image.
View SolutionA converging bundle of rays is intercepted by a biconcave lens. The radii of curvature of both surfaces are 20cm and the refractive index of the material of the lens is 1.5. If the rays originially converged to a point 10cm in front of the lens, where will they now converge after passing through the lens?
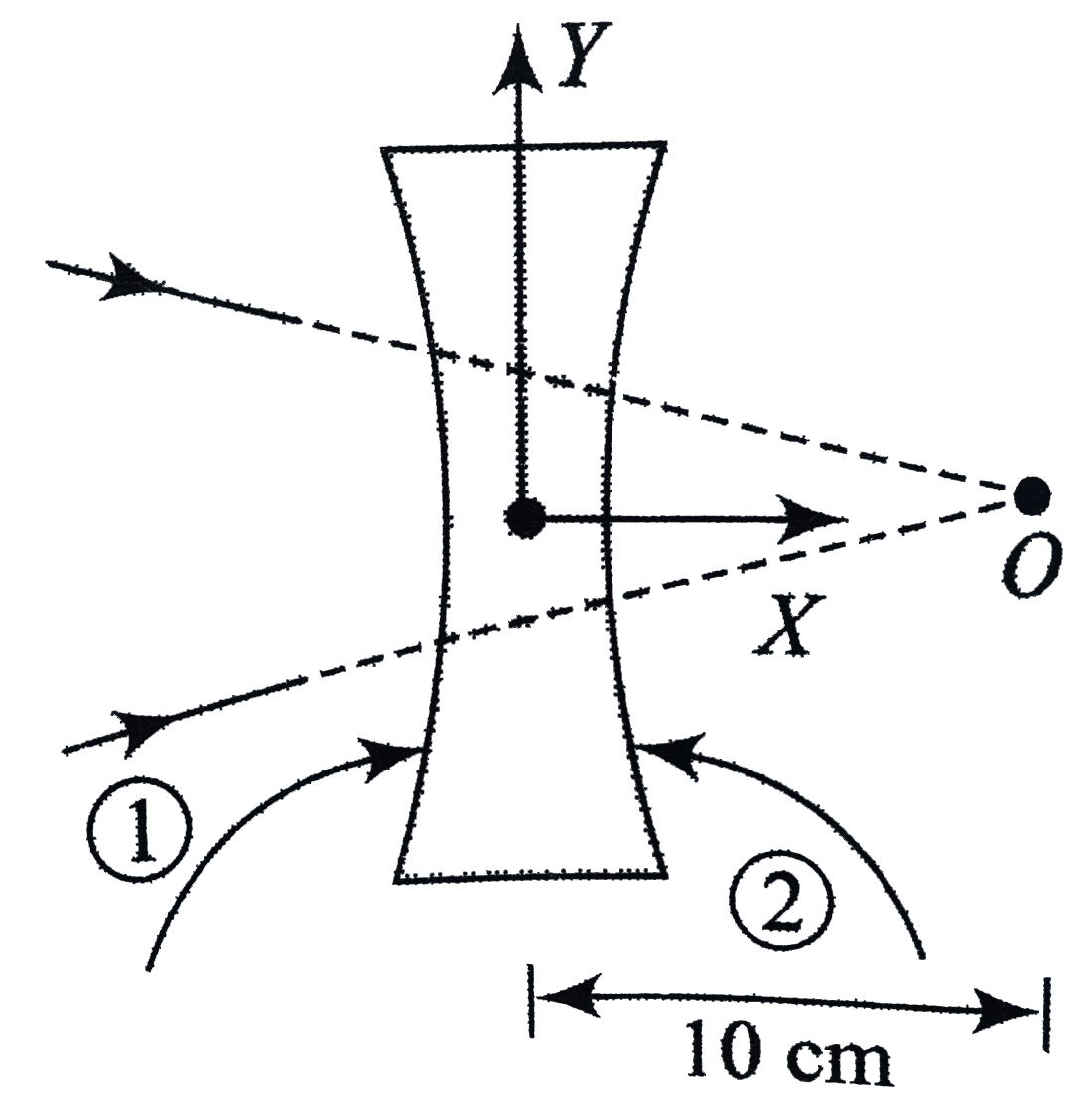
View Solution
Knowledge Check
The radius of curvature of the curved surface of a plano-convex lens is 20cm. If the refractive index of the material of the lens be 1.5, it will
Aact as a convex lens only for the objects that lie on its curved side.Bact as a concave lens for the objects that lie on its curved side.Cact as a convex lens irrespective of the side on which the object lies.Dact as a concave lens irrespective of side on which the object lies.
The radii of curvature of the two surfaces of a lens are 20 cm and 30 cm and the refractive index of the material of the lens is 1.5. If the lens is concave -convex, then the focal length of the lens is
View SolutionThe radii of curvature of the two surfaces of a lens are 20 cm and 30 cm and the refractive index of the material of the lens is 1.5. If the lens is concave -convex, then the focal length of the lens is
View SolutionThe radius of curvature of the convex face of a plano convex lens is 12cm and the refractive index of the material of the lends 1.5. Then, the focal length of the lens is
View SolutionConsider an equiconvex lens of radius of curvature R and focal length f. If f>R , the refractive index μ of the material of the lens
View SolutionThe effective focal length of the lens combination shown in figure is -60 cm. The radius of curvature of the curved surfaces of the plano-convex lenses are 12 cm each and refractive index of the material of the lens is 1.5. The refractive index of the liquid is
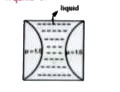
View SolutionA glass slab of thickness 2cm and refractive index 2 is placed in contact with a biconvex lens of focal length 20cm. The refractive index of the material of the lens 1.5 . The far side of the lens is silvered. Where should an object be placed in front of the slab so that it images on to itself?
View SolutionFind the radius of curvature of the convex surface of a plan-convex lens, whose focal length is 0.3 m and the refractive index of the material of the lens is 1.5.
View Solution
