One end of a horizontal cylindrical glass rod (μ=1.5) of radius 5.0 cm is rounded in the shape of a hemisphere. An object 0.5 mm high is placed perpendicular to the axis of the rod at a difference of 20.0 cm from the rounded edge. Locate the image of the object and find its height.
To solve the problem, we will follow these steps:
Step 1: Understand the Problem
We have a cylindrical glass rod with a hemispherical end. An object is placed at a distance from the rounded edge, and we need to find the image location and height.
Step 2: Identify Given Data
- Refractive index of glass, μ2=1.5
- Refractive index of air, μ1=1.0
- Radius of curvature, R=5cm
- Object height, ho=0.5mm=0.05cm (converted to cm)
- Object distance from the rounded edge, u=−20cm (negative since it is on the same side as the object)
Step 3: Use the Refraction Formula
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
Substituting the known values:
1.5v−1.0−20=1.5−1.05
Step 4: Simplify the Equation
This simplifies to:
1.5v+120=0.55
1.5v+120=0.1
Step 5: Solve for v
Rearranging gives:
1.5v=0.1−120
Finding a common denominator (20):
0.1=220
Thus:
1.5v=220−120=120
Cross-multiplying gives:
1.5⋅20=v⟹v=30cm
Step 6: Calculate Magnification
The magnification m is given by:
m=−μ1vμ2u
Substituting the values:
m=−1⋅301.5⋅(−20)=−30−30=1
Step 7: Calculate Image Height
The image height hi can be calculated using:
hi=m⋅ho
Substituting the values:
hi=1⋅0.05cm=0.05cm=0.5mm
Final Results
- The image is located at 30cm from the rounded edge.
- The height of the image is 0.5mm.
---
We have a cylindrical glass rod with a hemispherical end. An object is placed at a distance from the rounded edge, and we need to find the image location and height.
- Refractive index of glass, μ2=1.5
- Refractive index of air, μ1=1.0
- Radius of curvature, R=5cm
- Object height, ho=0.5mm=0.05cm (converted to cm)
- Object distance from the rounded edge, u=−20cm (negative since it is on the same side as the object)
The formula for refraction at a spherical surface is given by:
μ2v−μ1u=μ2−μ1R
Substituting the known values:
1.5v−1.0−20=1.5−1.05
This simplifies to:
1.5v+120=0.55
1.5v+120=0.1
Rearranging gives:
1.5v=0.1−120
Finding a common denominator (20):
0.1=220
Thus:
1.5v=220−120=120
Cross-multiplying gives:
1.5⋅20=v⟹v=30cm
The magnification m is given by:
m=−μ1vμ2u
Substituting the values:
m=−1⋅301.5⋅(−20)=−30−30=1
The image height hi can be calculated using:
hi=m⋅ho
Substituting the values:
hi=1⋅0.05cm=0.05cm=0.5mm
- The image is located at 30cm from the rounded edge.
- The height of the image is 0.5mm.
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -115videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Objective -27videos
GEOMETRICAL OPTICS
- BOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Short Question7videos
GAUSS LAW
- BOOK - HC VERMA ENGLISHCHAPTER - LIGHT WAVESEXERCISE - Question for short Answer11videos
LIGHT WAVES
Similar Questions
One end of a cylindrical glass rod (mu = 1.5) of radius 1.0 cm is rounded in the shape of a hemisphere. The rod is immersed in water (mu= 4/3) and an object is placed in the water along the axis of the rod at a distance of 8.0 cm from the rounded edge. Locate the image of the object.
View SolutionA 1 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. Find its distance from the mirror if the image formed is 0.6 cm in size.
View SolutionAn object of length 2.0 cm is placed perpendicular to the principal axis of a convex lens of focal length 12 cm. Find the size of the image of the object is at a distance of 8.0 cm from the lens.
View SolutionAn object is placed on the principal axis of concave mirror of focal length 10 cm at a distance of 8.0 cm from the pole. Find the position and the nature of the image.
View SolutionA cylinderical glass rod of radius 0.1m and refractive index √3 lies on a horizontal plane mirror. A horizontal ray of light moving perpendicular to the axis of the rod is incident on it. At what height from the mirror should the ray be incident so that it leaves the rod at a height of 0.1m above the plane mirror? At what centre to centre distance a second similar rod, parallel to the first, be placed on the mirror, such that the emergent ray from the second rod is in line with the incident ray on the first rod?
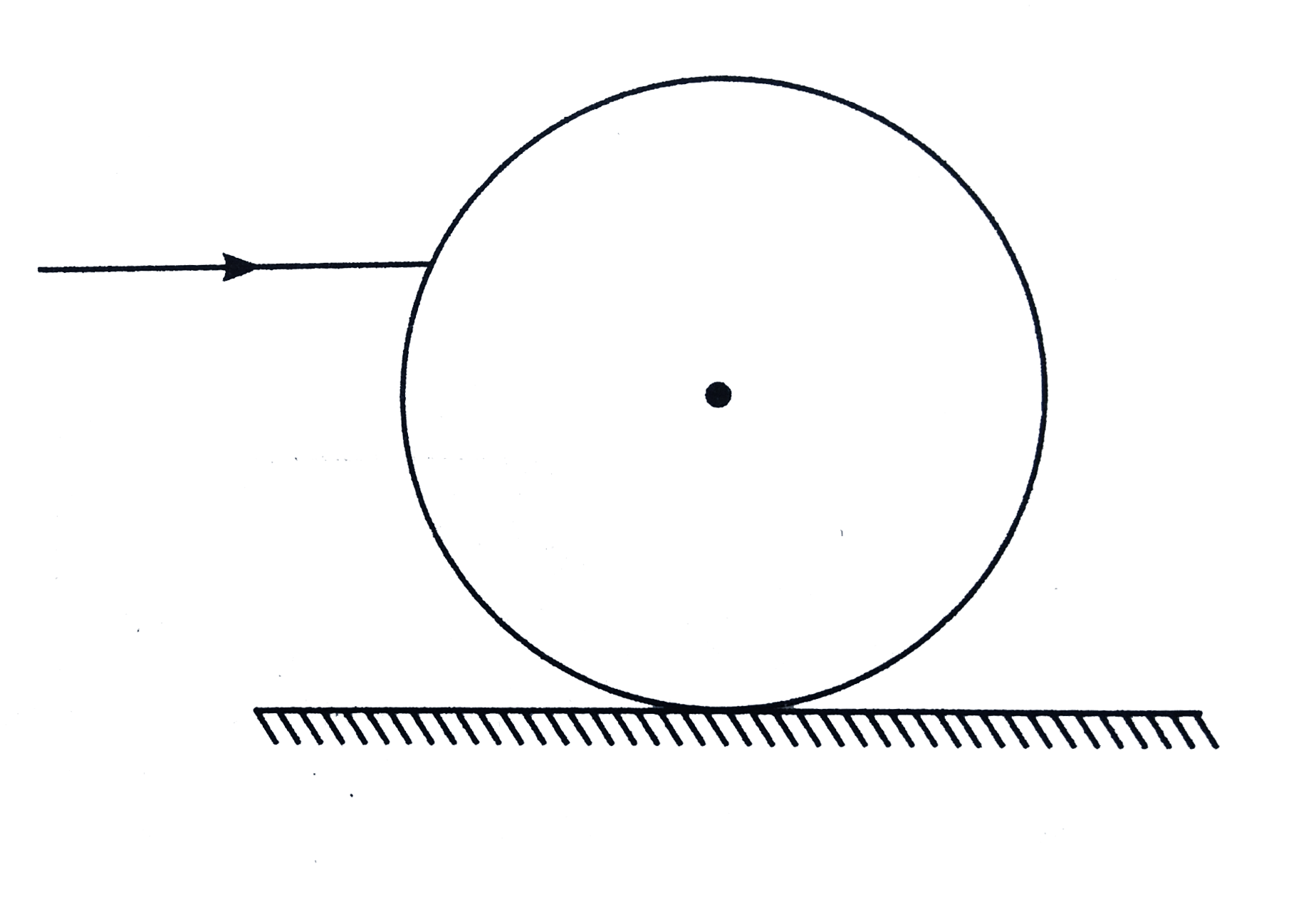 View Solution
View Solution
A plane wave front of light is incident on a plane mirror. Intensity is maximum at P whenView SolutionA 4 cm object is placed perpendicular to the principal axis of a convex mirror of focal length 7.5 cm. The distance of the image from the mirror if it is 0.6 cm in size is 51/x where x is
View SolutionAn object 2.5 cm high is placed at a distance of 10 cm from a concave mirror of radius of curvature 30 cm The size of the image is
View Solution20 circular plates each of radius 5 cm and thickenss 10 mm are placed one above another. What is the shape of solid made and find its height?
View SolutionOne end of a long glass rod (μ=1.5) is formed into a convex surface of radius 6.0cm. An object is positioned in air along the axis of the rod. Find the image positions corresponding to object distances of (a) 20.0cm, (b) 10.0cm, (c) 3.0cm from the end of the rod.
View Solution

