Three rods of lengths 20cm each and area of cross section 1cm2 are joined to from a triangle ABC. The conductivities of the rods are kAB=50Js−1m−1C−1 , kBC=200Js−1m−1C−1 , and kAC=400Js−1m−1C−1 . The junction A,B and C are maintained at 40∘C , 80∘C and 80∘C respectively. Find the rate of heat flowing through the rods AB, AC and BC .
The correct Answer is:A
To solve the problem, we need to calculate the rate of heat transfer through each of the rods AB, AC, and BC using Fourier's law of heat conduction. The formula for the rate of heat transfer (Q/t) through a rod is given by:
Qt=k⋅A⋅ΔTL
Where:
- k = thermal conductivity of the material (in Js−1m−1C−1)
- A = cross-sectional area (in m2)
- ΔT = temperature difference across the rod (in °C)
- L = length of the rod (in m)
Step 1: Calculate the rate of heat flow through rod AB
1. Identify the parameters for rod AB:
- kAB=50Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TB−TA=80°C−40°C=40°C
- L=20cm=0.2m
2. Substitute the values into the formula:
QtAB=50⋅(1×10−4)⋅400.2
3. Calculate:
QtAB=50⋅(1×10−4)⋅200=1W
Step 2: Calculate the rate of heat flow through rod AC
1. Identify the parameters for rod AC:
- kAC=400Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TC−TA=80°C−40°C=40°C
- L=20cm=0.2m
2. Substitute the values into the formula:
QtAC=400⋅(1×10−4)⋅400.2
3. Calculate:
QtAC=400⋅(1×10−4)⋅200=8W
Step 3: Calculate the rate of heat flow through rod BC
1. Identify the parameters for rod BC:
- kBC=200Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TC−TB=80°C−80°C=0°C
- L=20cm=0.2m
2. Substitute the values into the formula:
QtBC=200⋅(1×10−4)⋅00.2
3. Calculate:
QtBC=200⋅(1×10−4)⋅0=0W
Final Results:
- Rate of heat flow through rod AB: 1W
- Rate of heat flow through rod AC: 8W
- Rate of heat flow through rod BC: 0W
- k = thermal conductivity of the material (in Js−1m−1C−1)
- A = cross-sectional area (in m2)
- ΔT = temperature difference across the rod (in °C)
- L = length of the rod (in m)
- kAB=50Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TB−TA=80°C−40°C=40°C
- L=20cm=0.2m
QtAB=50⋅(1×10−4)⋅400.2
QtAB=50⋅(1×10−4)⋅200=1W
- kAC=400Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TC−TA=80°C−40°C=40°C
- L=20cm=0.2m
QtAC=400⋅(1×10−4)⋅400.2
QtAC=400⋅(1×10−4)⋅200=8W
- kBC=200Js−1m−1C−1
- A=1cm2=1×10−4m2
- ΔT=TC−TB=80°C−80°C=0°C
- L=20cm=0.2m
QtBC=200⋅(1×10−4)⋅00.2
QtBC=200⋅(1×10−4)⋅0=0W
- Rate of heat flow through rod AB: 1W
- Rate of heat flow through rod AC: 8W
- Rate of heat flow through rod BC: 0W
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER11videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II6videos
HEAT TRANSFER
- BOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 26videos
HEAT AND TEMPERATURE
- BOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer4videos
INTRODUCTION TO PHYSICS
Similar Questions
Two parallel plates A and B are joined together to form a compound plate . The thicknesses of the plate are 4.0cm and 2.5cm respectively and the area of cross section is 100cm2 for each plate. The thermal conductivities are KA=200Wm−1C−1 for plate A and KB=400Wm−1C−1 for the plate B. The outer surface of the plate A is maintained at100∘C and the outer surface of the plate B is maintained at 0∘C. Find (a) the rate of heat flow through any cross section, (b) the temperature at the interface and (c) the equivalent thermal conductivity of the compound plate.
View SolutionFind the thermal resistance of an aluminium rod of length 20cm and area of cross section 1cm2 . The heat current is along the length of the rod. Thermal conductivity of aluminium =200Wm−1K−1 .
View SolutionA steel frame (K=45Wm−1C−1) of total length 60cm and cross sectional area 0.2cm2 , Forms three side of a square. The free ends are maintained at 20∘C and 40∘C . Find the rate of heat flow through a cross section of the frame.
View Solution
A circular ring (centre O) of radius a, and of uniform cross section is made up of three different metallic rods AB, BC and CA (joined together at the points A, B and C in pairs) of thermal conductivityies α1,α2 and α3 respectively (see diagram). The junction A, B and C are maintained at the temperatures 100∘C,50∘C and 0∘C, respectively. All the rods are of equal lengths and cross sections. Under steady state conditions, assume that no heat is lost from the sides of the rods. Let Q1,Q2 and Q3 be the rates of transmission of heat along the three rods AB, BC and CA. ThenView Solution-
A circular ring (centre O) of radius a, and of uniform cross section is made up of three different metallic rods AB, BC and CA (joined together at the points A, B and C in pairs) of thermal conductivityies α1,α2 and α3 respectively (see diagram). The junction A, B and C are maintained at the temperatures 100∘C,50∘C and 0∘C, respectively. All the rods are of equal lengths and cross sections. Under steady state conditions, assume that no heat is lost from the sides of the rods. Let Q1,Q2 and Q3 be the rates of transmission of heat along the three rods AB, BC and CA. ThenView Solution The ends of copper rod of length 1m and area of cross section 1cm2 are maintained at 0∘C and 100∘C. At the centre of rod, there is a source of heat of 32W. The thermal conductivity of copper is 400W/mK
View SolutionFind the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1) . Thermal conductivity of the material of the rod is K.
View SolutionA rod AB of length 30 cm moves such that its ends always touching the co-ordinate axes. Determine the locus of a point C of the rod if AC : BC = 2 : 1
View SolutionThree conducting rods of same material and cross-section are shown in figure. Temperatures of A,D and C are maintained at 20∘C,90∘Cand0∘C. The ratio of lengths BD and BC if there is no heat flow in AB is
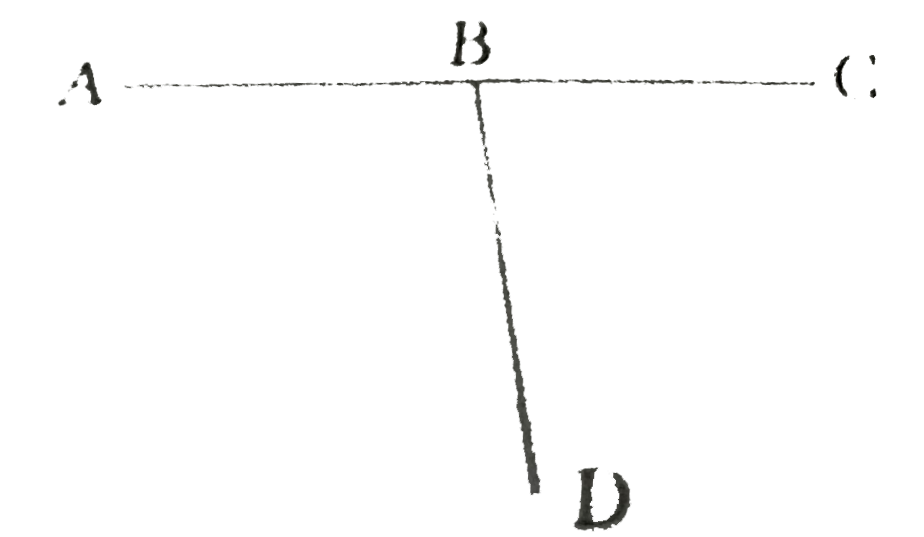View SolutionA rod of negligible heat capacity has length 20cm, area of cross section 1.0cm2 and thermal conductivity 200Wm−1C−1 . The temperature of one end is maintained at 0∘C and that of the other end is slowly and linearly varied from 0∘C to 60∘C in 10 minutes. Assuming no loss of heat through the sides, find the total heat transmitted through the rod in these 10 minutes.
View Solution