A hollow tube has a length l, inner radius R1 and outer radius R2 . The material has a thermal conductivity K. Find the heat flowing through the walls of the tube if (a) the flat ends are maintained at temperature T1 and T2(T2>T1) (b) the inside of the tube is maintained at temperature T1 and the outside is maintained at T2 .
The correct Answer is:A, B
To solve the problem of heat conduction through a hollow tube, we will analyze both scenarios step by step.
Given:
- Length of the tube: l
- Inner radius: R1
- Outer radius: R2
- Thermal conductivity: K
- Temperatures: T1 and T2 (where T2>T1)
Part (a): Flat ends maintained at temperatures T1 and T2
1. Identify the Heat Transfer Equation:
The heat transfer through conduction can be expressed using Fourier's law:
Q=K⋅A⋅(T2−T1)l
where A is the cross-sectional area through which heat is conducted.
2. Calculate the Cross-sectional Area:
The area A for a hollow tube is given by the difference in the areas of the outer and inner circles:
A=πR22−πR21=π(R22−R21)
3. Substitute the Area into the Heat Transfer Equation:
Now, substituting the area into the heat transfer equation:
Q=K⋅π(R22−R21)⋅(T2−T1)l
4. Final Expression for Part (a):
Thus, the heat flowing through the walls of the tube when the flat ends are maintained at temperatures T1 and T2 is:
Q=K⋅π(R22−R21)⋅(T2−T1)l
Part (b): Inside of the tube maintained at temperature T1 and outside at T2
1. Identify the Heat Transfer Equation:
In this case, the heat flows radially from the inner surface to the outer surface. We will use the differential form of Fourier's law:
dQ=−K⋅A⋅dTdr
2. Calculate the Area for Radial Flow:
The area A for a cylindrical shell of radius r and thickness dr is:
A=2πr⋅l
3. Set Up the Differential Equation:
Substituting the area into the differential equation:
dQ=−K⋅(2πr⋅l)⋅dTdr
4. Rearranging the Equation:
Rearranging gives us:
dQdr=−2πKlr⋅(T2−T1)
5. Integrate:
Integrate from R1 to R2 for Q and from T1 to T2 for T:
∫0QdQ=−2πKl∫R2R1(T2−T1)rdr
This results in:
Q⋅ln(R2R1)=−2πKl(T2−T1)
6. Final Expression for Part (b):
Rearranging gives us:
Q=2πKl(T1−T2)ln(R2R1)
Summary of Results:
- Part (a):
Q=K⋅π(R22−R21)⋅(T2−T1)l
- Part (b):
Q=2πKl(T1−T2)ln(R2R1)
- Length of the tube: l
- Inner radius: R1
- Outer radius: R2
- Thermal conductivity: K
- Temperatures: T1 and T2 (where T2>T1)
The heat transfer through conduction can be expressed using Fourier's law:
Q=K⋅A⋅(T2−T1)l
where A is the cross-sectional area through which heat is conducted.
The area A for a hollow tube is given by the difference in the areas of the outer and inner circles:
A=πR22−πR21=π(R22−R21)
Now, substituting the area into the heat transfer equation:
Q=K⋅π(R22−R21)⋅(T2−T1)l
Thus, the heat flowing through the walls of the tube when the flat ends are maintained at temperatures T1 and T2 is:
Q=K⋅π(R22−R21)⋅(T2−T1)l
In this case, the heat flows radially from the inner surface to the outer surface. We will use the differential form of Fourier's law:
dQ=−K⋅A⋅dTdr
The area A for a cylindrical shell of radius r and thickness dr is:
A=2πr⋅l
Substituting the area into the differential equation:
dQ=−K⋅(2πr⋅l)⋅dTdr
Rearranging gives us:
dQdr=−2πKlr⋅(T2−T1)
Integrate from R1 to R2 for Q and from T1 to T2 for T:
∫0QdQ=−2πKl∫R2R1(T2−T1)rdr
Q⋅ln(R2R1)=−2πKl(T2−T1)
Rearranging gives us:
Q=2πKl(T1−T2)ln(R2R1)
- Part (a):
Q=K⋅π(R22−R21)⋅(T2−T1)l
- Part (b):
Q=2πKl(T1−T2)ln(R2R1)
Topper's Solved these Questions
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - QUESTIONS FOR SHORT ANSWER
11 Videos
- HEAT TRANSFERBOOK - HC VERMA ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - OBJECTIVE II
6 Videos
- HEAT AND TEMPERATUREBOOK - HC VERMA ENGLISHCHAPTER - HEAT AND TEMPERATUREEXERCISE - Objective 2
6 Videos
- INTRODUCTION TO PHYSICSBOOK - HC VERMA ENGLISHCHAPTER - INTRODUCTION TO PHYSICSEXERCISE - Question for short Answer
4 Videos
Similar Questions
A thin capillary of inner radius r1 and outer radius r2 (The inner tube is solid) is dipped in water. To how much height will the water raise in the tube ? (Assume contact angle θ→0)
View SolutionA rod of length l and cross-section area A has a variable thermal conductivity given by K = α T, where α is a positive constant and T is temperature in kelvin. Two ends of the rod are maintained at temperature T1 and T2 (T1>T2). Heat current flowing through the rod will be
View SolutionTwo thin conectric shells made of copper with radius r1 and r2(r2>r1) have a material of thermal conductivity K filled between them. The inner and outer spheres are maintained at temperature TH and TC respectively by keeping a heater of power P at the centre of the two sphers. Find the value of P.
View SolutionA slab of cunductivity k is in the shape of trapezoid as shown I figure. If the two end faces be maintained at temperature T1=50∘CandT2=25∘C, then determine the thermal current through the slab by ignoring any heat loss through the lateral surfaces.
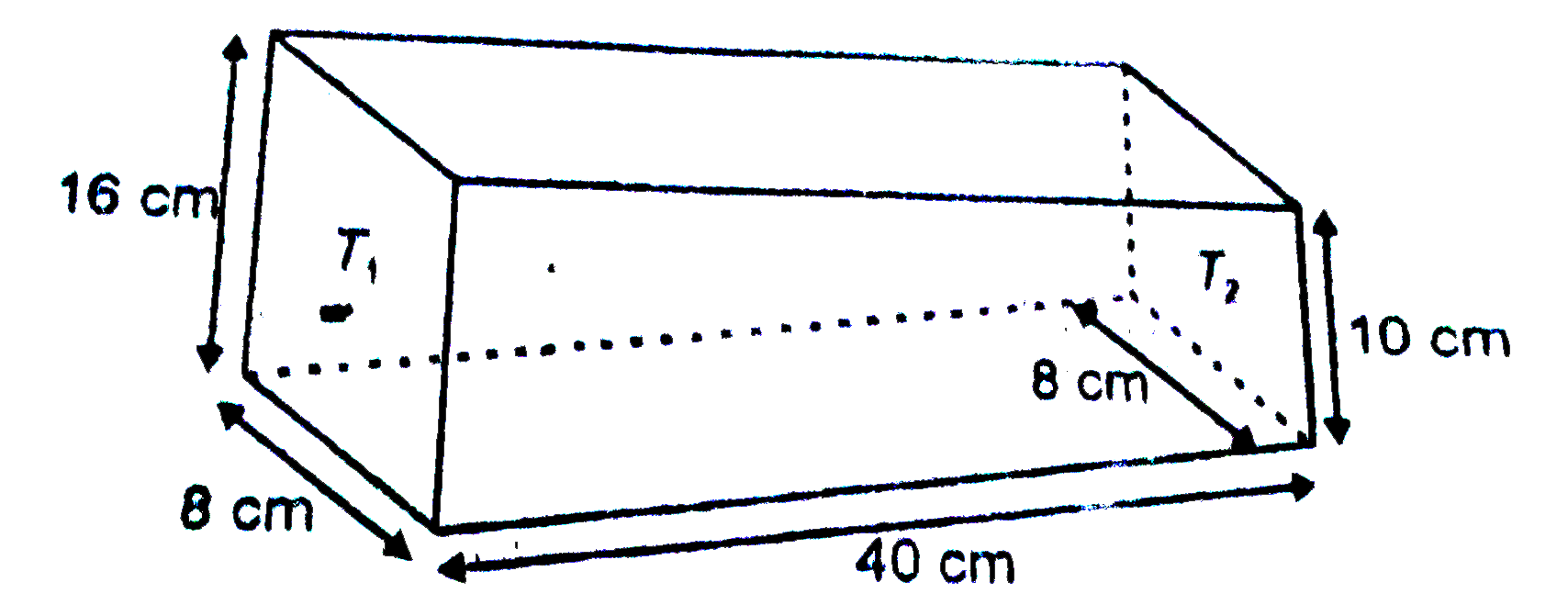 .
.
View SolutionA rod of length l and cross sectional area A has a variable conductivity given by K=αT, where α is a positive constant T is temperatures in Kelvin. Two ends of the rod are maintained at temperatures T1 and T2(T1>T2). Heat current flowing through the rod will be
View SolutionThree rods of same cross-section but different length and conductivity are joined in series . If the temperature of the two extreme ends are T1andT2(T1>T2) find the rate of heat transfer H.
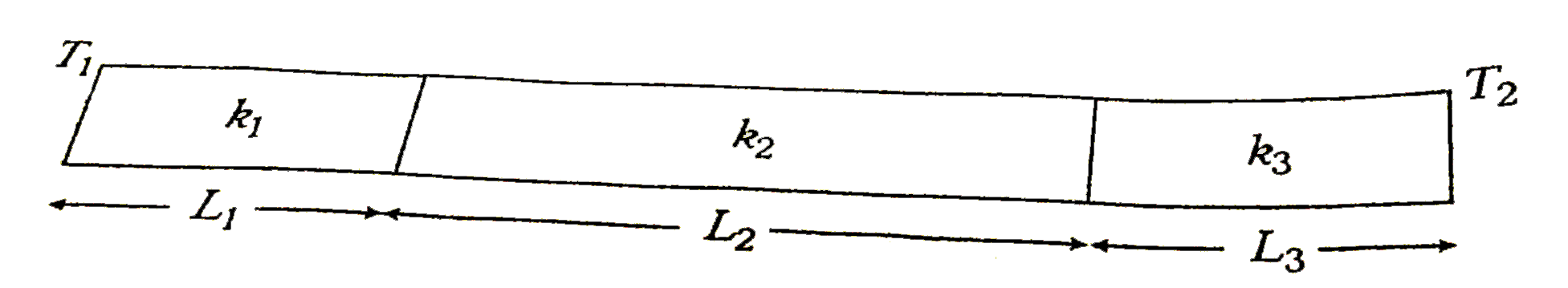
View SolutionA solid at temperature T1 is kept in an evacuated chamber at temperature T2>T1 . The rate of increase of temperature of the body is propertional to
View SolutionA cylinder of radius r made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius r and outer radius 2r made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. Show that the effective thermal conductivity of the system is (K1+3K2)/4.
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effective thermal conductivity of the system is
(a) K1+K2 (b) K1K2/(K1+K2)
(c )(K1+3K2)/4
(d) (3K1+K2)/4.
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effective thermal conductivity of the system is
View Solution
