Electric charge are distributed in a small volume. The flux of the electric field through a spherical surface of radius 10cm surrounding the total charge is 25 V m. The flux over a concentric sphere of radius 20 cm will be
25 V m
50 V m
100 V m
200 V m.
The correct Answer is:A
To solve the problem, we will use Gauss's Law, which states that the electric flux (Φ) through a closed surface is proportional to the charge (Q) enclosed by that surface. The formula for Gauss's Law is given by:
Φ=Qinϵ0
where:
- Φ is the electric flux,
- Qin is the total charge enclosed within the surface,
- ϵ0 is the permittivity of free space.
1. Identify the Given Information:
- The flux through a spherical surface of radius 10 cm is given as Φ1=25V m.
- We need to find the flux through a concentric spherical surface of radius 20 cm, denoted as Φ2.
2. Understand Gauss's Law:
- According to Gauss's Law, the electric flux through a closed surface depends only on the charge enclosed within that surface, not on the size or shape of the surface.
3. Apply Gauss's Law:
- Since the charge is enclosed within both the 10 cm and 20 cm spherical surfaces, the total charge Qin remains the same for both surfaces.
- Therefore, the flux through the first surface (10 cm) is equal to the flux through the second surface (20 cm):
Φ2=Φ1
4. Conclude the Calculation:
- Since Φ1=25V m, we have:
Φ2=25V m
Final Answer:
The flux over the concentric sphere of radius 20 cm will also be 25V m.
- Φ is the electric flux,
- Qin is the total charge enclosed within the surface,
- ϵ0 is the permittivity of free space.
- The flux through a spherical surface of radius 10 cm is given as Φ1=25V m.
- We need to find the flux through a concentric spherical surface of radius 20 cm, denoted as Φ2.
- According to Gauss's Law, the electric flux through a closed surface depends only on the charge enclosed within that surface, not on the size or shape of the surface.
- Since the charge is enclosed within both the 10 cm and 20 cm spherical surfaces, the total charge Qin remains the same for both surfaces.
- Therefore, the flux through the first surface (10 cm) is equal to the flux through the second surface (20 cm):
Φ2=Φ1
- Since Φ1=25V m, we have:
Φ2=25V m
The flux over the concentric sphere of radius 20 cm will also be 25V m.
Topper's Solved these Questions
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Objective 2
8 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Exercises
24 Videos
- GAUSS LAWBOOK - HC VERMA ENGLISHCHAPTER - GAUSS LAWEXERCISE - Work Out Exaples
8 Videos
- ELECTROMAGNETIC WAVESBOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise
8 Videos
- GEOMETRICAL OPTICSBOOK - HC VERMA ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Question For short Answer
18 Videos
Similar Questions
The electric charges are distributed in a small volume. The flux of the electric field through a spherica surface of radius 10 cm surrounding the total charge is 20V−m. The flux over a concentric sphere of radius 20 cm will be
View SolutionThe flux of electric field through the circular region of radius r as shown in the figure is
_PHY_E05_291_Q01.png)
View SolutionFind the flux due to the electric field through the curved surface (R is radius of curvature)

View SolutionA charge Q is placed at the centre of a cube. Find the flux of the electric field through the six surfaced of the cube.
View SolutionA capacitor of capacitance C is charged to a potential V. The flux of the electric field through a closed surface enclosing the capacitor is
View SolutionFigure shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is
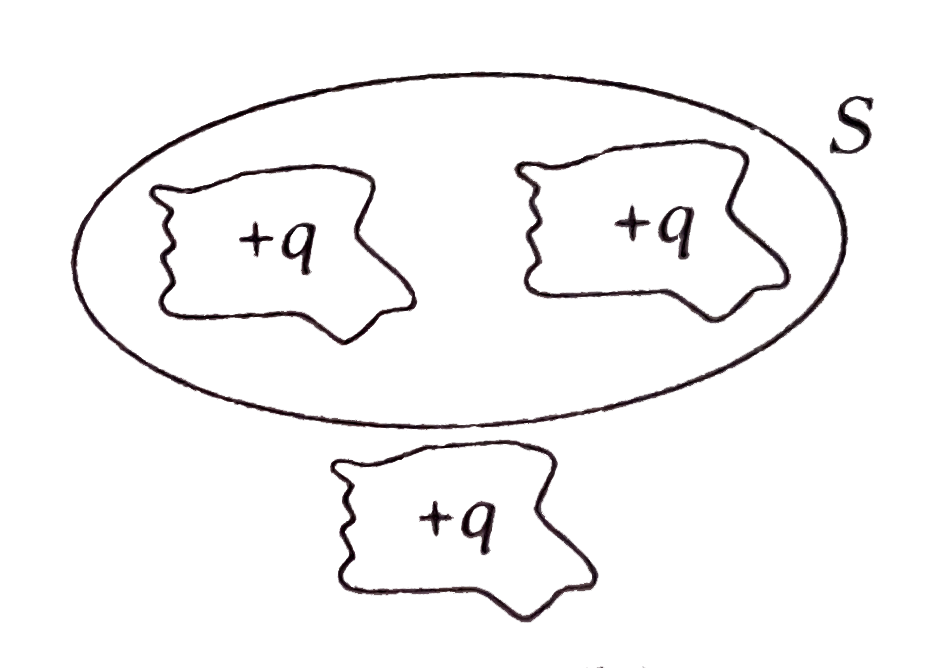
View SolutionFigure shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is

View SolutionA charge q is placed at the centre of the open end of a cylindrical vessel . The flux of the electric field through the surface of the vessel is

View SolutionFind the flux of the electric field through each of the five surfaces of the inclined plane as shown in figure. What is the total flux through the entire closed surface?

View SolutionA non-conducting spherical shell of radius R surrounds a point charge q (q at center). The electric flux through a cap of the shell of half angle θ is:
View Solution
