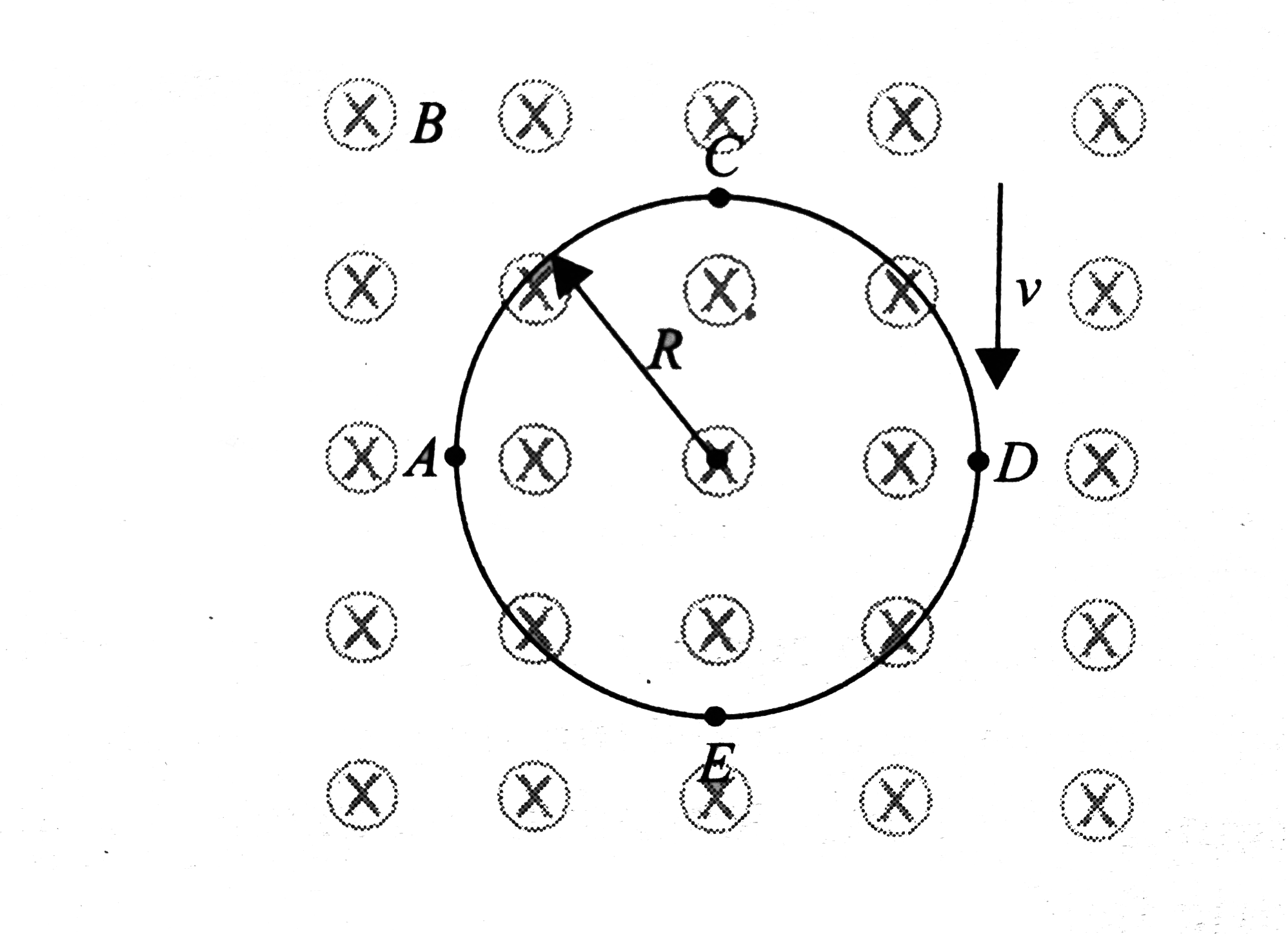
A circular copper ring of radius r translates in its plane with a constant velocity v. A uniform magnetic field B exists in the space in a direction perpendicular of the plane o the ring. Consider different pairs of diametrically opposite points on the ring. (a) Between which pair of points is the emf? (b) Between which pair of points is the emf minimum? What is the value of this minimum emf?
To solve the problem, we need to analyze the situation of a circular copper ring moving in a uniform magnetic field. The key points to consider are the induced electromotive force (emf) between different pairs of diametrically opposite points on the ring.
1. Understanding the Setup:
- We have a circular copper ring of radius r that is translating in its plane with a constant velocity v.
- A uniform magnetic field B is present, directed perpendicular to the plane of the ring.
2. Identifying Points on the Ring:
- Let's label two pairs of diametrically opposite points on the ring as P and Q, and R and S.
- The center of the ring is denoted as C.
3. Calculating EMF Between Points P and Q:
- The distance between points P and Q is the diameter of the ring, which is 2r.
- The induced emf (E) can be calculated using the formula:
E=vBL
where L is the length between the points. For P and Q:
L=2r
- Since the velocity v, magnetic field B, and the length L are mutually perpendicular, we can write:
EPQ=vB(2r)=2vBr
4. Calculating EMF Between Points R and S:
- For the points R and S, the distance L is the diameter of the ring, which is also 2r.
- However, in this case, the displacement vector L is parallel to the velocity vector v.
- Since v and L are parallel, the induced emf between R and S is given by:
ERS=0
- This indicates that the emf is minimum between these points.
5. Conclusion:
- (a) The emf is maximum between points P and Q and is given by 2vBr.
- (b) The emf is minimum between points R and S, and the value of this minimum emf is 0.
Summary of Results:
- Maximum emf between points P and Q: EPQ=2vBr
- Minimum emf between points R and S: ERS=0
- We have a circular copper ring of radius r that is translating in its plane with a constant velocity v.
- A uniform magnetic field B is present, directed perpendicular to the plane of the ring.
- Let's label two pairs of diametrically opposite points on the ring as P and Q, and R and S.
- The center of the ring is denoted as C.
- The distance between points P and Q is the diameter of the ring, which is 2r.
- The induced emf (E) can be calculated using the formula:
E=vBL
where L is the length between the points. For P and Q:
L=2r
- Since the velocity v, magnetic field B, and the length L are mutually perpendicular, we can write:
EPQ=vB(2r)=2vBr
- For the points R and S, the distance L is the diameter of the ring, which is also 2r.
- However, in this case, the displacement vector L is parallel to the velocity vector v.
- Since v and L are parallel, the induced emf between R and S is given by:
ERS=0
- This indicates that the emf is minimum between these points.
- (a) The emf is maximum between points P and Q and is given by 2vBr.
- (b) The emf is minimum between points R and S, and the value of this minimum emf is 0.
- Maximum emf between points P and Q: EPQ=2vBr
- Minimum emf between points R and S: ERS=0
Topper's Solved these Questions
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Questions for short answer9videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Objective 210videos
ELECTROMAGNETIC INDUCTION
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - Objective 27videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - HC VERMA ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Exercise8videos
ELECTROMAGNETIC WAVES
Similar Questions
A ring of radius 2m translates in its plane with a constant velocity 5m/s. A uniform magnetic field 0.1 T exists in the space in a direction perpendicular to the plane of the ring. Consider different pairs of diametrically opposite points on the ring.
(i) Between which pair of points is emf maximum? What is the value of this maximum emf?
(ii) Between which pair of points is the emf minimum? What is the value of this minimum emf?View SolutionA copper wire bent in the shape of a semicircle of radius r translates in its plane with a constant velocity v. A uniform magnetic field B exists in the direction perpendicular to the plane of the wire. Find the emf induced between the ends of the wire if (a) the velocity is perpendicular ot the diameter joining free ends, (b) the velocity is parallel to this diameter.
View Solution
Knowledge Check
- Question 1 - Select One
A conducting ring is placed in a uniform magnetic field with its plane perpendicular to the field . An emf is induced in the ring if
Ait is rotated about its axis.Bit is rotated about its diameter.Cit is not moved.Dit is given translational motion in the field.
A ring of radius R is rolling on a horizontal plane with constant velocity v. There is a constant and uniform magnetic field B which is perpendicular to the plane of the ring. Emf across the lowest point A and right-most point C as shown in the figure will
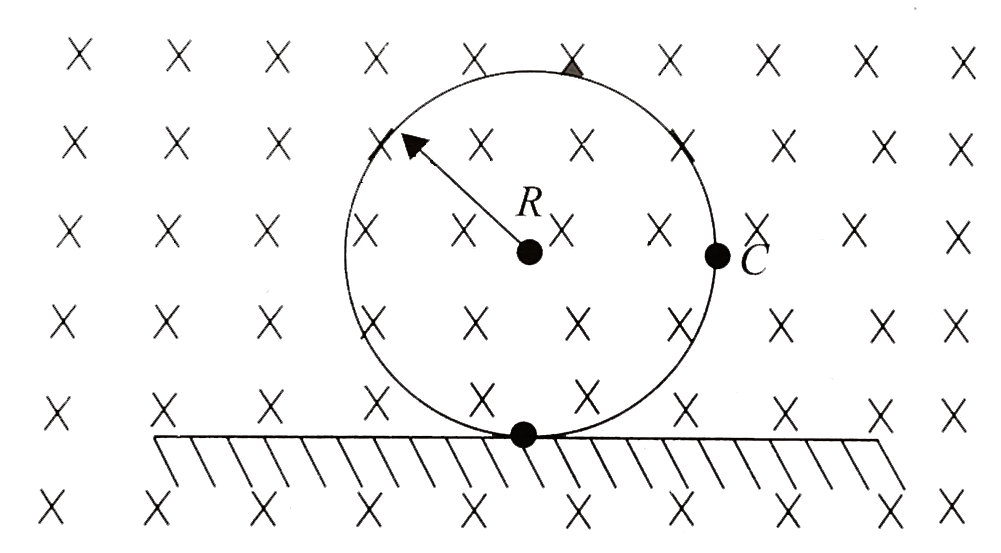 View Solution
View SolutionA ring of radius R moves with a velocity v in a uniform static magnetic field B as shown in diagram. The emf between P and Q is
View SolutionA conducting ring of radius r and resistance R rolls on a horizintal surface with constant velocity v. The magnetic field B is uniform and is normal to the plane of the loop. Choose the correct option.
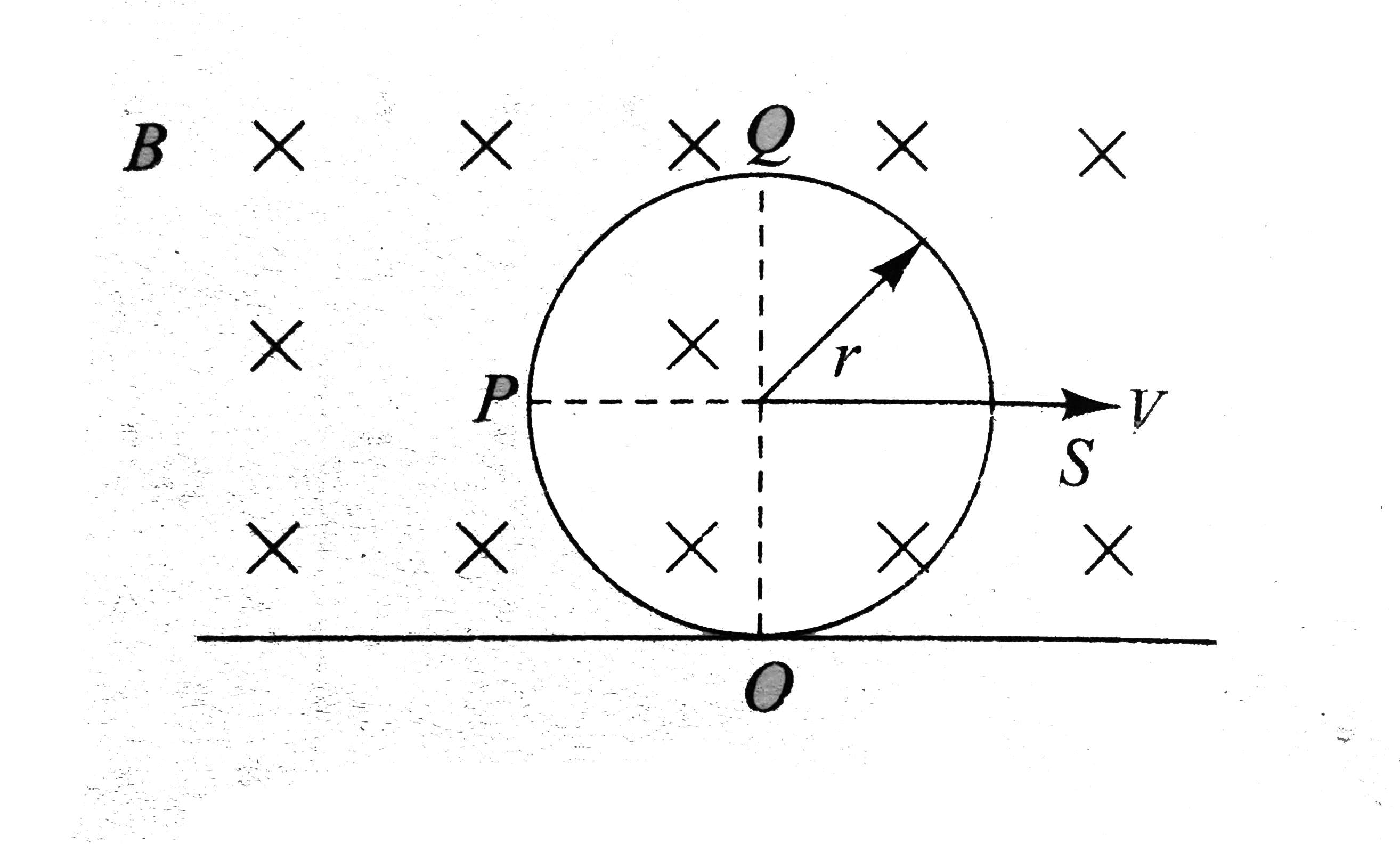 View Solution
View Solution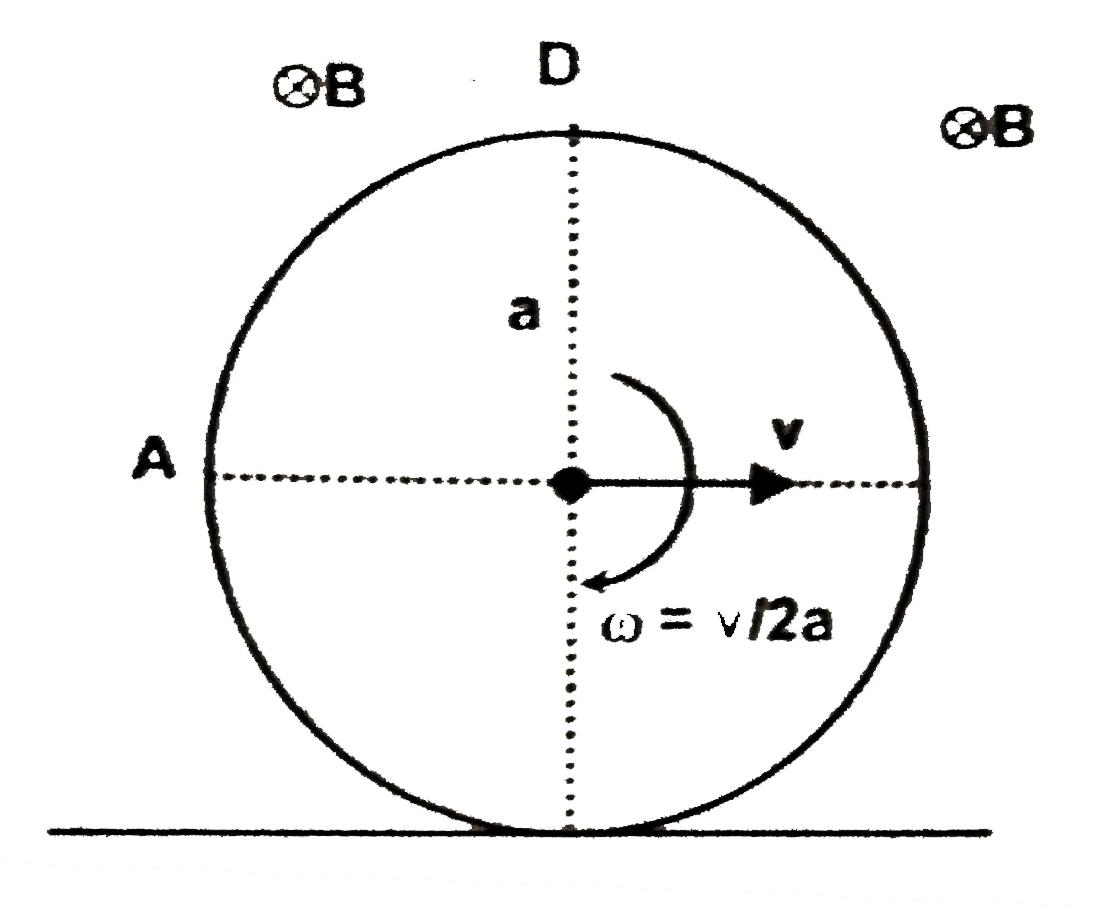
A circular conducting ring of radius 'a' is rolling with slipping on a horizontal surface as shown. A uniform magnetic field B is existing perpendicular to the plane of motion of the ring. The emf iniduced between the points A and D of the ring isView SolutionA vertical conducting ring of radius R falls vertically with a speed V in a horizontal uniform magnetic field B which is perpendicular to the plane of the ring. Which of the following statements is correct?
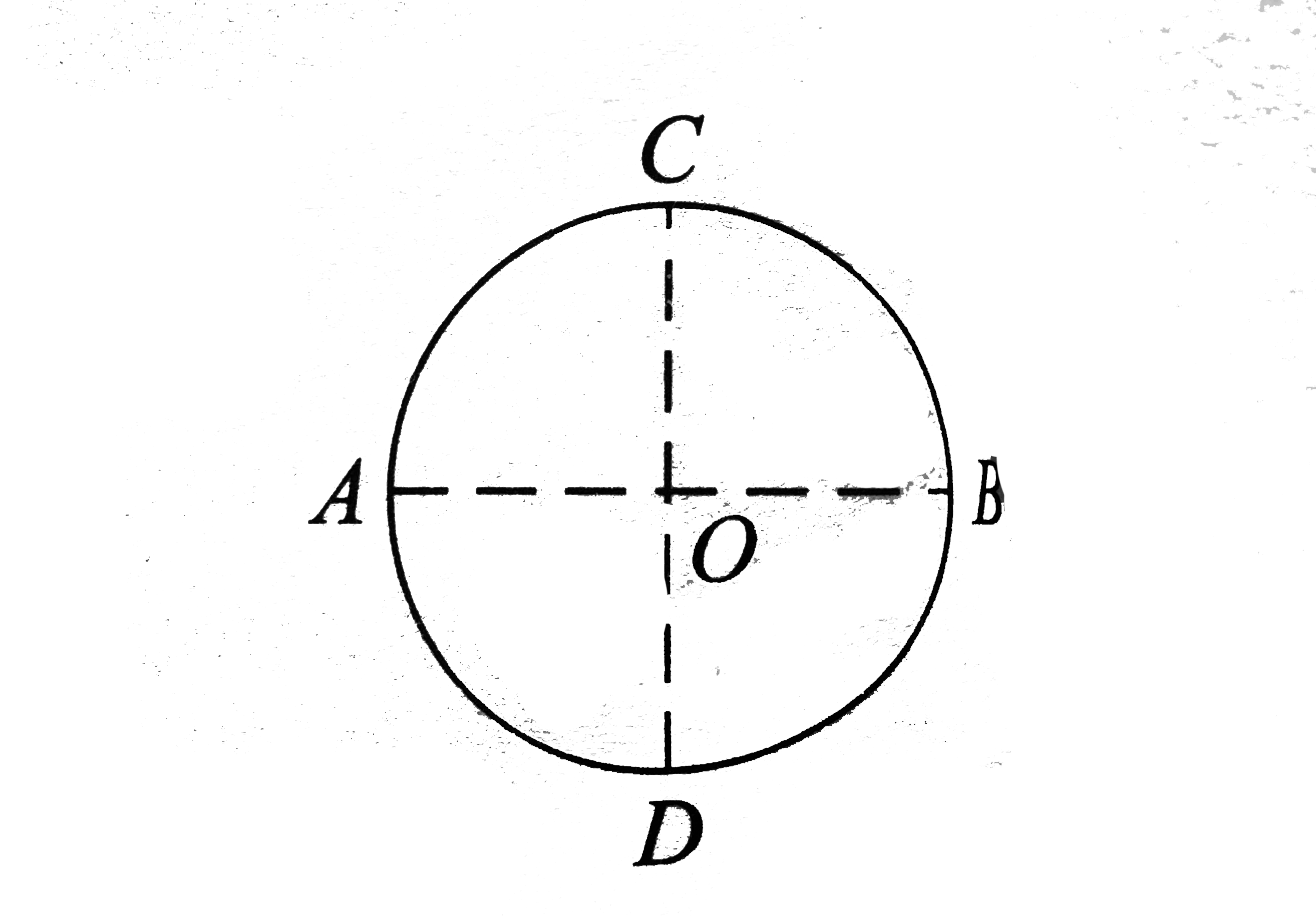 View Solution
View SolutionA semicircle conducting ring of radius R is placed in the xy plane, as shown in Fig. A uniform magnetic field is set up along the x-axis. No emf, will be induced in the ring if
 View Solution
View SolutionA vertical conducting ring ogradius R falls vertically in a horizontal magnetic field of magnitude B. The direction of B is perpendicular to the plane of the ring. When the speed of the ring is v,
 View Solution
View Solution

