For a reaction Akr=0.6M−1min−−−−−−−−→2B
starting with 1 M of 'A' only, concentration of B (in M) after 100 sec. and 200 sec. is respectively?
2 and 4
1 and 2
2 and 3
None of these
The correct Answer is:D
Step by step video & image solution for For a reaction Aoverset(k_(r)=0.6M min^(-1))to2B starting with 1 M of 'A' only, concentration of B (in M) after 100 sec. and 200 sec. is respectively? by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
Consider a reaction A(g)k=0.1M−1min−−−−−−−→2B(g). If initial concentration of A is 0.5 M then select correct graph.
View SolutionIn the following reaction A→B+C, rate constant is 0.001Ms−1. If we start with 1M of A then , cond, of A and B after 10 minutes are respectively.
View SolutionIn the reaction A→B+C, rate constant is 0.001Ms−1. If we start with 1 M of A then conc. Of A and B after 10 minutes are respectively :
View Solution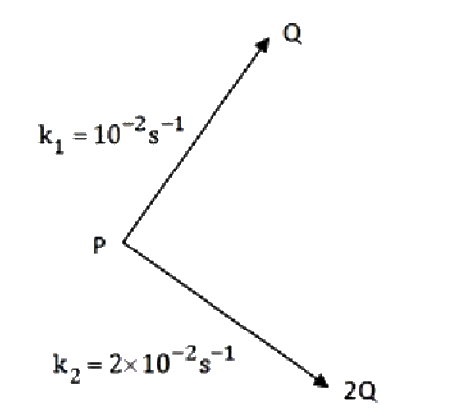
Let the initial concentration of P is 1 M, the concentration of P after 33.33 sec is equal to?View SolutionThe initial rate of zero order reaction of the gaseous equation A(g)→2B(g) is 10−2M−1min If the initial concentration of A is 0.1 M what would be concentration of B after 60 seconds ?
View SolutionFor a reaction scheme Ak1−−→Bk2−−→D , If the rate of formation if B is set to be Zero then the concentration of B is given by :
View SolutionA first order reaction has a rate constant of 0.0051min−1. If we begin with 0.10M concentration of the reaction , what concentration of reactant will remain in solution after 3 hours ?
View SolutionThe rate of a reaction starting with initial concentration of 2×10−3 and 1×10−3M are equal to 2.40×10−4 and 0.60×10−4Ms−1, respectively. Calculate the order or reaction w.r.t. reactant and also the rate constant.
View SolutionThe rate constant of a first order reaction is 4.5×10−2sec−1 What will be the time required for the initial concentration of 0.4 M of the reactant to be reduced to 0.2 M ?
View SolutionThe rate constant of a first order reaction is 4.5×10−2sec−1 What will be the time required for the initial concentration of 0.4 M of the reactant to be reduced to 0.2 M ?
View Solution
