The radioactive decay .21183Bi→20781Ti , takes place in 100L closed vessel at 27∘C Starting with 2 mols of _(83)211Bi(t1/2=130sec) , the presuure development in the vessel after 520 sec will be:
1.875 atm
0.2155atm
0.4618 atm
4.618 atm
The correct Answer is:C
To solve the problem of radioactive decay and pressure development in a closed vessel, we will follow these steps:
Step 1: Determine the number of half-lives that have passed
Given:
- Half-life of 21183Bi = 130 seconds
- Total time = 520 seconds
To find the number of half-lives (n):
n=Total timeHalf-life=520 sec130 sec=4
Hint: To find the number of half-lives, divide the total time by the half-life duration.
Step 2: Calculate the remaining moles of 21183Bi
Starting with 2 moles of 21183Bi:
Using the formula for remaining quantity after n half-lives:
Nt=N0(12)n
Where:
- N0=2 moles (initial amount)
- n=4 (number of half-lives)
Substituting the values:
Nt=2(12)4=2×116=0.125 moles
Hint: Use the formula for radioactive decay to find how much remains after several half-lives.
Step 3: Calculate the amount of 20781Ti produced
The amount of 20781Ti produced is the difference between the initial moles and the remaining moles of 21183Bi:
Moles of 20781Ti=N0−Nt=2−0.125=1.875 moles
Hint: The amount of product formed is equal to the initial amount minus the remaining amount of the reactant.
Step 4: Calculate the total moles in the vessel
The total moles in the vessel after decay:
Total moles=Remaining moles of 21183Bi+Moles of 20781Ti=0.125+1.875=2 moles
Hint: Add the remaining reactant and the product to find the total moles present.
Step 5: Calculate the pressure using the ideal gas law
Using the ideal gas law:
PV=nRT
Where:
- P = pressure (atm)
- V = volume (L) = 100 L
- n = total moles = 2 moles
- R = ideal gas constant = 0.0821 L·atm/(K·mol)
- T = temperature in Kelvin = 27+273=300 K
Rearranging the equation to solve for P:
P=nRTV
Substituting the values:
P=2×0.0821×300100=49.26100=0.4926 atm
Hint: Use the ideal gas law to relate moles, pressure, volume, and temperature.
Final Answer
The pressure development in the vessel after 520 seconds is approximately 0.4926 atm.
Given:
- Half-life of 21183Bi = 130 seconds
- Total time = 520 seconds
n=Total timeHalf-life=520 sec130 sec=4
Starting with 2 moles of 21183Bi:
Using the formula for remaining quantity after n half-lives:
Nt=N0(12)n
Where:
- N0=2 moles (initial amount)
- n=4 (number of half-lives)
Nt=2(12)4=2×116=0.125 moles
The amount of 20781Ti produced is the difference between the initial moles and the remaining moles of 21183Bi:
Moles of 20781Ti=N0−Nt=2−0.125=1.875 moles
The total moles in the vessel after decay:
Total moles=Remaining moles of 21183Bi+Moles of 20781Ti=0.125+1.875=2 moles
Using the ideal gas law:
PV=nRT
Where:
- P = pressure (atm)
- V = volume (L) = 100 L
- n = total moles = 2 moles
- R = ideal gas constant = 0.0821 L·atm/(K·mol)
- T = temperature in Kelvin = 27+273=300 K
P=nRTV
Substituting the values:
P=2×0.0821×300100=49.26100=0.4926 atm
The pressure development in the vessel after 520 seconds is approximately 0.4926 atm.
Similar Questions
83Bi2H(t12=130sec) decays to 81Tl207 by α-emission. In an experiment starting with 5 moles of 83Bi211. how much pressure would be developed in a 350 L closed vessel at 25 C after 760 sec? [Antilog (1.759) = 57 41]
View SolutionThe synthesis of ammonia is given as:
N2(g)+3H2(g)⇔2NH3(g),ΔHɵ=−92.6kJmol−1 given Kc=1.2 and temperature (T)=375∘C
Starting with 2 mol of each (N2,H2andNH3) in 5.0L reaction vessel at 375∘C, predict what is true for the reaction?
View SolutionThe reaction A(g)→B(g)+2C(g) is a first-order reaction with a rate constant of 2.303×10−3s−1. Strating with 0.1 moles of 'A' in a 2 litre vessel, find the concentration of A after 301 sec when the reaction is allowed to take place at constant pressure at 300 K.
View SolutionThe reaction A(g)→B(g)+2C(g) is a first order reaction with rate constant 2.772×10−3sec−1 reaction is started with only 0.1 mol of A in a container with volume 2 litre and is allowed to take place at constant volume and at constant temperature 300K[R=0.082 litre atm mol−1K−1]
(log2=0.30)
Q. Concentration of A after 250 sec will be:
View SolutionFor the 1st order reacton: A(g)→2B(g)+C(s), the t12=24 min. The reaction is carried out taking certain mass of A enclosed in a vessel in which it exerts a pressure of 400 mm Hg. The pressure of the reaction mixture in mm Hg after expiry of 48 min will be
View SolutionMZA(g)→M−8Z−4B(g)+(α− particles )
(α− particles are helium nuclei, so will form helium gas by trapping electrons )
The radioactive disintegration follows first − order kinetics Starting with 1 mol of A in a 1- litre closed flask at 27∘C pressure developed after tw0 half- lives is approximately.
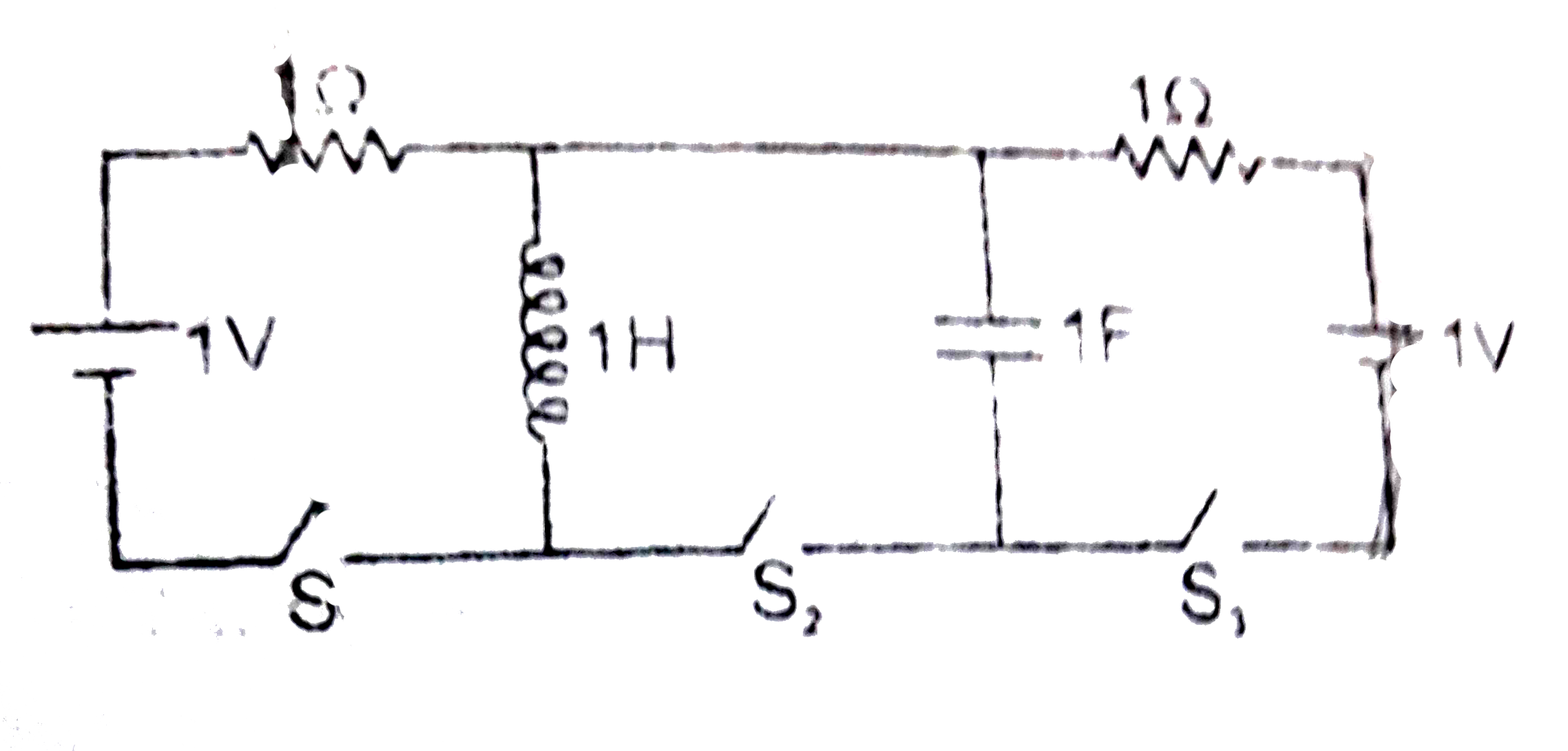
View SolutionIn the circuit shown switches S1 and S2 have been closed for 1sec and S2 remained open.Just after 1 second is over switch S2 is closed and S1,S3 are opened.Find after that instant The charge on the upper plate of the capacitor as function of time taking the instant of switching on of S2 and switching off all time switches to be t=0 is q=x×10−2cos(t+π4).Findout value of x.
View SolutionA heating coil is immersed in a 100 g sample of H2O (l) at a 1 atm and 100∘ C in a closed vessel. In this heating process , 60% of the liquid is converted to the gaseous form at constant pressure of 1 atm . The densities of liquid and gas under these conditions are 1000 kg/m3 and 0.60 kg/m3 respectively . Magnitude of the work done for the process is :
(Take : 1L-atm= 100 J)
View SolutionA tube of uniform cross sectional area 1cm−2 is closed at one end with semi permeable mebrane. A solution having 5 gm glucose per glucose per 100 ml is placed inside the tube and dipped in pure water at 27∘C. When the equilibrium is established. what will be the osmotic pressure height developed in vertical column. Also caiculate molality and molarity of the solution Density of glucose is 1gm/ml.
View Solution1mole ofN2 and 3 moles of H2 are placed in 1L vessel. Find the concentration of NH3 at equilibrium, if the equilibrium constant (Kc) at 400Kis427
View Solution