A disc of mement of inertia I is rotating due to external torque. Its kinetic energy is equal to Kθ2. Where K is the positive constant. Its angular acceleration at an angle θ will be:
7KθI
6KθI
2KθI
4KθI
The correct Answer is:C
To find the angular acceleration α of a disc with moment of inertia I and kinetic energy given by Kθ2, we can follow these steps:
Step 1: Write the expression for kinetic energy
The kinetic energy K of a rotating disc is given by:
K=12Iω2
According to the problem statement, the kinetic energy is also given as:
K=Kθ2
where K is a positive constant.
Step 2: Set the two expressions for kinetic energy equal
Equating the two expressions for kinetic energy, we have:
12Iω2=Kθ2
Step 3: Rearrange the equation to solve for ω2
Multiplying both sides by 2 gives:
Iω2=2Kθ2
Now, dividing both sides by I:
ω2=2Kθ2I
Step 4: Differentiate both sides with respect to θ
To find the angular acceleration α, we need to differentiate ω2 with respect to θ:
ddθ(ω2)=ddθ(2Kθ2I)
Using the chain rule on the left side:
2ωdωdθ=2K⋅2θI
Step 5: Simplify the equation
This simplifies to:
2ωdωdθ=4KθI
Now, we can express dωdθ as:
dωdθ=4Kθ2Iω=2KθIω
Step 6: Relate angular acceleration to the derivative
We know that angular acceleration α is defined as:
α=ωdωdθ
Substituting our expression for dωdθ:
α=ω⋅2KθIω=2KθI
Final Answer
Thus, the angular acceleration α at an angle θ is:
α=2KθI
The kinetic energy K of a rotating disc is given by:
K=12Iω2
According to the problem statement, the kinetic energy is also given as:
K=Kθ2
where K is a positive constant.
Equating the two expressions for kinetic energy, we have:
12Iω2=Kθ2
Multiplying both sides by 2 gives:
Iω2=2Kθ2
Now, dividing both sides by I:
ω2=2Kθ2I
To find the angular acceleration α, we need to differentiate ω2 with respect to θ:
ddθ(ω2)=ddθ(2Kθ2I)
Using the chain rule on the left side:
2ωdωdθ=2K⋅2θI
This simplifies to:
2ωdωdθ=4KθI
Now, we can express dωdθ as:
dωdθ=4Kθ2Iω=2KθIω
We know that angular acceleration α is defined as:
α=ωdωdθ
Substituting our expression for dωdθ:
α=ω⋅2KθIω=2KθI
Thus, the angular acceleration α at an angle θ is:
α=2KθI
Topper's Solved these Questions
- JEE MAINSBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINSExercise:Chemistry
Explore 1 Video
- JEE MAINBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINExercise:All Questions
Explore 452 Videos
- JEE MAINS 2020Book:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINS 2020Exercise:PHYSICS
Explore 250 Videos
Similar Questions
A disc of the moment of inertia 'l1' is rotating in horizontal plane about an axis passing through a centre and perpendicular to its plane with constant angular speed 'ω1' . Another disc of moment of inertia 'I2'. having zero angular speed is placed discs are rotating disc. Now, both the discs are rotating with constant angular speed 'ω2'. The energy lost by the initial rotating disc is
View SolutionIf angular velocity of a disc depends an angle rotated θ as ω=θ2+2θ, then its angular acceleration α at θ=1 rad is :
View SolutionA circular disc of moment of inertia It is rotating in a horizontal plane about its symmetry axis with a constant angular velocity ωi. Another disc of moment of inertia Ib is dropped co-axially onto the rotating disc. Initially, the second disc has zero angular speed. Eventually, both the discs rotate with a constant angular speed ωf. Calculate the energy lost by the initially rotating disc due to friction.
View SolutionA round disc of moment of inertia I2 about its axis perpendicular to its plane and passing through its centre is placed over another disc of moment of inertia I1 rotating with an angular velocity ω about the same axis. The final angular velocity of the combination of discs is.
View SolutionA disc with moment of inertia I is rotating with some angular speed. Second disc is initially at rest. Now second disc with moment of inertia 3I is placed on first disc and starts rotating. Find loss of kinetic energy in fraction
View SolutionA disc is rolling without slipping. The ratio of its rotational kinetic energy and translational kinetic energy would be -
View SolutionA uniform disc of mass m and radius R is pivoted at point P and is free rotate in vertical plane. The centre C of disc is initially in horizontal position with P as shown in figure. If it is released from this position, then its angular acceleration when the line PC is inclined to the horizontal at an angle θ is
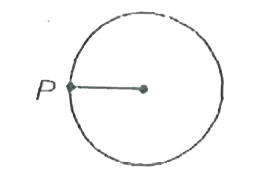
View SolutionA stationary horizontal disc is free to rotate about its axis. When a torque is applied on it its, kinetic energy as a function of θ, where θ is the angle by which it has rotated, is given as kθ. If its moment of inertia is I then the angular acceleration of the disc is :
View SolutionA disc with moment of inertial I is rotating with some angular speed. Second disc is initially at rest. Now second disc with moment of inertia 3I is placed on first disc and starts rotating. Find loss of kinetic energy in fraction
View SolutionIf a body is rolling on a surface without slipping such that its kinetic energy of translation is equal to kinetic energy of rotation then it is a
View Solution