A thin circular plate of mass M and radius R has its density varying as ρ(r)=ρ0r with ρ0 as constant and r is the distance from its center. The moment of Inertia of the circular plate about an axis perpendicular to the plate and passing through its edge is I=aMR2 The value of the coefficient a is :
1/2
3/5
8/5
3/2
The correct Answer is:C
To find the moment of inertia of a thin circular plate with a varying density, we can follow these steps:
1. Understanding the Density Variation:
The density of the circular plate varies as ρ(r)=ρ0r, where ρ0 is a constant and r is the distance from the center of the plate.
2. Element of Mass:
We consider a thin ring of radius r and thickness dr within the circular plate. The area of this thin ring is given by:
dA=2πrdr
The mass of this ring can be expressed as:
dm=ρ(r)⋅dA=ρ0r⋅(2πrdr)=2πρ0r2dr
3. Moment of Inertia of the Ring:
The moment of inertia dI of this thin ring about an axis perpendicular to the plate and passing through its center is given by:
dI=r2dm=r2⋅(2πρ0r2dr)=2πρ0r4dr
4. Total Moment of Inertia:
To find the total moment of inertia I, we integrate dI from 0 to R:
I=∫R0dI=∫R02πρ0r4dr
Evaluating the integral:
I=2πρ0(r55)R0=2πρ0R55=2πρ0R55
5. Finding Total Mass:
The total mass M of the plate can be found by integrating dm from 0 to R:
M=∫R0dm=∫R02πρ0r2dr=2πρ0(r33)R0=2πρ0R33
6. Expressing ρ0 in terms of M:
Rearranging the equation for mass:
ρ0=3M2πR3
7. Substituting ρ0 back into the Moment of Inertia:
Substitute ρ0 into the expression for I:
I=2π(3M2πR3)R55=3MR25
8. Comparing with the Given Form:
The moment of inertia is given in the form I=aMR2. From our calculation:
I=35MR2
Therefore, we can identify:
a=35
Final Answer:
The value of the coefficient a is 35.
The density of the circular plate varies as ρ(r)=ρ0r, where ρ0 is a constant and r is the distance from the center of the plate.
We consider a thin ring of radius r and thickness dr within the circular plate. The area of this thin ring is given by:
dA=2πrdr
The mass of this ring can be expressed as:
dm=ρ(r)⋅dA=ρ0r⋅(2πrdr)=2πρ0r2dr
The moment of inertia dI of this thin ring about an axis perpendicular to the plate and passing through its center is given by:
dI=r2dm=r2⋅(2πρ0r2dr)=2πρ0r4dr
To find the total moment of inertia I, we integrate dI from 0 to R:
I=∫R0dI=∫R02πρ0r4dr
Evaluating the integral:
I=2πρ0(r55)R0=2πρ0R55=2πρ0R55
The total mass M of the plate can be found by integrating dm from 0 to R:
M=∫R0dm=∫R02πρ0r2dr=2πρ0(r33)R0=2πρ0R33
Rearranging the equation for mass:
ρ0=3M2πR3
Substitute ρ0 into the expression for I:
I=2π(3M2πR3)R55=3MR25
The moment of inertia is given in the form I=aMR2. From our calculation:
I=35MR2
Therefore, we can identify:
a=35
The value of the coefficient a is 35.
Topper's Solved these Questions
- JEE MAINSBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINSExercise:Chemistry
Explore 1 Video
- JEE MAINBook:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINExercise:All Questions
Explore 452 Videos
- JEE MAINS 2020Book:JEE MAINS PREVIOUS YEAR ENGLISHChapter:JEE MAINS 2020Exercise:PHYSICS
Explore 250 Videos
Similar Questions
Consider a uniform square plate of of side and mass m. The moment of inertia of this plate about an axis perpendicular to its plane and passing through one of its corners is -
View SolutionFrom a circular disc of radius R and mass 9 M , a small disc of radius R/3 is removed from the disc. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through O is

View Solution
Knowledge Check
From a uniform circular disc of radius R and mass 9M, a small disc of radius R3 is removed as shown in the figure. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through centre of disc is :
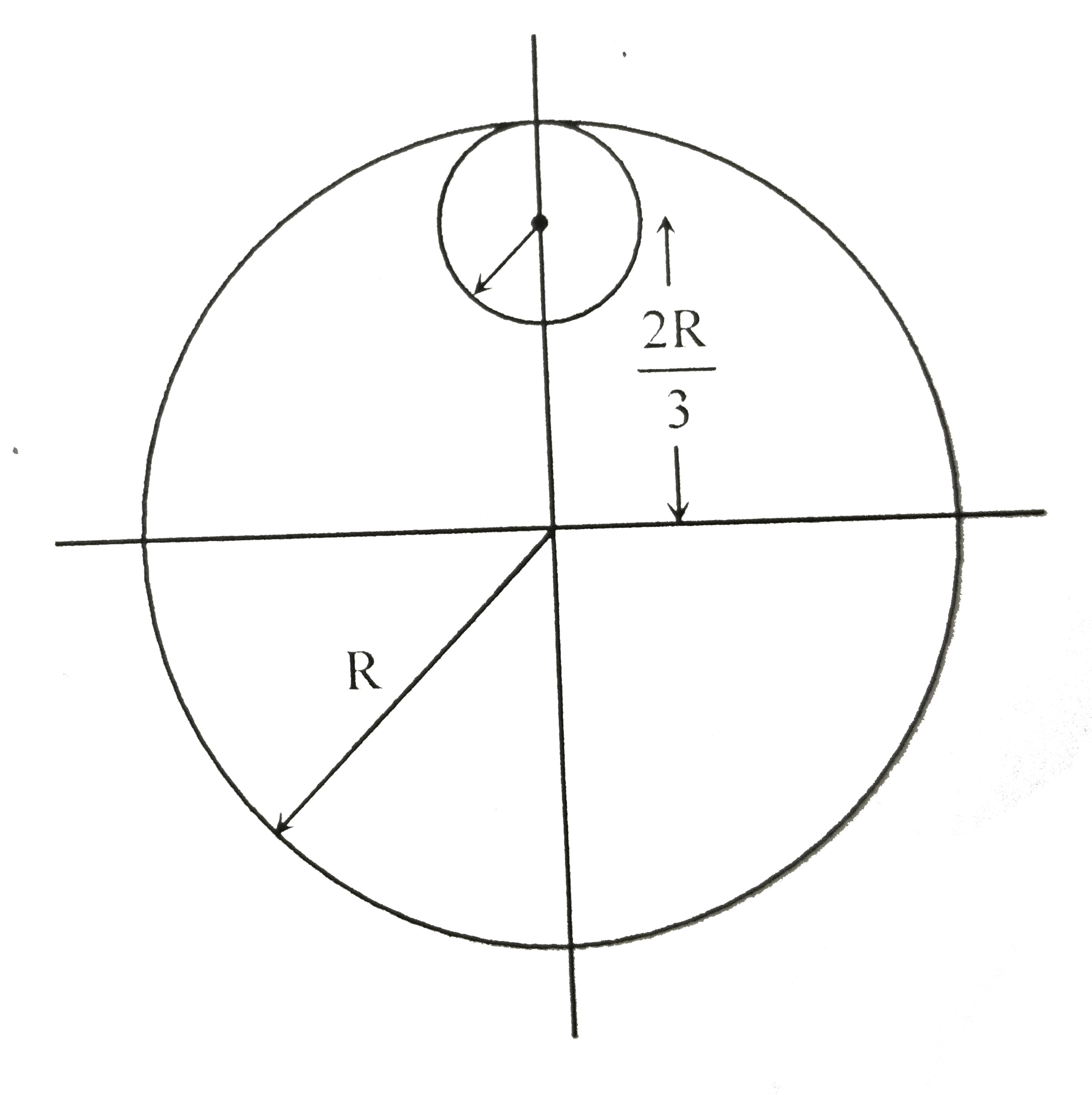 A4MR2B409MR2C40MR2D379MR2
A4MR2B409MR2C40MR2D379MR2
From a disc of radius R, a concentric circular portion of radius r is cut out so as to leave an annular disc of mass M. The moment of inertia of this annular disc about the axis perpendicular to its plane and passing through its centre of gravity is
View SolutionThe moment of inertia of thin uniform circular disc about one of the diameter is I. Its moment of inertia about an axis perpendicular to the circular surface and passing through its centre is
View SolutionMoment of inertia of a circular wire of mass M and radius R about its diameter is
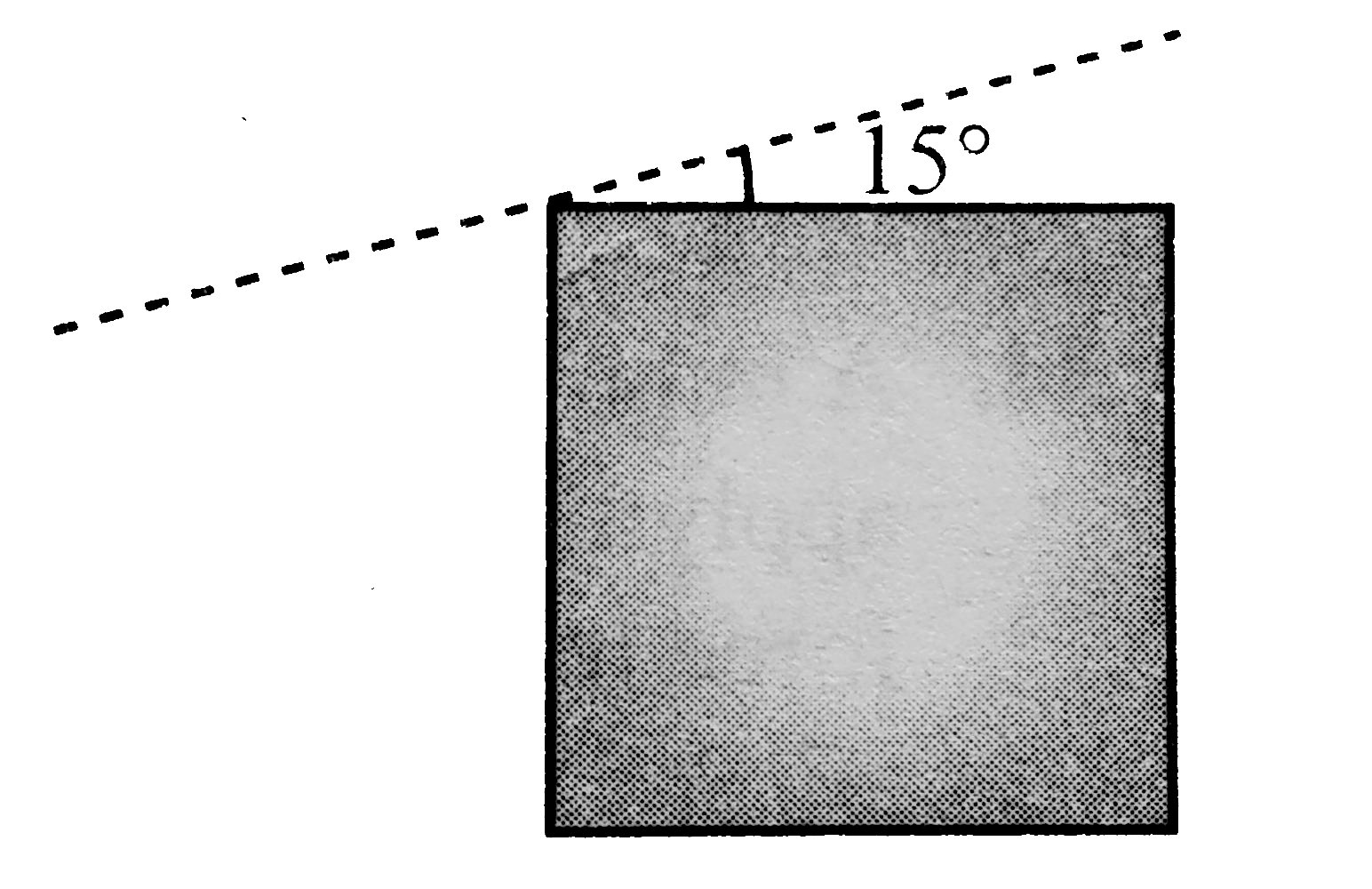
View SolutionA square plate of mass M and edge L is shown in the figure. The moment of inertia of the plate about the axis in the plane of plate and passing through one of its vertex making an angle 15∘ horizontal is
View SolutionA hole of radius R/2 is removed from a thin circular plate of radius R and mass M. The moment of inertia of the remaining plate about an axis through centre O and perpendicular to the plane of the plate is

View SolutionThe surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as ρ(r)=A+Br. Find its moment of inertia about the line perpendicular to the plane of the disc through its centre.
View SolutionThe surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as ρ(r)=A+Br. Find its moment of inertia about the line perpendicular to the plane of the disc through its centre.
View Solution