A parallel plate capacitor having capacitance 12pF is charged bya battery to a potential difference of 10V between its plates. The charging battery is now disonnected and a porcelain slab of dielectric constant 6.5 is slipped between the plates. The work done by the capacitor on the slab is
692 pJ
508pJ
560pJ
600 pJ
Step by step video & image solution for A parallel plate capacitor having capacitance 12pF is charged bya battery to a potential difference of 10V between its plates. The charging battery is now disonnected and a porcelain slab of dielectric constant 6.5 is slipped between the plates. The work done by the capacitor on the slab is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
Similar Questions
Figure shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
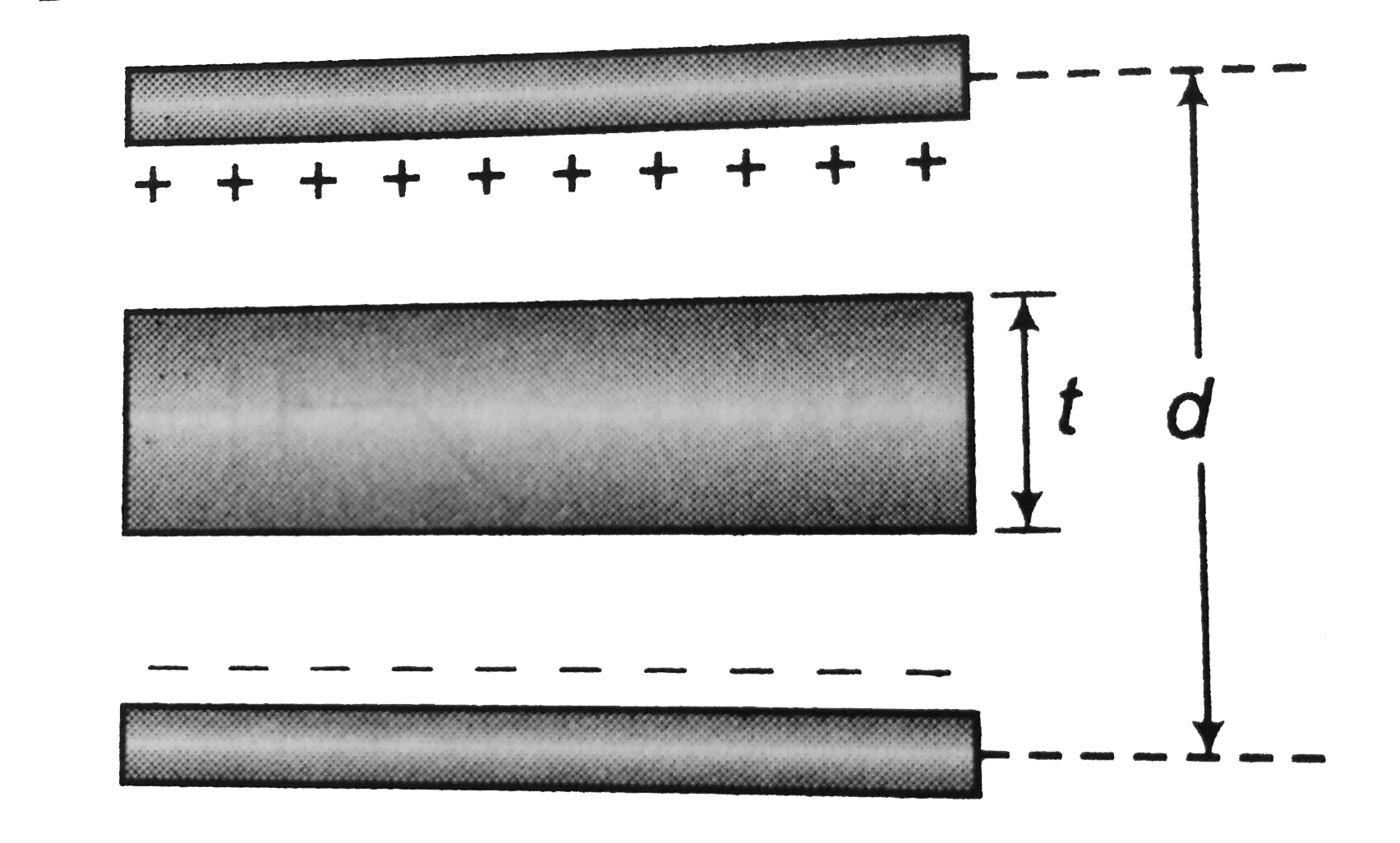
The electric field in the gaps between the plates and the electric slab will beView SolutionFigue shows a parallel plate capacitor with plate area A and plate separation d. A potential difference is being applied between the plates. The battery is then disconnected and a dielectric slab of dieletric constant K is placed in between the plates of the capacitor as shown.
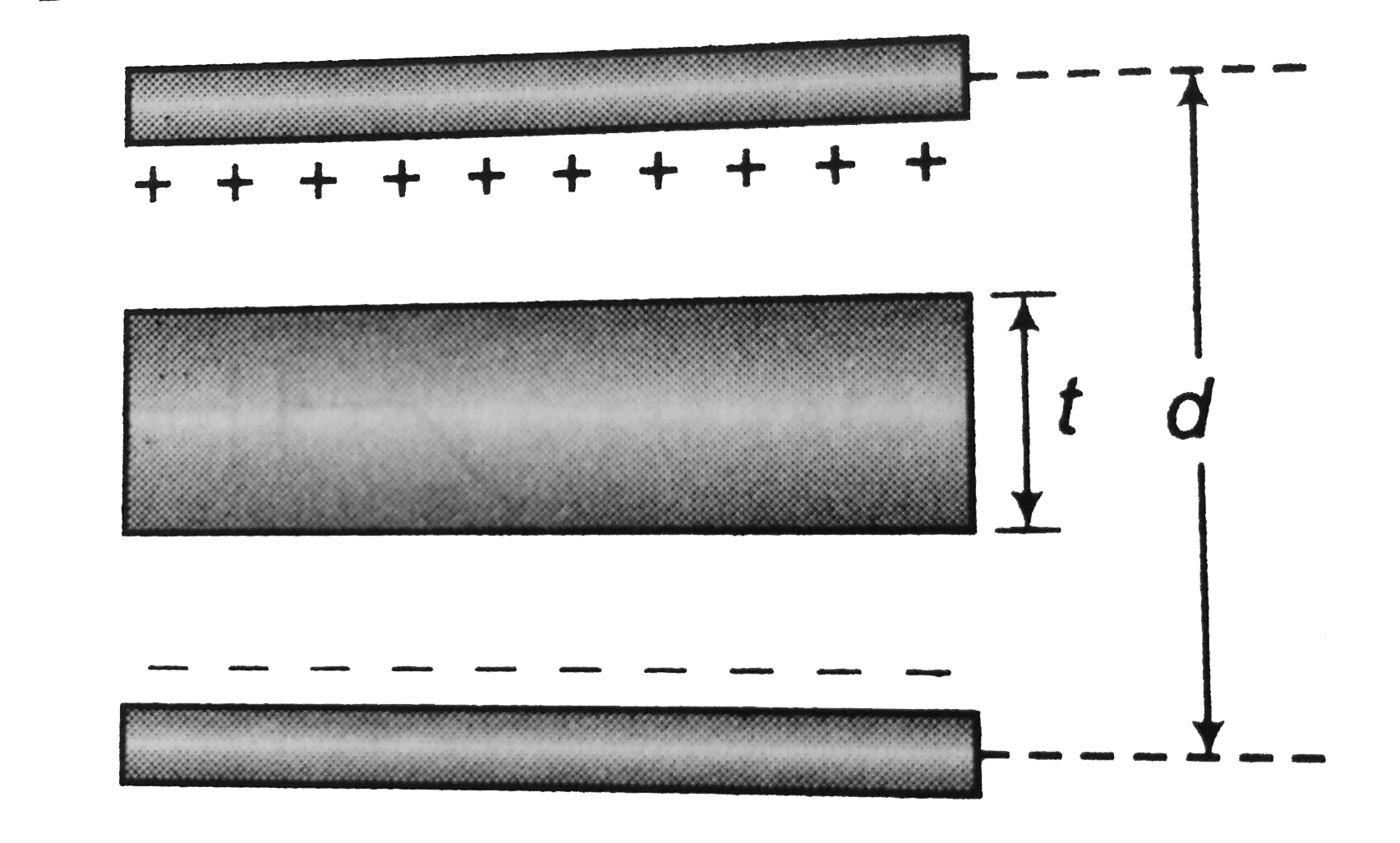
The electric field in the dielectric slab isView SolutionIn a parallel plate capacitor of capacitor 1μF two plates are given charges 2μC and 4μC the potential difference between the plates of the capacitor is
_2019_CBT2_E01_002_Q01.png)
View SolutionA parallel - plate capacitor of plate area A and plate separation d is charged to a potential difference V and then the battery is disconnected . A slab of dielectric constant K is then inserted between the plate of the capacitor so as to fill the space between the plate .Find the work done on the system in the process of inserting the slab.
View SolutionA capacitor having a capacitance of 100μf is changed to a potential difference of 50V.(a) What is the magnitude of the charge on each plate? (b)The charging battery is disconnected and a dielectric of dielectric constant 2.5 is inserted . Calculate the new potential difference between the plate .(c) What charge would have produced this potential difference in absence of the dielectric slab.(d) Find the charge induced at a surface of the dielectric slab.
View SolutionA capacitor having a capacitance of 100μf is changed to a potential difference of 50V.(a) What is the magnitude of the charge on each plate? (b)The charging battery is disconnected and a dielectric of dielectric constant 2.5 is inserted . Calculate the new potential difference between the plate .
View SolutionA capacitor is charged by using a battery which is then disconnected. A dielectric slab is then slipped between the plates, which results in
View SolutionA parallel plate capacitor of plate area A and plate separation d is charged to potential difference V and then the battery is disconnected. A slab of dielectric constant K is then inserted between the plates of the capacitor so as to fill the space between the plates. If Q, E and W denote respectively, the magnitude of charge on each plate, the electric field between the plates (after the slab is inserted), and work done on the system, in question, in the process of inserting the slab, then
View SolutionA parallel plate capacitor each with plate area A and separation ‘d’ is charged to a potential difference V. The battery used to charge it is then disconnected. A dielectric slab of thickness d and dielectric constant K is now placed between the plates. What change if any, will take place in
(i) charge on the plates
(ii) electric field intensity between the plates,
(iii) capacitance of the capacitor
Justify your answer in each caseView SolutionA dielectric slab is inserted between the plates of an isolated capacitor. The force between the plates will
View Solution