A particle of mass m and positive charge q is projected with a speed of v0 in y–direction in the presence of electric and magnetic field are in x–direction. Find the instant of time at which the speed of particle becomes double the initial speed.
√3mv0qE
√2mv0qE
mv0qE
√3mv02qE
The correct Answer is:A
To solve the problem, we need to analyze the motion of a charged particle in the presence of electric and magnetic fields. The particle is projected in the y-direction, while both fields are aligned in the x-direction. Our goal is to find the time at which the speed of the particle becomes double its initial speed.
1. Understanding Initial Conditions:
- The particle has a mass m and a charge q.
- It is projected with an initial speed v0 in the y-direction.
- The electric field E and magnetic field B are both in the x-direction.
2. Force Analysis:
- The magnetic force does not do any work on the particle because it acts perpendicular to the direction of motion. Therefore, it does not change the speed of the particle.
- The electric field exerts a force on the particle given by F=qE.
- The acceleration a of the particle in the x-direction can be expressed as:
ax=Fm=qEm
3. Velocity in the x-direction:
- The initial velocity in the x-direction vx0=0.
- The velocity in the x-direction at time t can be expressed as:
vx=vx0+axt=0+qEmt=qEmt
4. Velocity in the y-direction:
- The velocity in the y-direction remains constant:
vy=v0
5. Finding the Resultant Speed:
- The resultant speed vnet is given by:
vnet=√v2x+v2y
- We want to find the time when the resultant speed is double the initial speed:
vnet=2v0
- Squaring both sides gives:
(2v0)2=v2x+v2y
4v20=v2x+v20
- Rearranging gives:
v2x=4v20−v20=3v20
- Taking the square root:
vx=√3v0
6. Finding Time t:
- We already have the expression for vx:
vx=qEmt
- Setting this equal to √3v0:
√3v0=qEmt
- Solving for t:
t=√3mv0qE
Final Answer:
The time at which the speed of the particle becomes double the initial speed is:
t=√3mv0qE
- The particle has a mass m and a charge q.
- It is projected with an initial speed v0 in the y-direction.
- The electric field E and magnetic field B are both in the x-direction.
- The magnetic force does not do any work on the particle because it acts perpendicular to the direction of motion. Therefore, it does not change the speed of the particle.
- The electric field exerts a force on the particle given by F=qE.
- The acceleration a of the particle in the x-direction can be expressed as:
ax=Fm=qEm
- The initial velocity in the x-direction vx0=0.
- The velocity in the x-direction at time t can be expressed as:
vx=vx0+axt=0+qEmt=qEmt
- The velocity in the y-direction remains constant:
vy=v0
- The resultant speed vnet is given by:
vnet=√v2x+v2y
- We want to find the time when the resultant speed is double the initial speed:
vnet=2v0
- Squaring both sides gives:
(2v0)2=v2x+v2y
4v20=v2x+v20
- Rearranging gives:
v2x=4v20−v20=3v20
- Taking the square root:
vx=√3v0
- We already have the expression for vx:
vx=qEmt
- Setting this equal to √3v0:
√3v0=qEmt
- Solving for t:
t=√3mv0qE
The time at which the speed of the particle becomes double the initial speed is:
t=√3mv0qE
Topper's Solved these Questions
Similar Questions
A particle having mass m and charge q is released from the origin in a region in which ele field and magnetic field are given by B=−B0ˆjand E=E0ˆk.
Find the y- component of the velocity and the speed of the particle as a function of it z-coordinate.
View SolutionA particle of mass m and having a positive charge q is projected from origin with speed v0 along the positive X-axis in a magnetic field B = −B0ˆK, where B0 is a positive constant. If the particle passes through (0,y,0), then y is equal to
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity
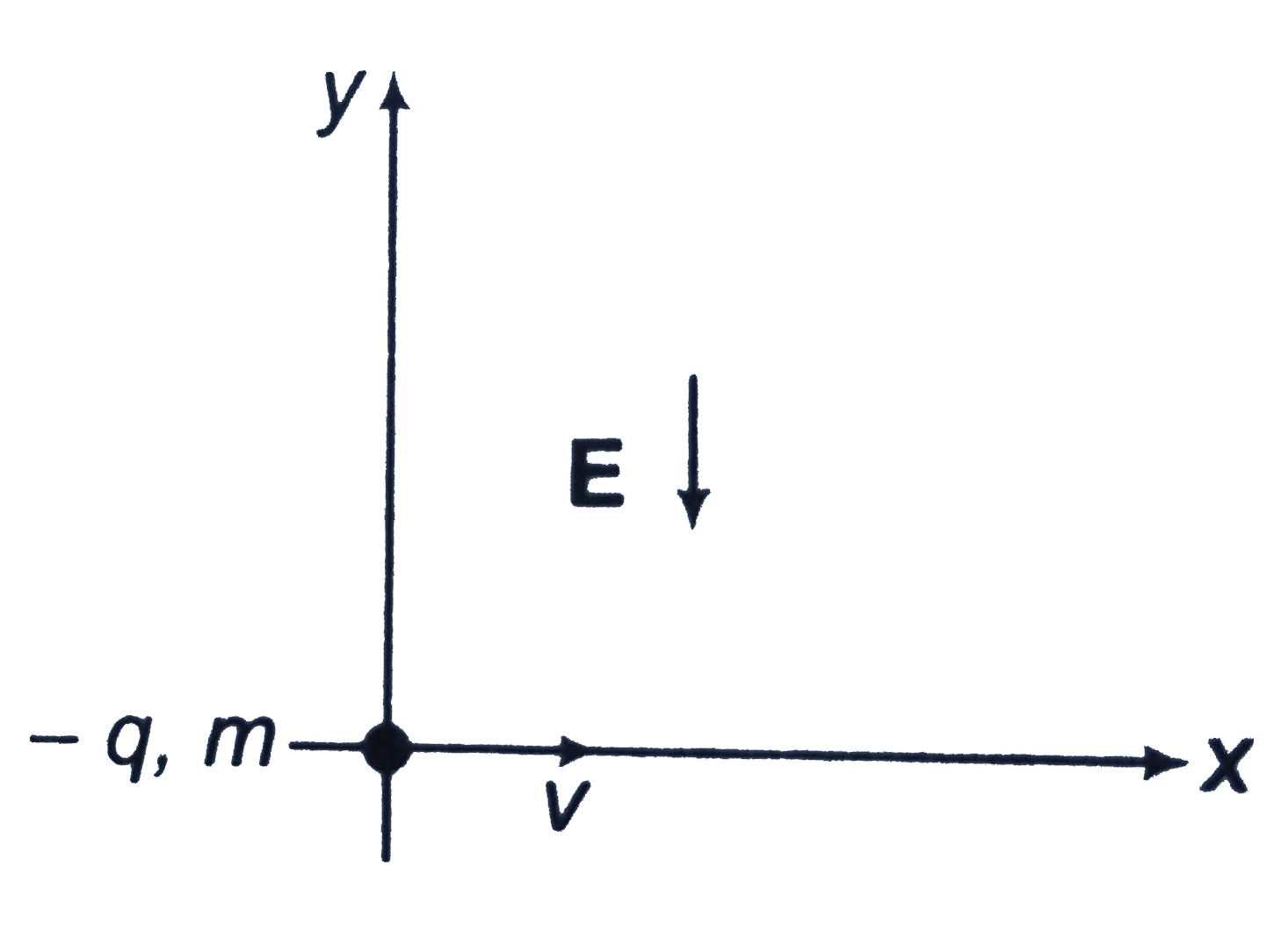
View SolutionA particle of mass m and charge −q is projected from the origin with a horizontal speed v into an electric field of intensity E directed downward. Choose the wrong statement. Neglect gravity

View SolutionA particle having mass m and charge q is released from the origin in a region in which electric field and magnetic field are given by
→B=−B0→Jand→E−E0→K.
Find the speed of the particle as a function of its z-coordinate.
View SolutionA charge particle travels along a straight line with a speed v in region where both electric field E and magnetic field b are present.It follows that
View SolutionPositively charged particles are projected into a magnetic field. If the direction of the magnetic field is along the direction of motion of the charged particles, the particles get
View SolutionA charged particle (charge q, mass m) has velocity v0 at origin in +x direction. In space there is a uniform magnetic field B in -z direction. Find the y coordinate of particle when is crosses y axis.
View SolutionFind the condition under which the charged particles moving with different speeds in the presence of electric and magnetic field vectors can be used to select charged particles of a particular speed.
View SolutionA positive charge particle of mass m and charge q is projected with velocity v as shown in Fig. If radius of curvature of charge particle in magnetic field region is greater than d, then find the time spent by the charge particle in magnetic field.
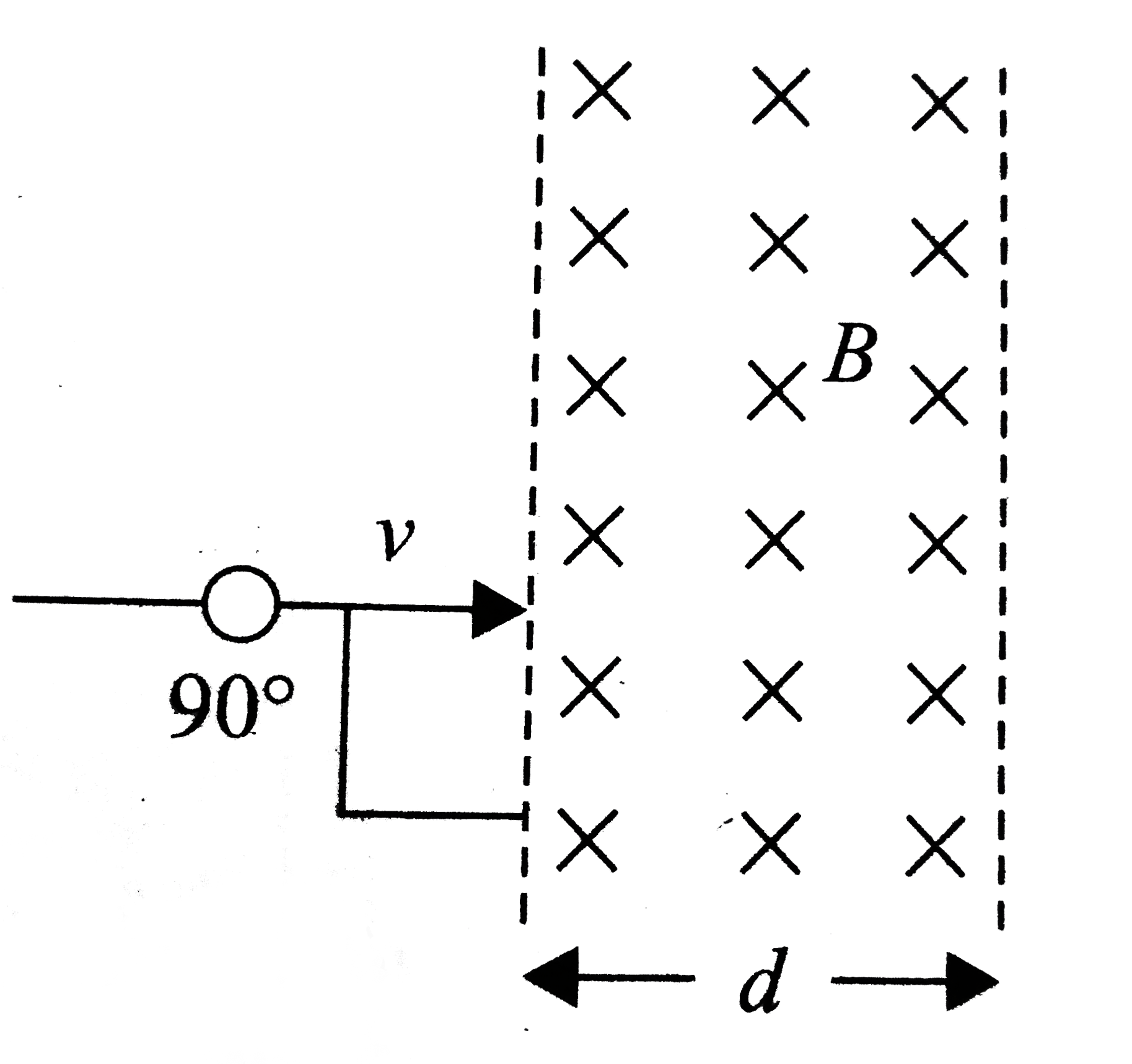
View Solution
