A block of mass m is connected at one end of spring fixed at other end having natural length l0 and spring constant K. The block is rotated with constant angular speed (ω) in gravity free space. Find the elongation in spring.
l0mω2k−mω2
l0mω2k+mω2
l0mω2k−mω
l0mω2k+mω
The correct Answer is:A
To solve the problem of finding the elongation in the spring when a block of mass m is rotated with a constant angular speed ω in gravity-free space, we can follow these steps:
1. Identify the Forces Involved:
- The block is rotating in a circular path, and the spring force acts as the centripetal force required for this circular motion.
2. Define the Variables:
- Let l0 be the natural length of the spring.
- Let K be the spring constant.
- Let x be the elongation of the spring.
- The total length of the spring when elongated is L=l0+x.
3. Centripetal Force Requirement:
- The required centripetal force for the block to maintain circular motion is given by:
Fc=mω2r
- Here, r is the radius of the circular path, which in this case is equal to the total length of the spring L:
r=l0+x
4. Spring Force:
- The force exerted by the spring when it is elongated is given by Hooke's law:
Fs=Kx
5. Set the Forces Equal:
- Since the spring force provides the necessary centripetal force, we can set them equal:
Kx=mω2(l0+x)
6. Rearranging the Equation:
- Expanding the right-hand side:
Kx=mω2l0+mω2x
- Rearranging gives:
Kx−mω2x=mω2l0
- Factoring out x:
(K−mω2)x=mω2l0
7. Solving for Elongation x:
- Finally, we can solve for x:
x=mω2l0K−mω2
Final Result:
The elongation in the spring when the block is rotated with angular speed ω is given by:
x=mω2l0K−mω2
- The block is rotating in a circular path, and the spring force acts as the centripetal force required for this circular motion.
- Let l0 be the natural length of the spring.
- Let K be the spring constant.
- Let x be the elongation of the spring.
- The total length of the spring when elongated is L=l0+x.
- The required centripetal force for the block to maintain circular motion is given by:
Fc=mω2r
- Here, r is the radius of the circular path, which in this case is equal to the total length of the spring L:
r=l0+x
- The force exerted by the spring when it is elongated is given by Hooke's law:
Fs=Kx
- Since the spring force provides the necessary centripetal force, we can set them equal:
Kx=mω2(l0+x)
- Expanding the right-hand side:
Kx=mω2l0+mω2x
- Rearranging gives:
Kx−mω2x=mω2l0
- Factoring out x:
(K−mω2)x=mω2l0
- Finally, we can solve for x:
x=mω2l0K−mω2
The elongation in the spring when the block is rotated with angular speed ω is given by:
x=mω2l0K−mω2
Topper's Solved these Questions
Similar Questions
A particle of mass m is fixed to one end of a massless spring of spring constant k and natural length l0. The system is rotated about the other end of the spring with an angular velocity ω ub gravity-free space. The final length of spring is
View SolutionA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
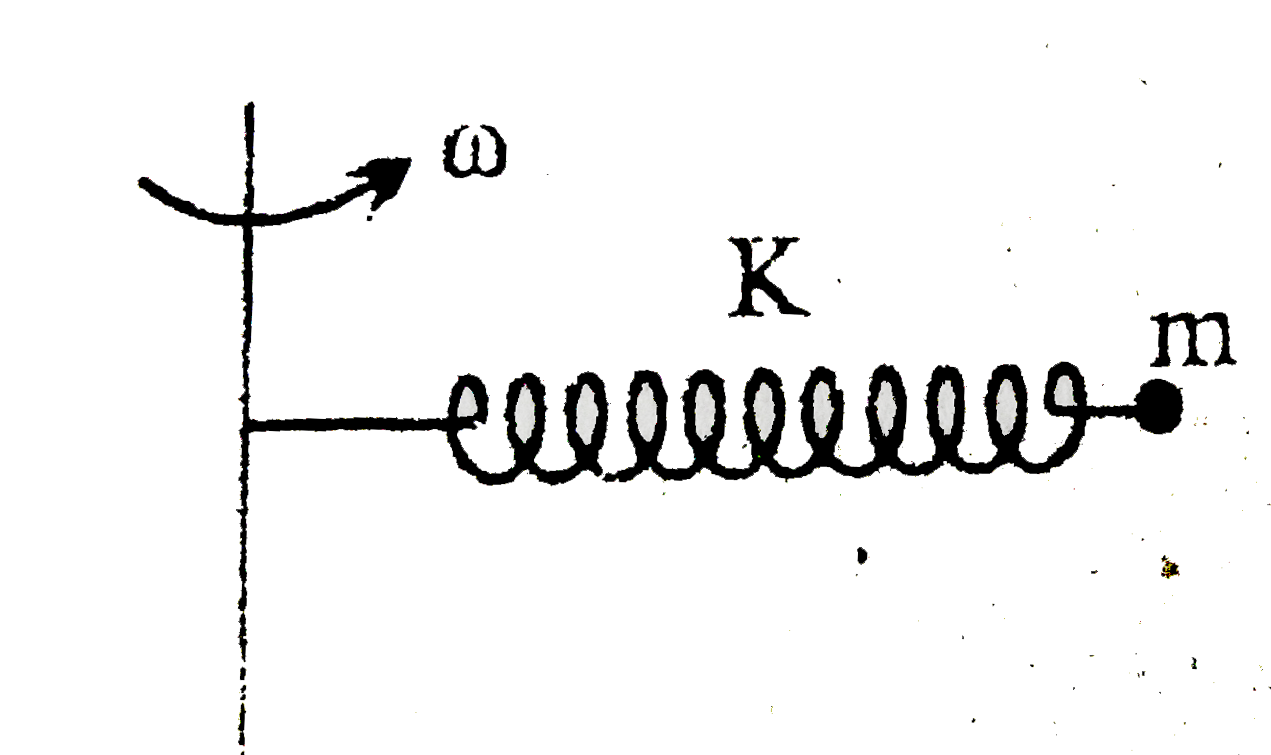
View SolutionA block of mass m fitted with a light spring of stiffness k and natural length l0 kept on a smooth radial groove made on a disc rotaing with a constant angular axis . If the block is released slowly . Find the maximum elongation of the spring

View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionThe block of mass m is released when the spring was in its natrual length. Spring constant is k. Find the maximum elongation of the spring.

View SolutionFigure shows a smooth track, a part of which is a circle of radius r. A block of mass m is pushed against a spring of spring constant k fixed at the left end and is then released. Find the initial compression of the spring so that the block presses the track with a force mg when it reaches the point P, where the radius of the track is horizontal.
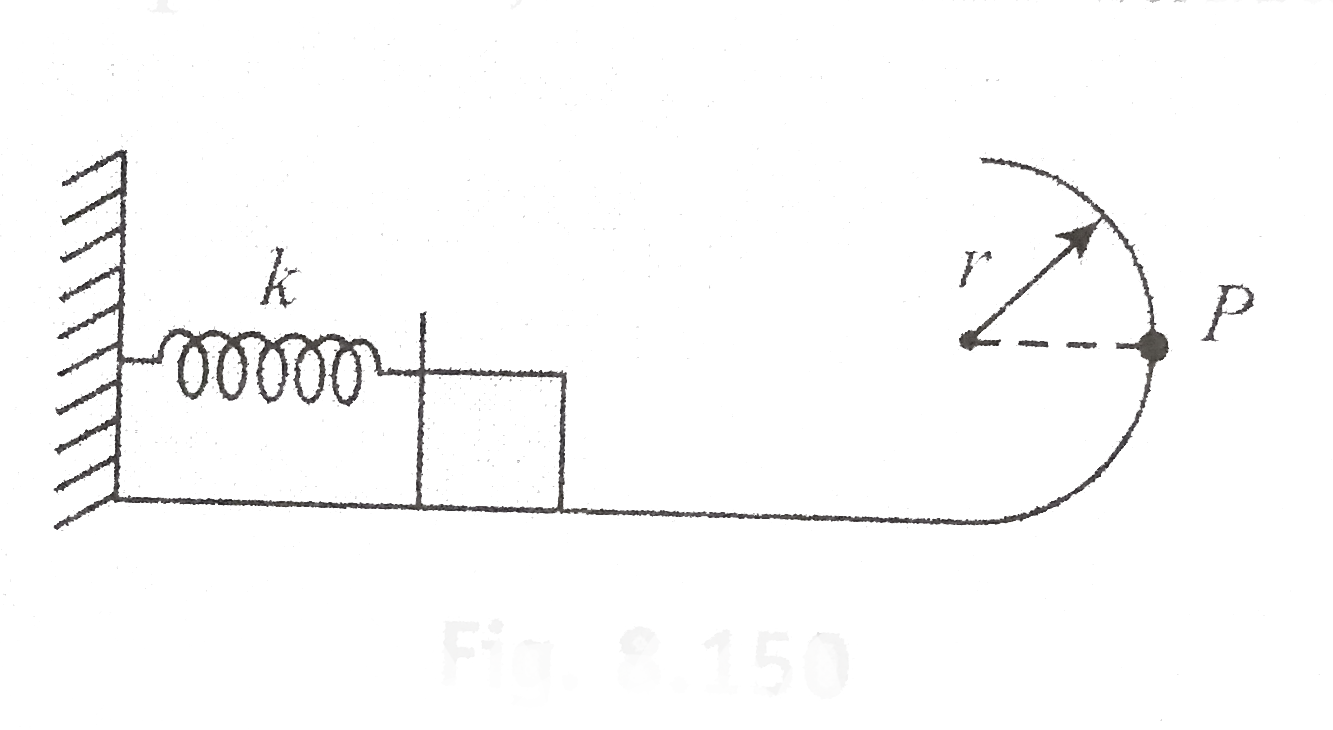
View SolutionA block of mass m is connected to another block of mass M by a massless spring of spring constant k. the blocks are kept of a smooth horizontal plane and are at rest. The spring is unstretched when a constant force F starts acting on the block of mass M of pull it. Find the maximum extension of the spring

View SolutionA block of mass m suspended from a spring of spring constant k. Find the amplitude of S.H.M.
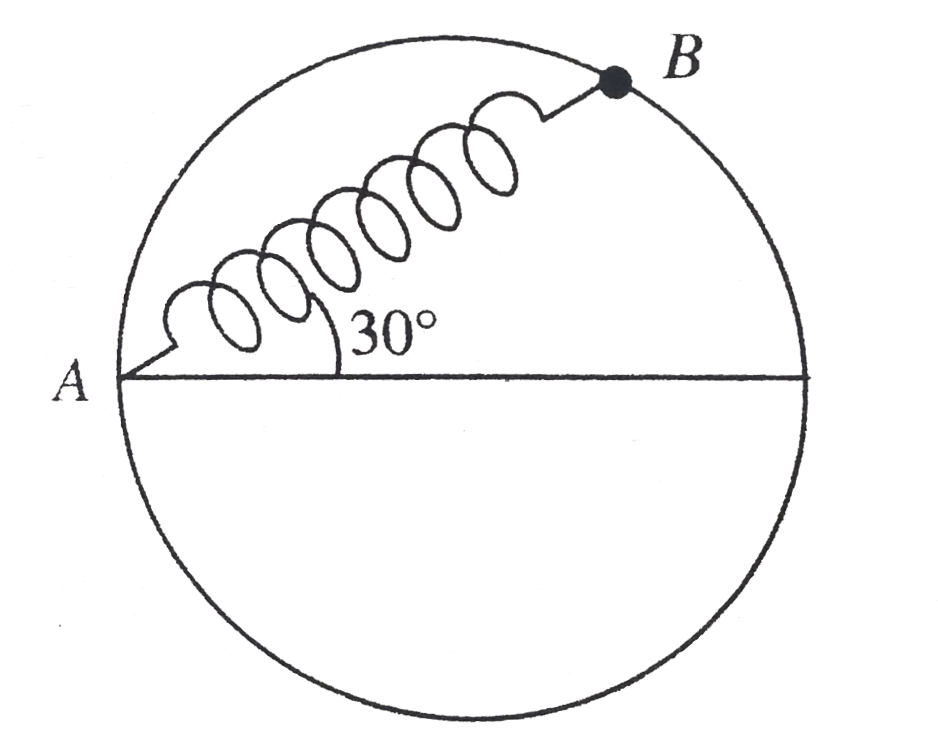
View SolutionA bead of mass m is attached to one end of a spring of natural length R and spring constant K=(√3+1)mgR. The other end of the spring is fixed at a point A on a smooth vertical ring of radius R as shown in fig. The normal reaction at B just after it is released to move is
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is μ then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View Solution
