Two batteries (connected in series) of same emf 10 V of internal resistances 20ohm and 5ohm are connected to a load resistance of 30ohm. Now an unknown resistance x is connected in parallel to the load resistance. Find value of x so that potential drop of battery having internal resistance 20ohm becomes zero.
The correct Answer is:30
To solve the problem, we need to find the value of the unknown resistance x that, when connected in parallel to a load resistance of 30Ω, causes the potential drop across the battery with an internal resistance of 20Ω to become zero.
1. Understanding the Circuit:
We have two batteries connected in series, each with an emf of 10V. The internal resistances are R1=20Ω and R2=5Ω. A load resistance RL=30Ω is connected, and we need to find the resistance x that is connected in parallel to RL.
2. Condition for Zero Potential Drop:
For the potential drop across the battery with internal resistance R1 (i.e., 20Ω) to be zero, the voltage across it must equal its emf:
V1=E1−I⋅R1=0
where E1=10V and R1=20Ω.
3. Finding the Current I:
Rearranging the equation gives:
I⋅R1=E1⟹I=E1R1=10V20Ω=0.5A
4. Calculating Voltage Across the Second Battery:
Now, we can find the voltage drop across the second battery with internal resistance R2=5Ω:
V2=E2−I⋅R2=10V−0.5A⋅5Ω=10V−2.5V=7.5V
5. Applying Kirchhoff's Current Law:
Let I1 be the current through the load resistance RL and I2 be the current through the unknown resistance x. According to Kirchhoff's current law:
I=I1+I2
where I=0.5A.
6. Finding Currents Through RL and x:
The current through the load resistance RL is given by:
I1=V2RL=7.5V30Ω=0.25A
The current through the unknown resistance x is:
I2=I−I1=0.5A−0.25A=0.25A
7. Finding the Value of x:
Using Ohm's law for the current through x:
I2=V2x⟹0.25A=7.5Vx
Rearranging gives:
x=7.5V0.25A=30Ω
Final Answer:
The value of the unknown resistance x is 30Ω.
We have two batteries connected in series, each with an emf of 10V. The internal resistances are R1=20Ω and R2=5Ω. A load resistance RL=30Ω is connected, and we need to find the resistance x that is connected in parallel to RL.
For the potential drop across the battery with internal resistance R1 (i.e., 20Ω) to be zero, the voltage across it must equal its emf:
V1=E1−I⋅R1=0
where E1=10V and R1=20Ω.
Rearranging the equation gives:
I⋅R1=E1⟹I=E1R1=10V20Ω=0.5A
Now, we can find the voltage drop across the second battery with internal resistance R2=5Ω:
V2=E2−I⋅R2=10V−0.5A⋅5Ω=10V−2.5V=7.5V
Let I1 be the current through the load resistance RL and I2 be the current through the unknown resistance x. According to Kirchhoff's current law:
I=I1+I2
where I=0.5A.
The current through the load resistance RL is given by:
I1=V2RL=7.5V30Ω=0.25A
The current through the unknown resistance x is:
I2=I−I1=0.5A−0.25A=0.25A
Using Ohm's law for the current through x:
I2=V2x⟹0.25A=7.5Vx
Rearranging gives:
x=7.5V0.25A=30Ω
The value of the unknown resistance x is 30Ω.
Topper's Solved these Questions
Similar Questions
24 cells of emf 1.5V each having internal resistance of 1 ohm are connected to an external resistance of 1.5 ohms. To get maximum current,
View SolutionThe resultant resistance value of n resistance each of r ohms and connected is series is X. When those n , resistance are connected in parallel , the resultant values is .
View SolutionA generator has an EMF of 440 V and internal resistance of 400 ohm . Its terminals are connected to a load of 4000 ohm , the voltage across the load is
View SolutionWhen two resistors of (600±3) ohm and (300±6) ohm are connected in parallel, value of the equivalent resistance is
View SolutionThe figure shows two capacitors connected in parallel with two resistance and a battery of emf 10V , internal resistance 5Ω. At steady state.
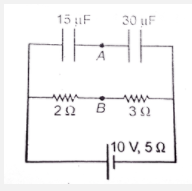
View SolutionA cell of emf ε and internal resistance r is connected across a load resistance R
(i) Find the maximum power delivered at the load ?
.
View SolutionA battery is designed by connecting four cells, each of emf 1.5 V and resistance 2.0 ohm in parallel. If this battery is now connected to an resistance of 2.5 ohm, find
the total resistance of the circuit
View SolutionThe potentiometer wire 10m long and 20 ogm resistance is connected to a 3 volt emf battery and a 10ohm resistance. The value of potential gradient in volt/m of the wire will be
View Solution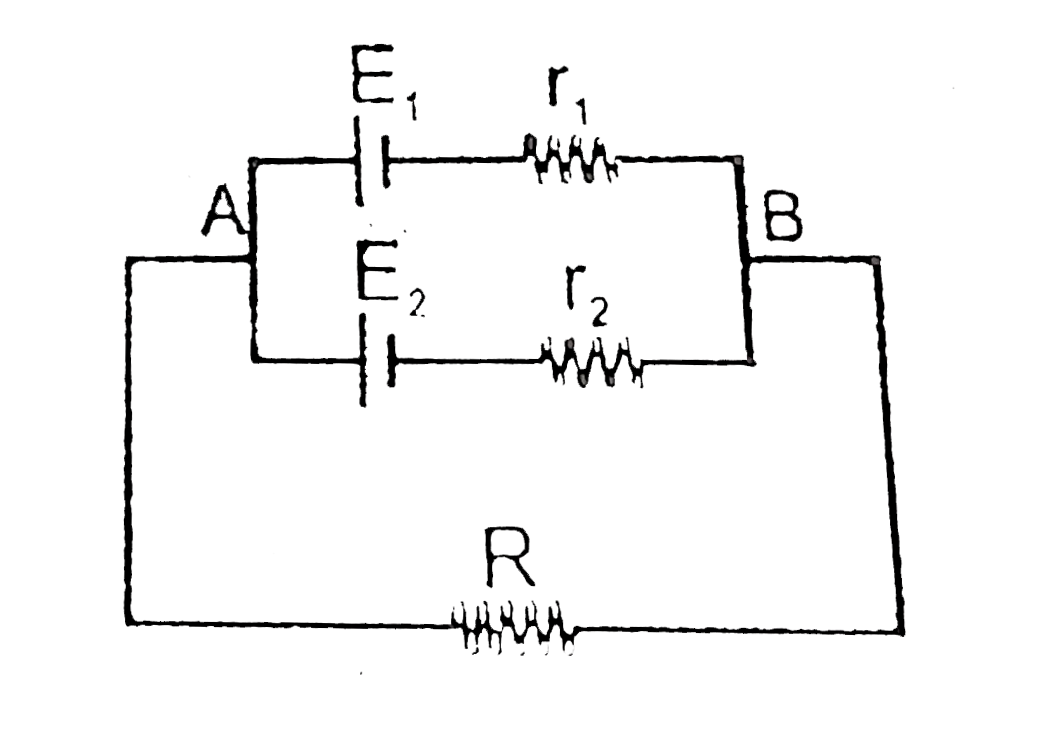
Two batteries of E1 internal resistance r1 & emf E2 internal resistance r2 are connected in parallel and makes a battery system. An external resistance R is connected across this system as shown in the figure.
View SolutionA battery of emf 2V and internal resistance 0.5(Ω)is connected across a resistance 1.5(Ω). Find the current flow through battery ?
View Solution
