Question

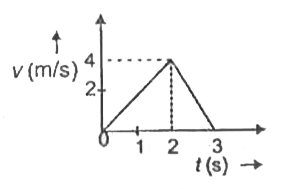
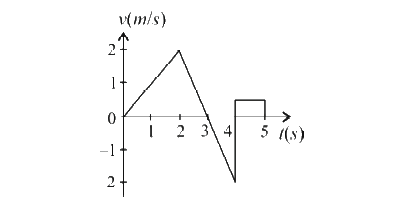
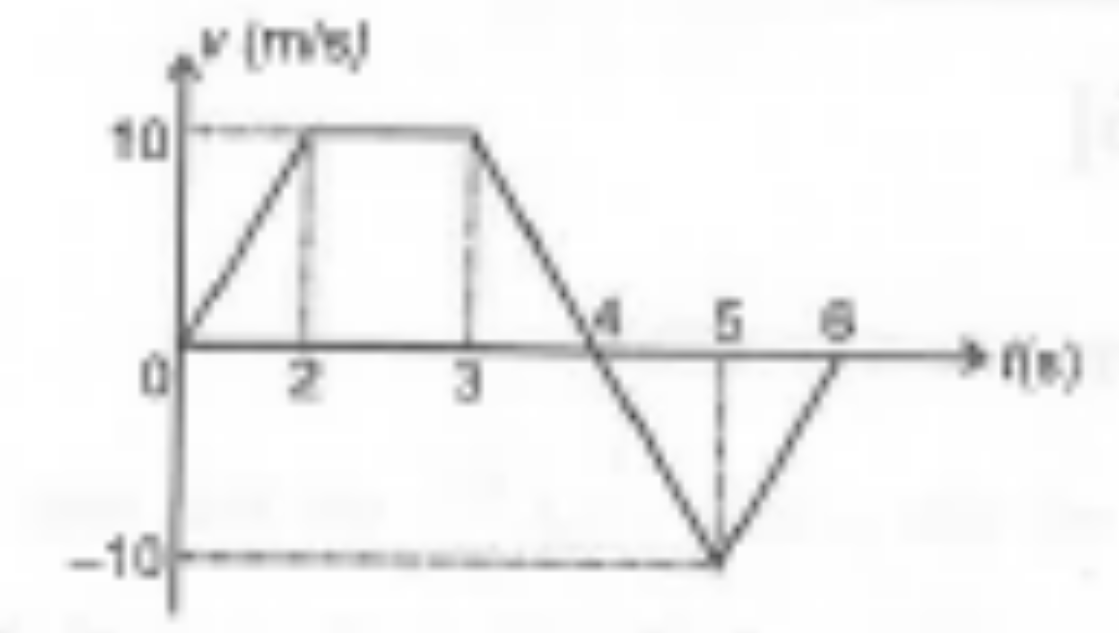
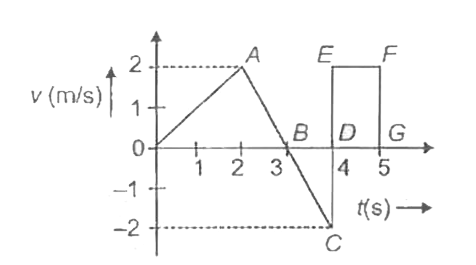
The velocity (v) and time (t) graph of a body in a straight line motion is shown in the figure . The point S is at 4.333 seconds . The total distance covered by the body in 6s is :

Options

A. `(37)/(3) m`
B. 12 m
C. 11m
D. `(49)/(4)m`

Explanation

To solve the problem of finding the total distance covered by the body in 6 seconds using the velocity-time graph, we will follow these steps:

Step 1: Identify the time intervals and the areas under the graph
- The graph shows the velocity of the body at different time intervals. We need to break down the graph into segments to calculate the area under the curve for each segment.
- The point S is at 4.333 seconds, which is approximately 1/3 seconds after 4 seconds.

Step 2: Calculate the area of the trapezium from 0 to 5 seconds
- The trapezium is formed between the time intervals 0 to 4 seconds and 4 to 5 seconds.
- The height of the trapezium is the velocity at these points.
- The area of a trapezium is given by the formula:
\( \text{Area} = \frac{1}{2} \times (b_1 + b_2) \times h \)
where \(b_1\) and \(b_2\) are the lengths of the parallel sides (velocities) and \(h\) is the height (time interval).

Step 3: Calculate the area of the triangle from 5 to 6 seconds
- The triangle is formed between the time intervals 5 to 6 seconds.
- The area of a triangle is given by the formula:
\( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \)
where the base is the time interval and the height is the velocity.

Step 4: Calculate the total distance
- Add the areas of the trapezium and triangle to find the total distance covered.

Detailed Calculation:
1. Area of the trapezium (0 to 5 seconds):
- From the graph, let's assume:
- Velocity at 0 seconds = 0 m/s
- Velocity at 4 seconds = 4 m/s
- Velocity at 5 seconds = 0 m/s
- The area of the trapezium:
\( \text{Area}_{\text{trapezium}} = \frac{1}{2} \times (0 + 4) \times 4 = \frac{1}{2} \times 4 \times 4 = 8 \text{ m} \)

2. Area of the triangle (5 to 6 seconds):
- From the graph, the velocity decreases to -2 m/s at 6 seconds.
- The base of the triangle = 1 second (from 5 to 6 seconds)
- The height = 2 m/s (magnitude of -2 m/s)
- The area of the triangle:
\( \text{Area}_{\text{triangle}} = \frac{1}{2} \times 1 \times 2 = 1 \text{ m} \)

3. Total distance covered:
\( \text{Total Distance} = \text{Area}_{\text{trapezium}} + \text{Area}_{\text{triangle}} = 8 + 1 = 9 \text{ m} \)

Final Answer:
The total distance covered by the body in 6 seconds is 9 meters.