A cylindrical vessel of uniform cross-section contains liquid upto the height' H’ . At a depth $H = \frac{H}{2}$ below the free surface of the liquid there is an orifice. Using Bernoulli's theorem, find the velocity of efflux of liquid.

Video Solution

Text Solution

Generated By DoubtnutGPT

To find the velocity of efflux of liquid from an orifice located at a depth $H / 2$ below the free surface of the liquid in a cylindrical vessel, we will use Bernoulli's theorem. Here’s a step-by-step solution:
Step 1: Identify the points
We will consider two points for applying Bernoulli's equation:
- Point 1: At the free surface of the liquid.
- Point 2: Just outside the orifice at depth $H / 2$ .
Step 2: Write Bernoulli's equation
According to Bernoulli's theorem, the equation can be expressed as:
$P_{1} + \frac{1}{2} ρ V_{1}^{2} + ρ g z_{1} = P_{2} + \frac{1}{2} ρ V_{2}^{2} + ρ g z_{2}$
where:
- $P$ is the pressure,
- $ρ$ is the density of the liquid,
- $V$ is the velocity of the liquid,
- $g$ is the acceleration due to gravity,
- $z$ is the height above a reference level.
Step 3: Define the parameters at Point 1
At Point 1 (free surface):
- The pressure $P_{1} = P_{atm}$ (atmospheric pressure),
- The velocity $V_{1} \approx 0$ (since the area of the surface is much larger than the orifice),
- The height $z_{1} = 0$ (taking the free surface as the reference level).
Step 4: Define the parameters at Point 2
At Point 2 (just outside the orifice):
- The pressure $P_{2} = P_{atm}$ (also atmospheric pressure),
- The velocity $V_{2}$ is what we want to find,
- The height $z_{2} = - \frac{H}{2}$ (since it is below the reference level).
Step 5: Substitute values into Bernoulli's equation
Substituting the values into the Bernoulli equation:
$P_{atm} + \frac{1}{2} ρ (0)^{2} + ρ g (0) = P_{atm} + \frac{1}{2} ρ V_{2}^{2} + ρ g (- \frac{H}{2})$
Step 6: Simplify the equation
Cancel $P_{atm}$ from both sides:
$0 = \frac{1}{2} ρ V_{2}^{2} - \frac{1}{2} ρ g H$
Step 7: Rearrange to solve for $V_{2}$
Rearranging gives:
$\frac{1}{2} ρ V_{2}^{2} = \frac{1}{2} ρ g H$
Dividing both sides by $\frac{1}{2} ρ$ :
$V_{2}^{2} = g H$
Step 8: Take the square root
Taking the square root of both sides gives:
$V_{2} = \sqrt{g H}$
Conclusion
The velocity of efflux of the liquid from the orifice is:
$V_{2} = \sqrt{g H}$

Updated on:7/8/2024

Two vessels A and B of cross sections as shown contain a liquid up to the same height. As the temperature rises, the liquid pressure at the bottom (neglecting expansion of the vessels ) will :

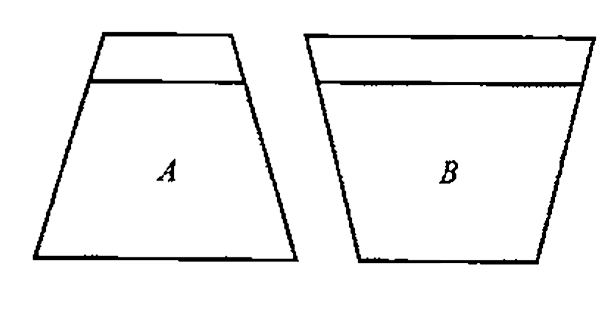AIncrease in A , decrease in B
Bincrease in B , decrease in A
CIncrease in both A and B but more in A
DIncrease in both A and B equally
A cylindrical vessel of cross section A contains water to a height h. There is a hole in the bottom of radius 'a'. The time in which it will be emptied is:
A $\frac{2 A}{π a^{2}} \sqrt{\frac{h}{g}}$
B $\frac{\sqrt{2} A}{π a^{2}} \sqrt{\frac{h}{g}}$
C $\frac{2 \sqrt{2} A}{π a^{2}} \sqrt{\frac{h}{g}}$
D $\frac{A}{\sqrt{2} π a^{2}} \sqrt{\frac{h}{g}}$
A liquid is filled in a vessel and a hole is made at a depth h below the free surface of the liquid.
Statement-1 : Greater is the distance of the hole form the free surface of liquid, greater will be the velocity of efflux.
And
Statement-2 The speed of the liquid coming out of the orifice depends on the quantity of liquid in the vessel.
AStatement-1 is True, Statement-2 is True, Statement-2 is a correct explanation for Statement-1
BStatement-1 is True, Statement-2 is True, Statement-2 is not a correct explanation for Statement-1
CStatement-1 is True, Statement-2 is False
DStatement-1 is False, Statement-2 is True