Question

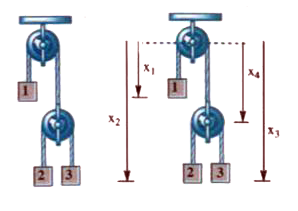
Find the constraint relation between $a_1,a_2\text{and}{a}_3$.

Explanation

Points 1,2,3 and 4 are movable. Let their dis-placements from a fixed line be ` x_1 , x_2 , x_3 and x_4`
We have ` x_1 + x_4 = l_1` ( length of first string ) ..... (i)
and `(x_2 - x_4)+ (x_3 - x_4) = l_2` (length of second string )
or ` x_2 + x_3 - 2x_4 = l_2`............ (ii)
On double ,differentiating with respect to time ,
we get ` a_1 + a_4 =0` ............ (iii)
and ` a_2 + a_3 - 2a_4 = 0` ......... (iv)
But since ` a_4 = -a_1 a_4 = - a_1 ` [From equation (iii) ]
we have ,` a_2 + a_3 + 2a_1 =0`
This is the required constraint relation between ` a_1 , a_2 and a_3`