In the young's double slit experiment, the intensities at two points P(1) and P2 on the screen are respectively I1 and I2. If P1 is located at the centre of a bright fringe and P2 is located at a distance equal to a quarter of fringe width from P1, then I1I2 isSolution in Telugu
2
1/2
4
16
The correct Answer is:A
Step by step video, text & image solution for In the young's double slit experiment, the intensities at two points P(1) and P_(2) on the screen are respectively I_(1) and I_(2). If P_(1) is located at the centre of a bright fringe and P_(2) is located at a distance equal to a quarter of fringe width from P_(1), then I_(1)/I_(2) is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PRACTICE SHEET EXERCISE -I (LEVEL II - ADVANCED)(Straight Objective Type Questions)9videos
WAVE OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PRACTICE SHEET EXERCISE -I (LEVEL II - ADVANCED)(More than one correct answer Type Questions)2videos
WAVE OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - LECTURE SHEET EXERCISE-III (LEVEL -II) (MAIN) (Straight Objective Type Questions)8videos
WAVE OPTICS
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - PROBLEMS (LEVEL - II)81videos
WAVE MOTION AND SOUND
- BOOK - AAKASH SERIESCHAPTER - WAVESEXERCISE - EXERCISE-III (Doppler effect :)15videos
WAVES
Similar Questions
A beam of light consisting of two wavelength , 6500A∘ and 5200A∘ is used to obtain interference fringes in a Young's double slit experiment (1Å=10−10m). The distance between the slits 2.0 mm and the distance between the plane of the slits and the screen is 120 cm. Find the distance of the third bright fringe on the screen from the central maximum for the wavelength 6500Å.
04:06View SolutionThe figure shows two points source which emit light of wavelength λ in phase with each other and are at a distance d=5.5λ apart along a line which is perpendicular to a large screen at a distance L from the centre of the source. Assume that d is much less than L. Which of the following statement is (are) correct ?
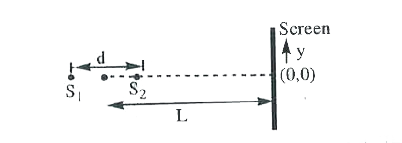 View Solution
View SolutionUnpolarised light of intensity 32 Wm−2 passes through three polarisers such that the transmission axis of the last polariser s crossed with first . If the intensity of the emerging light is 32Wm−2 , the angle between the axes of the first two polarisers is
02:30View SolutionA wave is described by the equation
y=(1.0mm)sinπ(x2.0cm−10.01s)
Find the time period and the wavelength.03:46View SolutionWhen a train is approaching the stationary observer, the apparent frequency of the whistle observed as 100 Hz, while when it has passed away from the observer with same speed, it is 50 Hz. Calculate the frequency of the whistle when the observer moves with the train (V = 330 m/s)
05:17View SolutionUnpolarized light is intensity I0 passes through two polaroids P1 and P2 such that pass axis of P2 makes an angle θ with the pass axis of P1. A third polaroid P3 is placed between P1 and P2 with pass axis of P3 making an angle β with that of P1. If I1,I2,I3 represent intensities of light trnasmitted by P1,P2,P3, determine the values of angle θ and β for which I1=I2=I3.
08:19View SolutionThe polaroids P1 and P2 are placed in crossed position. A third polaroid P3 is kept between P1 and P2 such that pass axis of P3 is parallel to that of P1. How would the intensity of light (I2) transmitted through P2 vary as P3 is rotated ? Draw plot of intensity I2 versus angle θ between pass axis of P1 and P3. In which orientation will the transmitted intensity be minimum and maximum ?
03:44View SolutionTwo Polaroids P1 and P2 are placed with their axis perpendicular to eachother. Unpolarised light I0 is nicident on P1. A third polaroid P3 is kept in between P1 and P2 such that its axis makes an angle 45∘ with that of P1. The intensity of transmitted light through P2 is
03:16View SolutionA light source is located at P1 as shown in the figure. All sides of the polygon are equal. The intensity of illumination at P2 is I0 . What will be the intensity of illumination at P3
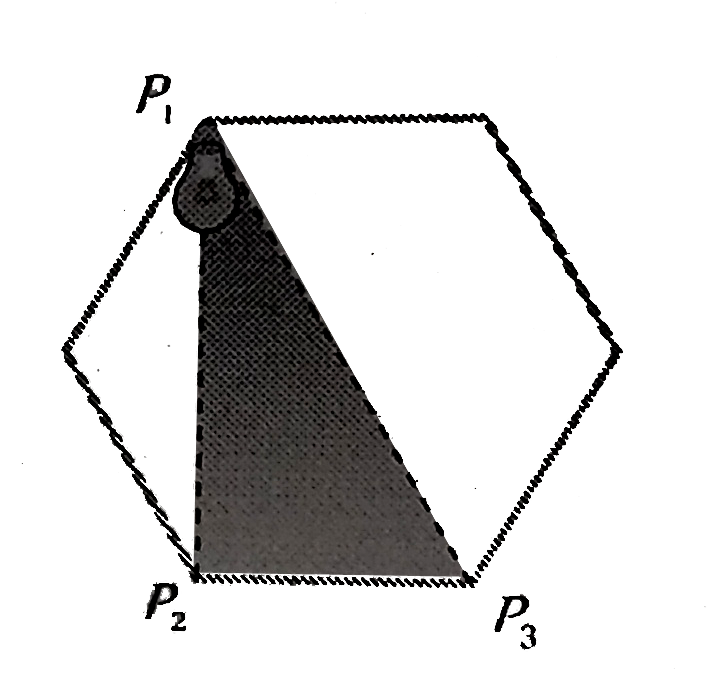 View Solution
View SolutionIn the Young's double slit experiment, the intensities at two points P1 and P2 on the screen are respectively I1 and I2 If P1 is located at the centre of a bright fringe and P2 is located at a distance equal to a quarter of fringe width from P1 then I1I2 is
View SolutionThe intensity of each of the two slits in Young's double slit experiment is I_(0) Calculate the minimum separation between the two points on the screen where intensities are 2I_(0) and I_(0) Given the fringe width equal to alpha
03:35View SolutionIn the young's double slit experiment, the intensities at two points P(1) and P_(2) on the screen are respectively I_(1) and I_(2) . If P_(1) is located at the centre of a bright fringe and P_(2) is located at a distance equal to a quarter of fringe width from P_(1) , then I_(1)/I_(2) is
04:30View SolutionIn Young's double slit experiment of central fringe is I0 and fringe width is β. If a point is at a distance x from the central fringe, then the intensity at that point is
06:19View SolutionState clearly how an unpolarised light gets linearly polarised when passed through a polaroid.
(i) Unpolarised light of intensity I0 is incident on a polaroid P1 which is kept near another polaroid P2 whose pass axis is parallel to that of P1. how will the intensities of light, I1 and I2, transmitted by the polaroids P1andP2 respectively, change on rotating P1 without disturbing P2 ?
(ii) Write the relation between the intensities I1andI2.03:06View Solution(a) Unpolarised light of intensity I0 passes through two polaroids P1 and P2 such that pass axis of P2 makes an angle θ with the pass axis of P1, Plot a graph showing the variation of intensity of light transmitted through P2 as the angle θ varies from zero to 180∘.
(b) A third polariod P3 is placed between P1 and P2 with pass axis of P3 making an angle β with that of P1. If I1, I2 and I3 represent the intensities of light transmitted by P1, P2 and P3 determine the values of angle θ and β for which I1=I2=I3.03:01View Solution

