Find out the location of centre of mass of a uniform rod.

Suppose a rod of mass M and length L is lying along the x-axis with its one end at x=0 and the other at x = L
Mass per unit length of the rod =ML
Hence, mass of the element PQ of length dx situated at a distance V from the origin is dm =ML dx
The coordinates of the element PQ are (x, 0,0). Therefore, x -coordinate of CM of the rod will be:
xCM=∫L0xdm∫dm=∫L0(x)(MLdx)M=IL∫L0xdx=L2,ycm=∫ydm∫dm=0
Similar Questions
Find the location of centre of mass of a uniform semicirular plate of radius R and mass M.
View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.
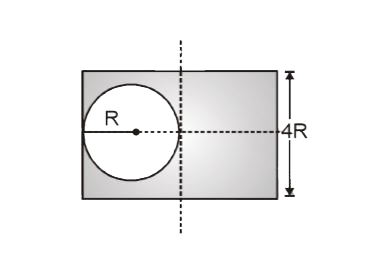
View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.from center of cavity

View SolutionWhat is the location of the centre of mass of a uniform triangular lamina ?
View SolutionLocate the position of centre of mass of a uniform plate of Mass m as shown in fig 4E 34

View SolutionCentre Of Mass Of Uniform Rod
View SolutionFind the centre of mass of a uniform solid cone.
View SolutionFind the centre of mass of a uniform solid cone.
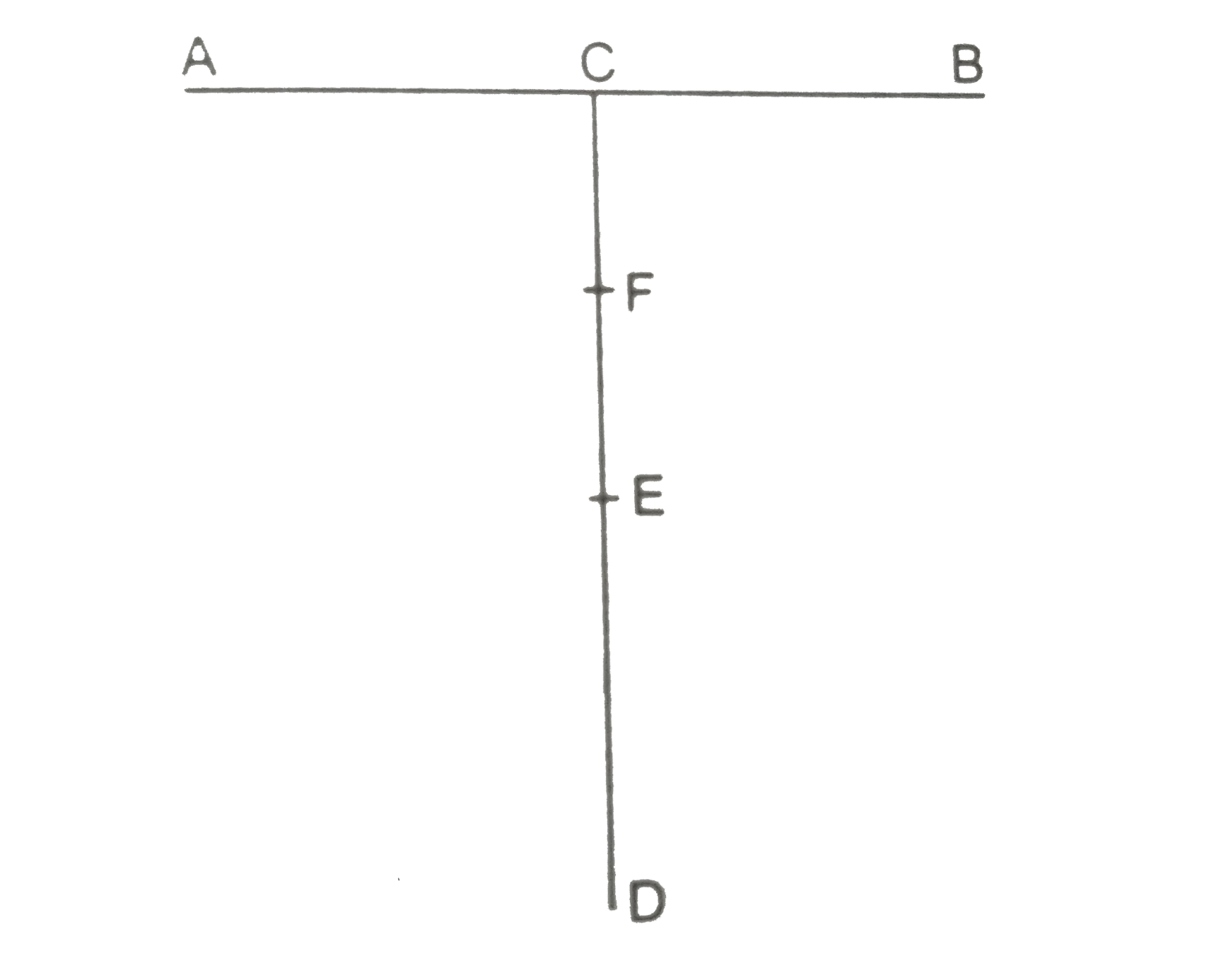
View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
View SolutionThe linear mass density lambda of a rod AB is given by λ=aplha+βxkgm taking O as origin. Find the location of the centre of mass from the end A?
View Solution
