Two metal wires of identical dimesnios are connected in series. If σ1 and σ2 are the conducties of the metal wires respectively, the effective conductivity of the combination is
σ1+σ2
σ1+σ22
√σ1+σ2
2σ1+σ2σ1+σ2
The correct Answer is:D
To find the effective conductivity of two metal wires of identical dimensions connected in series, we will follow these steps:
Step 1: Understand the relationship between conductivity and resistivity
Conductivity (σ) is the reciprocal of resistivity (ρ). Therefore, we have:
σ=1ρ
This means that resistivity can be expressed as:
ρ=1σ
Step 2: Write the formula for resistance
The resistance (R) of a wire can be expressed in terms of its resistivity, length (L), and cross-sectional area (A):
R=ρLA
Substituting the expression for resistivity, we have:
R=LσA
Step 3: Calculate the individual resistances
For two wires with conductivities σ₁ and σ₂, the resistances R₁ and R₂ can be written as:
R1=Lσ1A
R2=Lσ2A
Step 4: Find the effective resistance
Since the two wires are connected in series, the total or effective resistance (R_effective) is the sum of the individual resistances:
Reffective=R1+R2=Lσ1A+Lσ2A
Step 5: Simplify the effective resistance expression
Factoring out common terms, we get:
Reffective=LA(1σ1+1σ2)
Step 6: Relate effective resistance to effective conductivity
Let the effective conductivity be σ_effective. We can express the effective resistance in terms of effective conductivity:
Reffective=LσeffectiveA
Setting the two expressions for R_effective equal to each other:
LσeffectiveA=LA(1σ1+1σ2)
Step 7: Cancel common terms and solve for effective conductivity
Canceling L and A from both sides gives:
1σeffective=1σ1+1σ2
Taking the reciprocal of both sides, we find:
σeffective=2σ1σ2σ1+σ2
Final Result
Thus, the effective conductivity of the combination of the two metal wires is:
σeffective=2σ1σ2σ1+σ2
---
Conductivity (σ) is the reciprocal of resistivity (ρ). Therefore, we have:
σ=1ρ
This means that resistivity can be expressed as:
ρ=1σ
The resistance (R) of a wire can be expressed in terms of its resistivity, length (L), and cross-sectional area (A):
R=ρLA
Substituting the expression for resistivity, we have:
R=LσA
For two wires with conductivities σ₁ and σ₂, the resistances R₁ and R₂ can be written as:
R1=Lσ1A
R2=Lσ2A
Since the two wires are connected in series, the total or effective resistance (R_effective) is the sum of the individual resistances:
Reffective=R1+R2=Lσ1A+Lσ2A
Factoring out common terms, we get:
Reffective=LA(1σ1+1σ2)
Let the effective conductivity be σ_effective. We can express the effective resistance in terms of effective conductivity:
Reffective=LσeffectiveA
Setting the two expressions for R_effective equal to each other:
LσeffectiveA=LA(1σ1+1σ2)
Canceling L and A from both sides gives:
1σeffective=1σ1+1σ2
Taking the reciprocal of both sides, we find:
σeffective=2σ1σ2σ1+σ2
Thus, the effective conductivity of the combination of the two metal wires is:
σeffective=2σ1σ2σ1+σ2
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - CELLS, EMF, INTERNAL RESISTANCE
6 Videos
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - KIRCHOFF S LAW
14 Videos
- CURRENT ELECTRICITYBOOK - NCERT FINGERTPS ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ELECTRICAL ENERGY, POWER
8 Videos
- COMMUNITCATION SYSTEMSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - COMMUNITCATION SYSTEMSEXERCISE - Assertion And Reason
30 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - Assertion And Reason
15 Videos
Similar Questions
Knowledge Check
A metal wire of length L1 and area of cross section A is attached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
A[(Y1Y2)A][2(Y1L2+Y2L1)]B[(Y1Y2)A](L1L2)2C[(Y1Y2)A](Y1L2+Y2L1)D[(Y1Y2)1/2A](L2+L1)1/2A metal wire of length L1 and area of cross section A is ttached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
AY1Y2A2(Y1L2+Y2L1)BY1Y2A(L1L2)1/2CY1Y2A(Y1L2+Y2L1)D(Y1Y2)1/2A(L1L2)1/2
Electrical conductivies of Ge and Na are σ1 and σ2 respectively. If these substances are heard, then
View SolutionTwo teams A and B have the same mean and their coefficients of variance are 4, 2 respectively. If σA,σB are the standard deviations of teams A, B respectively then the relation between item is
View SolutionTwo wires of same dimension but resistivity p1 and p2 are connected in series. The equivalent resistivity of the combination is
View SolutionTwo wires of the same dimensions but resistivities ρ1 and ρ2 are connected in series. The equivalent resistivity of the combination is
View SolutionTwo wires of the same dimensions but resistivities p1 and p2 are connected in series. The equivalent resistivity of the combination is
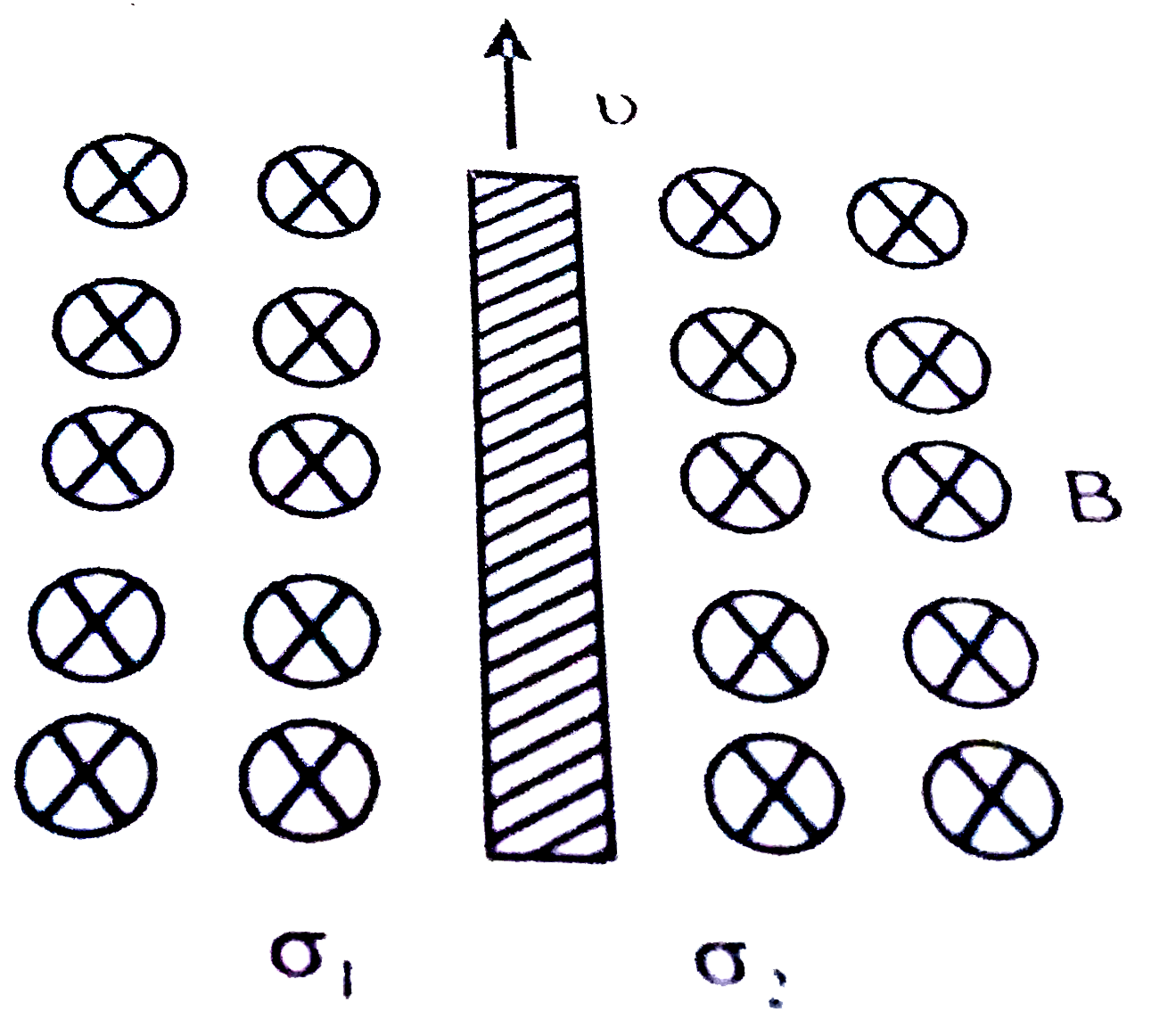
View SolutionConsider a thin metallic sheet perpendicular to the plane of the paper moving with speed υ in a uniform magnetic field B going into the plane of the paper (See figure.) If charge densities σ1andσ2 are induced on the left and right surfaces, respectively, of the sheet then (ignore fringe effects.)
View Solution

