Two wires of same dimension but resistivity and are connected in series. The equivalent resistivity of the combination is
The correct Answer is:B
To find the equivalent resistivity of two wires of the same dimension but different resistivities p1 and p2 connected in series, we can follow these steps:
1. Identify the Resistances:
- Let the resistances of the two wires be R1 and R2.
- The resistivity of the first wire is p1 and the second wire is p2.
- Both wires have the same length L and the same cross-sectional area A.
2. Calculate Individual Resistances:
- The resistance of the first wire can be calculated using the formula:
R1=p1⋅LA
- Similarly, the resistance of the second wire is:
R2=p2⋅LA
3. Calculate Total Resistance in Series:
- When resistors are connected in series, the total resistance R is the sum of the individual resistances:
R=R1+R2=p1⋅LA+p2⋅LA
- This can be simplified to:
R=(p1+p2)⋅LA
4. Express Total Resistance in Terms of Equivalent Resistivity:
- We can express the total resistance R in terms of an equivalent resistivity P:
R=P⋅(2L)A
- Here, 2L is the combined length of the two wires when treated as a single wire.
5. Equate the Two Expressions for Resistance:
- Now, we can set the two expressions for R equal to each other:
(p1+p2)⋅LA=P⋅(2L)A
6. Cancel Common Terms:
- Since L and A are common in both sides, we can cancel them out:
p1+p2=2P
7. Solve for Equivalent Resistivity P:
- Rearranging the equation gives us:
P=p1+p22
Final Answer:
The equivalent resistivity of the combination is:
P=p1+p22
- Let the resistances of the two wires be R1 and R2.
- The resistivity of the first wire is p1 and the second wire is p2.
- Both wires have the same length L and the same cross-sectional area A.
- The resistance of the first wire can be calculated using the formula:
R1=p1⋅LA
- Similarly, the resistance of the second wire is:
R2=p2⋅LA
- When resistors are connected in series, the total resistance R is the sum of the individual resistances:
R=R1+R2=p1⋅LA+p2⋅LA
- This can be simplified to:
R=(p1+p2)⋅LA
- We can express the total resistance R in terms of an equivalent resistivity P:
R=P⋅(2L)A
- Here, 2L is the combined length of the two wires when treated as a single wire.
- Now, we can set the two expressions for R equal to each other:
(p1+p2)⋅LA=P⋅(2L)A
- Since L and A are common in both sides, we can cancel them out:
p1+p2=2P
- Rearranging the equation gives us:
P=p1+p22
The equivalent resistivity of the combination is:
P=p1+p22
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two resistors of resistances R1=(300±3)Ω and R2=(500±4)Ω are connected in series. The equivalent resistance of the series combination is
A(800±1)ΩB(800±7)ΩC(200±7)ΩD(200±1)Ω
Two wires of the same dimensions but resistivities ρ1 and ρ2 are connected in series. The equivalent resistivity of the combination is
View SolutionThe wires is of same dimension but resistivities ρ1 and ρ2 are connected in parallel . The equivalent resistivity of the combination is
View SolutionThe equivalent resistance of the combination across AB is .
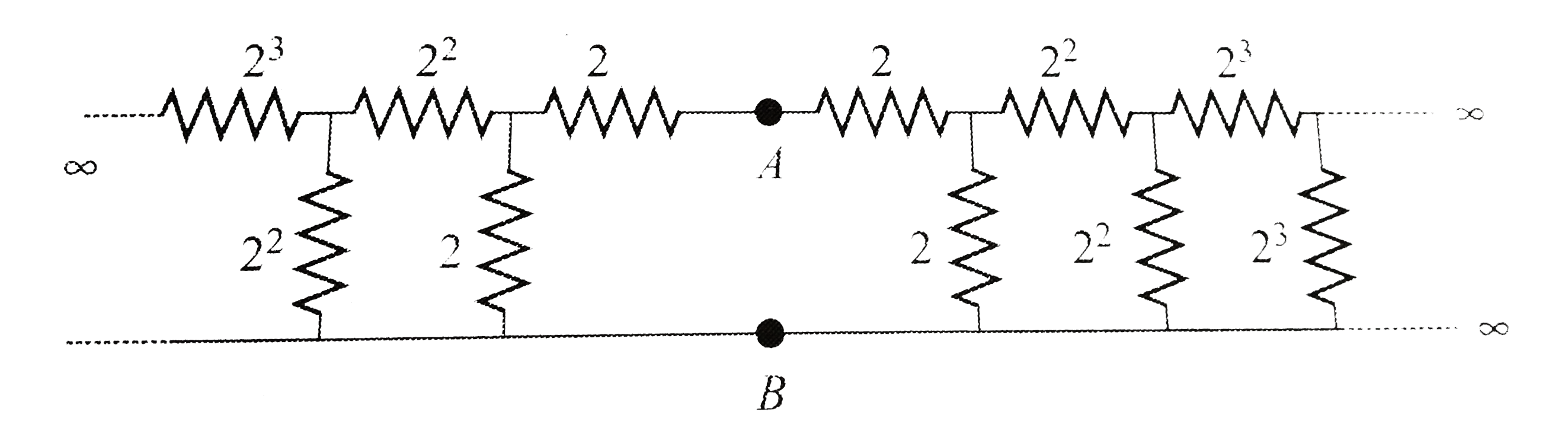
View SolutionTwo resistors of 2.092 and 3.0 22 are connected
in series. Find the effective resistance of combination .
View SolutionA wire of resistance R is cut into ‘ n ’ equal parts. These parts are then connected in parallel. The equivalent resistance of the combination will be
View SolutionA uniform wire of resistance r is cut into n parts and they are connected in parallel. Effective resistance of the combination is
View SolutionThe resistance 4R,16R,64R....∞ are connected in series. Find their equivalent resistance.
View Solution
