A series LCR circuit with R=20Ω,L=1.5H and C=35μF is connected to a variable frequency 200V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power in kW transferred to the circuit in one complete cycle?
200 W
2000 W
100 W
4000 W
The correct Answer is:B
To find the average power transferred to the circuit in one complete cycle when the frequency of the supply equals the natural frequency of the circuit, we can follow these steps:
Step 1: Identify the given parameters
- Resistance, R=20Ω
- Inductance, L=1.5H
- Capacitance, C=35μF=35×10−6F
- Voltage, V=200V
Step 2: Calculate the natural frequency of the circuit
The natural frequency f0 of an LCR circuit is given by the formula:
f0=12π√LC
Substituting the values of L and C:
f0=12π√1.5×35×10−6
Step 3: Calculate the impedance at resonance
When the frequency of the supply equals the natural frequency of the circuit, the impedance Z of the circuit is equal to the resistance R:
Z=R=20Ω
Step 4: Calculate the average power
The average power P in an AC circuit is given by the formula:
P=V2rmsR
Here, Vrms=200V. Thus,
P=(200)220
Calculating this gives:
P=4000020=2000W
Step 5: Convert power to kilowatts
To convert watts to kilowatts, we divide by 1000:
P=20001000=2kW
Final Answer
The average power transferred to the circuit in one complete cycle is 2kW.
---
- Resistance, R=20Ω
- Inductance, L=1.5H
- Capacitance, C=35μF=35×10−6F
- Voltage, V=200V
The natural frequency f0 of an LCR circuit is given by the formula:
f0=12π√LC
Substituting the values of L and C:
f0=12π√1.5×35×10−6
When the frequency of the supply equals the natural frequency of the circuit, the impedance Z of the circuit is equal to the resistance R:
Z=R=20Ω
The average power P in an AC circuit is given by the formula:
P=V2rmsR
Here, Vrms=200V. Thus,
P=(200)220
Calculating this gives:
P=4000020=2000W
To convert watts to kilowatts, we divide by 1000:
P=20001000=2kW
The average power transferred to the circuit in one complete cycle is 2kW.
Topper's Solved these Questions
Similar Questions
A series L-C-R circuit with R = 20Ω, L = 1.5 H and C =35μF is connected to a variable frequency 200 V, AC supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transfered to the circuit, what is the average power transfered to the circuit in one complete cycle ?
View SolutionA series LCR circuit with L=0.12H,C=480nF, and R=23Ω is connected to a 230V variable frequency supply.
(a) What is the source frequency for which current amplitude is maximum? Find this maximum value.
(b) What is the source frequency for which average power absorbed by the circuit is maximum? Obtain the value of maximum power.
(c ) For which frequencies of the source is the power transferred to the circuit half the power at resonant frequency?
(d) What is the Q-factor of the circuit?View Solution
Knowledge Check
- Question 1 - Select One
A series LCR circuit with R=22Ω,L=1.5H and C=40μF is connected to a variable frequency 220 V ac supply. When the frequency of the supply equals the natural frequency of the circuit, what is the average power transferred to the circuit in one complete cycle?
A2000 WB2200 WC2400 WD2500 W - Question 2 - Select One
A series LCR circuit has R=5Ω,L=40mH and C=1μF, the bandwidth of the circuit is
A10 HzB20 HzC30 HzD40 Hz - Question 3 - Select One
Figure shows a series LCR circuit connected to a variable frequency 230 V source.
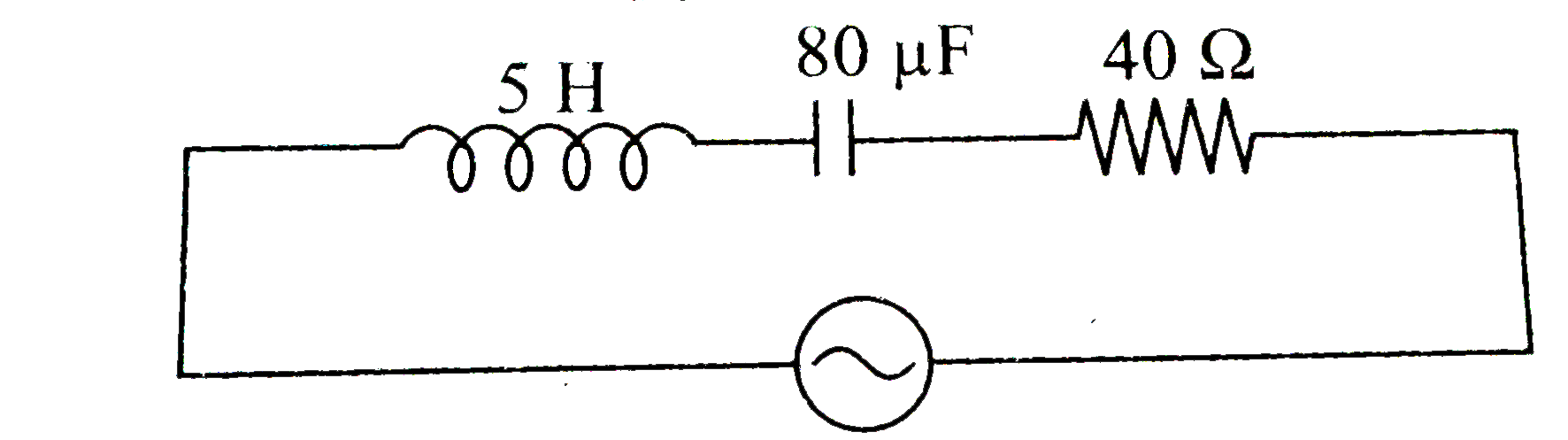
The source frequency which drives the circuit the circuit in resonance isA4 HzB5 HzC6 HzD8 Hz
A series LCR circuit with L=4.0H,C=100μF and R=60Ω is connected to a variable frequency 240 V source. Calculate
(i) angular frequency of the source which drives the circuit in resonance,
(ii) current at the resonating frequency,
(iii) rms potential drop across the inductance at resonance.View SolutionFig. shows series LCR circuit with L=5.0H,C=80μF,R=40Ω connected to a variable frequency 240 V source. Calculate
(i) the angular frequency of the source which drives the circuit at resonance.
(ii) Current at the resonating frequency.
(iii) the rms pot. drop across the capacitor at resonance.
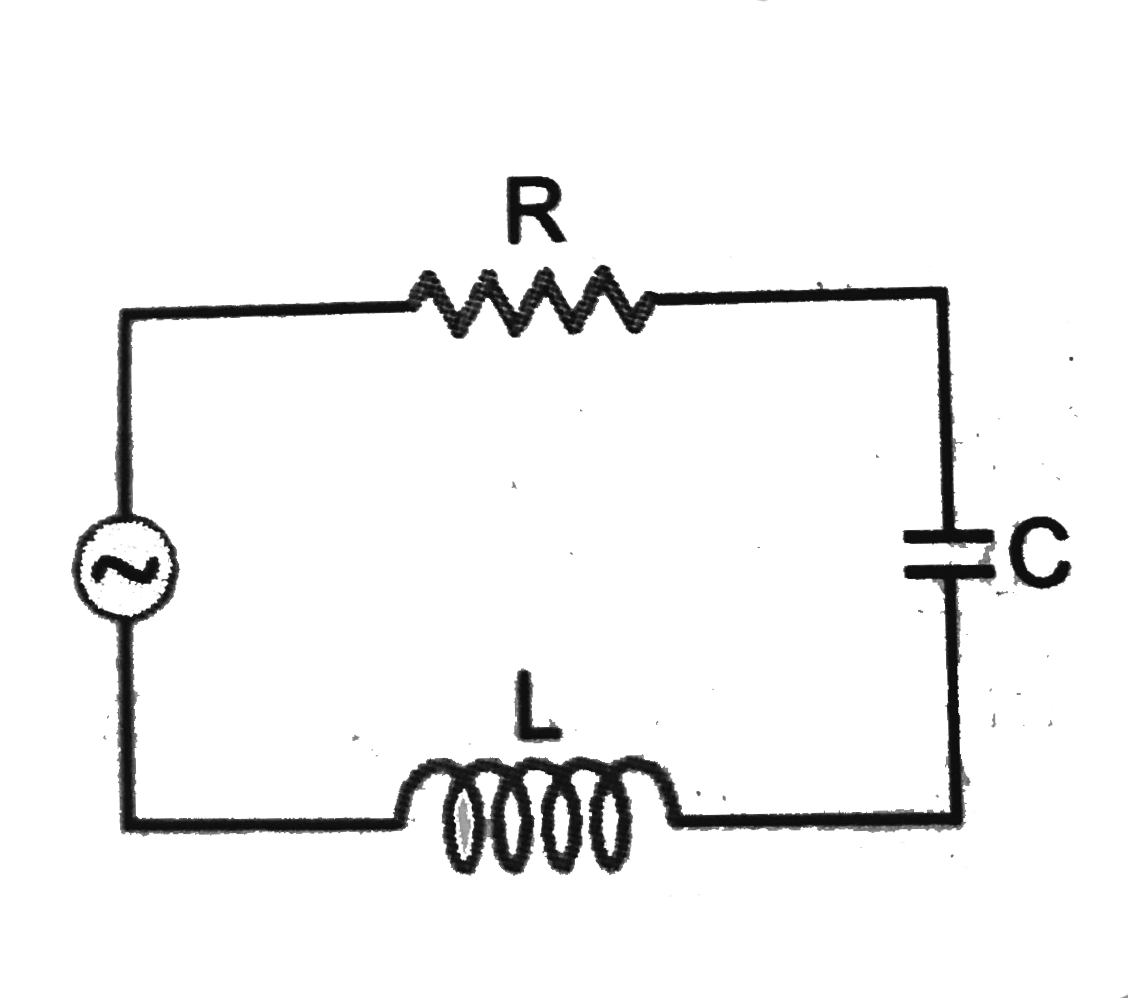 View Solution
View SolutionAn LCR series circuit with L=100mH,C=100μF,R=120Ω is connected to an AC source of emfε=(30V)sin(100s−1)t. Find the impedane, the peak current and the resonant frequency of the circuit.
View SolutionAn inductor L and a capacitor C are connected in the circuit as shown in the figure. The frequency of the power supply is equal to the resonant frequency of the circuit. Which ammeter will read zero ampere
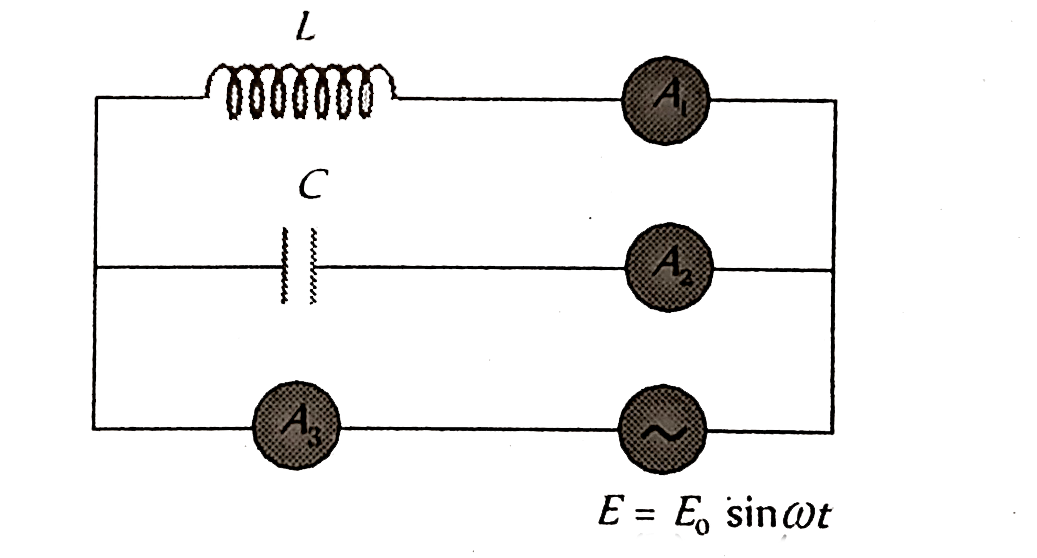 View Solution
View SolutionThe figure shows a series LCR circuit with L=10.0H2,C=40μF,R=60Ω connected to a variable frequency 240 V source. Calculate
(i) The angular frequency of the source which drives the circuit at resonance.
(ii) The current at the resonating frequency.
(iii) The rms potential drop across the capacitor at resonance.
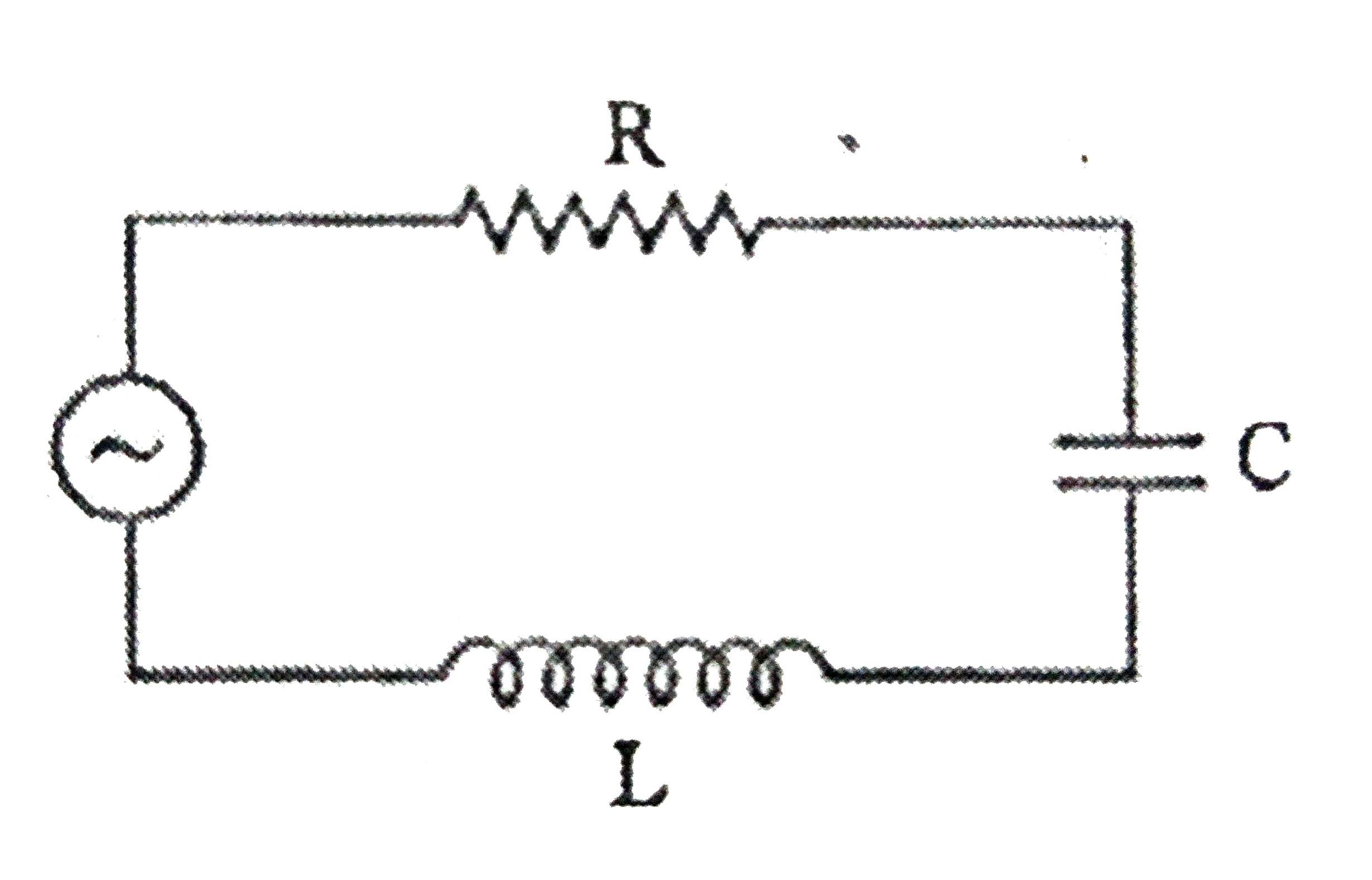 View Solution
View Solution

