A ray of light in incident on a glass plate at an angle of 60∘. What is the refractive index of glass if the reflected and refracted rays are perpendicular to each other?
12
√32
32
√3
The correct Answer is:D
To solve the problem, we need to find the refractive index of glass when a ray of light is incident at an angle of 60∘ and the reflected and refracted rays are perpendicular to each other.
1. Understand the Geometry of the Problem:
- When light hits a surface, it is both reflected and refracted. The angle of incidence (i) is given as 60∘.
- The reflected ray will make the same angle with the normal as the angle of incidence. Therefore, the angle of reflection (r) is also 60∘.
- According to the problem, the reflected ray and the refracted ray are perpendicular to each other. This means that the angle between the reflected ray and the refracted ray is 90∘.
2. Using the Relationship Between Angles:
- If the reflected ray and refracted ray are perpendicular, we can express this relationship mathematically:
r+r′=90∘
where r′ is the angle of refraction. Since r=60∘, we have:
60∘+r′=90∘
Thus,
r′=90∘−60∘=30∘
3. Applying Snell's Law:
- Snell's Law states that:
n1sin(i)=n2sin(r′)
- Here, n1 is the refractive index of air (approximately 1), i is the angle of incidence (60∘), n2 is the refractive index of glass (which we need to find), and r′ is the angle of refraction (30∘).
- Substituting the known values into Snell's Law gives:
1⋅sin(60∘)=n2⋅sin(30∘)
4. Calculating the Sine Values:
- We know:
sin(60∘)=√32andsin(30∘)=12
- Substituting these values into the equation gives:
√32=n2⋅12
5. Solving for the Refractive Index:
- Rearranging the equation to solve for n2:
n2=√3/21/2=√3
6. Final Answer:
- The refractive index of glass is:
n2=√3≈1.732
- When light hits a surface, it is both reflected and refracted. The angle of incidence (i) is given as 60∘.
- The reflected ray will make the same angle with the normal as the angle of incidence. Therefore, the angle of reflection (r) is also 60∘.
- According to the problem, the reflected ray and the refracted ray are perpendicular to each other. This means that the angle between the reflected ray and the refracted ray is 90∘.
- If the reflected ray and refracted ray are perpendicular, we can express this relationship mathematically:
r+r′=90∘
where r′ is the angle of refraction. Since r=60∘, we have:
60∘+r′=90∘
Thus,
r′=90∘−60∘=30∘
- Snell's Law states that:
n1sin(i)=n2sin(r′)
- Here, n1 is the refractive index of air (approximately 1), i is the angle of incidence (60∘), n2 is the refractive index of glass (which we need to find), and r′ is the angle of refraction (30∘).
- Substituting the known values into Snell's Law gives:
1⋅sin(60∘)=n2⋅sin(30∘)
- We know:
sin(60∘)=√32andsin(30∘)=12
- Substituting these values into the equation gives:
√32=n2⋅12
- Rearranging the equation to solve for n2:
n2=√3/21/2=√3
- The refractive index of glass is:
n2=√3≈1.732
Topper's Solved these Questions
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Papper 2
50 Videos
- PRACTICE PAPPERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - PRACTICE PAPPEREXERCISE - Practice Papper 3
50 Videos
- NUCLEIBOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason
15 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - NCERT FINGERTPS ENGLISHCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - NCERT Exemplar
11 Videos
Similar Questions
Unpolarized light is incident on a plane glass surface. What should be the angle of incidence so that the reflected and refracted rays are perpendicular to eachother ?
View SolutionA ray of light is incident on a transparent medium at polarizing angle. What is the angle between the reflected ray and the refracted ray .
View Solution
Knowledge Check
Unpolarized light is incident on a plane glass surface. The angle of incidence so that reflected and refracted rays and perpendicular to each other, then
Ataniβ=μ2Btaniβ=μCsiniβ=μDcosiβ=μLight is incident on a glass surface at polarising angle of 57.5∘ Then the angle between the incident ray and the refracted ray is
A57.5∘B115∘C205∘D145∘
A ray of light is incident on a transparent glass slab of refractive index √3. If the reflected and refracted rays are mutually perpendicular, what is the angle of incidence?

View SolutionUnpolarized light is incident on a plane glass surface having refractive index . The angle of incidence at which reflected and refracted rays would become perpendicular to each other is :
View SolutionStatement I: A light ray is incident on a glass slab. Some portion of it is reflected and some is refracted. Refracted and reflected rays are always perpendicular to each other.
Statement II: Angle of incidence is equal to angle of reflection.
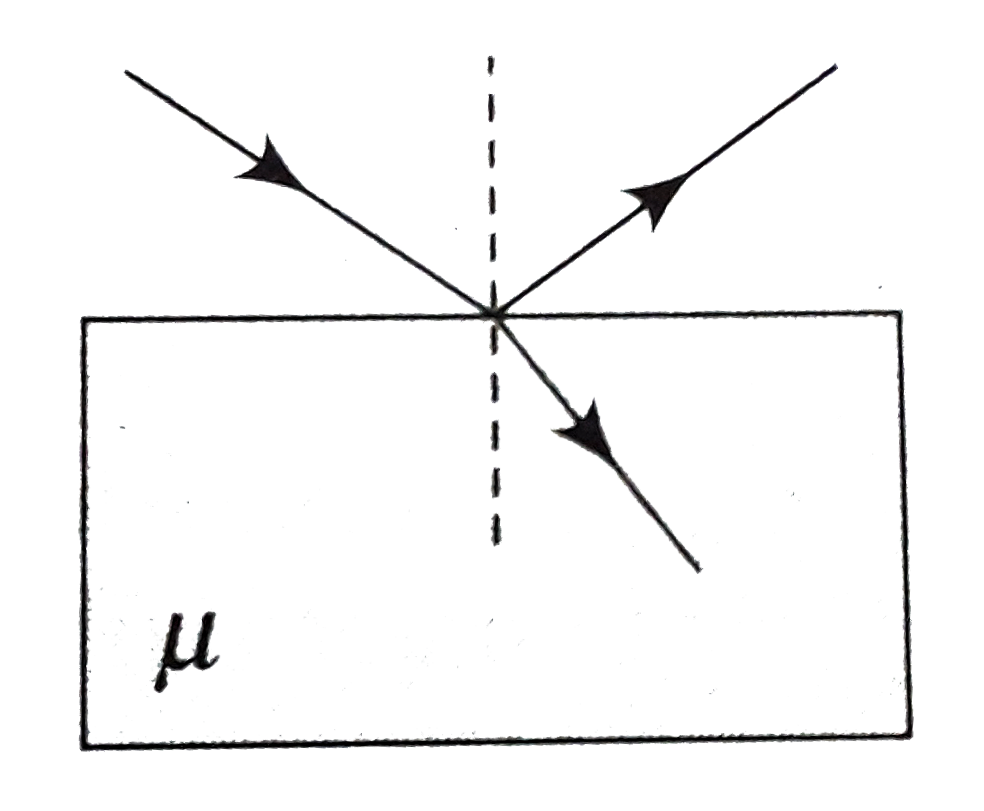
View SolutionA ray of light falls on a transparent glass slab of refractive index 1.62. If the reflected ray and the refracted rays are mutually perpendicular, what is the angle of refraction ?
View SolutionA ray of light falls on a transparent glass slab of refractive index √2. If the reflected and refracted rays are mutually perpendicular, then the angle of incidence is
View SolutionUnpolarised light is incident from air on a plane surface of a material of refractive index μ. At a particular angle of incidence i, it is found that the reflected and refracted rays are perpendicular to each other. Which of the following options is correct for this situation?
View Solution
