A parallel plate air capacitor has a capacitance C. When it is half filled with a dielectric of dielectric constant 5, the percentage increase in the capacitance will be
400%
66.6%
33.3%
200%
The correct Answer is:B
To solve the problem of finding the percentage increase in the capacitance of a parallel plate air capacitor when it is half-filled with a dielectric, we can follow these steps:
1. Understand the Initial Capacitance:
The initial capacitance C of a parallel plate capacitor filled with air (or vacuum) is given by:
C=ε0Ad
where ε0 is the permittivity of free space, A is the area of the plates, and d is the separation between the plates.
2. Identify the Configuration After Filling with Dielectric:
When the capacitor is half-filled with a dielectric of dielectric constant k=5, we can consider the capacitor as two capacitors in series:
- The first capacitor C1 (air part) has a distance of d2.
- The second capacitor C2 (dielectric part) also has a distance of d2 but with a dielectric constant k=5.
3. Calculate the Capacitance of Each Section:
- For the air section:
C1=ε0Ad2=2ε0Ad
- For the dielectric section:
C2=kε0Ad2=5ε0Ad2=10ε0Ad
4. Use the Series Capacitance Formula:
The total capacitance C′ for capacitors in series is given by:
1C′=1C1+1C2
Substituting the values of C1 and C2:
1C′=12ε0Ad+110ε0Ad
This simplifies to:
1C′=d2ε0A+d10ε0A=5d+d10ε0A=6d10ε0A
Therefore:
C′=10ε0A6d=5ε0A3d
5. Calculate the Percentage Increase in Capacitance:
The percentage increase in capacitance can be calculated using the formula:
Percentage Increase=(C′−CC)×100
Substituting C′ and C:
Percentage Increase=⎛⎜⎝5ε0A3d−ε0Adε0Ad⎞⎟⎠×100
Simplifying this gives:
=⎛⎝53−11⎞⎠×100=(23)×100=2003≈66.67%
Final Answer:
The percentage increase in capacitance is approximately 66.67%.
The initial capacitance C of a parallel plate capacitor filled with air (or vacuum) is given by:
C=ε0Ad
where ε0 is the permittivity of free space, A is the area of the plates, and d is the separation between the plates.
When the capacitor is half-filled with a dielectric of dielectric constant k=5, we can consider the capacitor as two capacitors in series:
- The first capacitor C1 (air part) has a distance of d2.
- The second capacitor C2 (dielectric part) also has a distance of d2 but with a dielectric constant k=5.
- For the air section:
C1=ε0Ad2=2ε0Ad
- For the dielectric section:
C2=kε0Ad2=5ε0Ad2=10ε0Ad
The total capacitance C′ for capacitors in series is given by:
1C′=1C1+1C2
Substituting the values of C1 and C2:
1C′=12ε0Ad+110ε0Ad
This simplifies to:
1C′=d2ε0A+d10ε0A=5d+d10ε0A=6d10ε0A
Therefore:
C′=10ε0A6d=5ε0A3d
The percentage increase in capacitance can be calculated using the formula:
Percentage Increase=(C′−CC)×100
Substituting C′ and C:
Percentage Increase=⎛⎜⎝5ε0A3d−ε0Adε0Ad⎞⎟⎠×100
Simplifying this gives:
=⎛⎝53−11⎞⎠×100=(23)×100=2003≈66.67%
The percentage increase in capacitance is approximately 66.67%.
Topper's Solved these Questions
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - HOTS
5 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITANCE BOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCE EXERCISE - EXEMPLAR PROBLEMS
3 Videos
- ELECTROMAGNETIC WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - Assertion And Reason
15 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
Similar Questions
A parallel plate air capacitor has capacitance C. Half of space between the plates is filled with dielectric of dielectric constant K as shown in figure . The new capacitance is C . Then

View SolutionA paralle plate air capacitor has capacitance C. Now half of the space is fillel with a dielectric material with dielectric constant K as shown in figure . The new capacitance is C . Then

View SolutionA parallel plate capacitor with air between the plates has a capacitance C. If the distance between the plates is doubled and the space between the plates is filled with a dielectric of dielectric constant 6, then the capacitance will become.
View SolutionA capacitor of plate area A and separation d is filled with two dielectrics of dielectric constant K1=6andK2=4. New capacitance will be

View SolutionAn LR circuit with a battery connected at t =0. Which of the following quantities will be zero just after the connection?
View SolutionA parallel plate capacitor has plate area of A separated by distance between them. It is completely filled with dielectric medium, its dielectric constant increases linearly as move from one plate to other plate from k1 to k2 . Then the capacitance of system is given by :
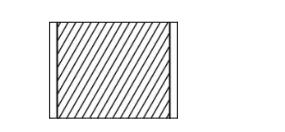
View SolutionA parallel plate capacitor has plate area of A separated by distance between them. It is completely filled with dielectric medium, its dielectric constant increases linearly as move from one plate to other plate from k1 to k2 . Then the capacitance of system is given by :

View SolutionA parallel plate condenser has a capacitance 50μF in air and 110μF when immersed in an oil. The dielectric constant k of the oil is
View SolutionA parallel plate capacitar, of capacitance, 20μF, is connected to a 100 V supply. After some time, the battery is disconnected , and the space, between the plates of the capacitor is filled with a dielectric, of dielectric constant 5. Calculate the energy stored in the capacitar (i) before (ii)after dielectric has been put in between its plates.
View SolutionConsider a parallel plate capacitor. When half of the space between the plates is filled with some dielectric material of dielectric constant K as Shown in Fig. (1) below, the capacitance is C1 . However , if the same dielectric material fills half the space as shown as shown in Fig. (2), the capacitance is C2. Therefore, the ratio C1:C2is
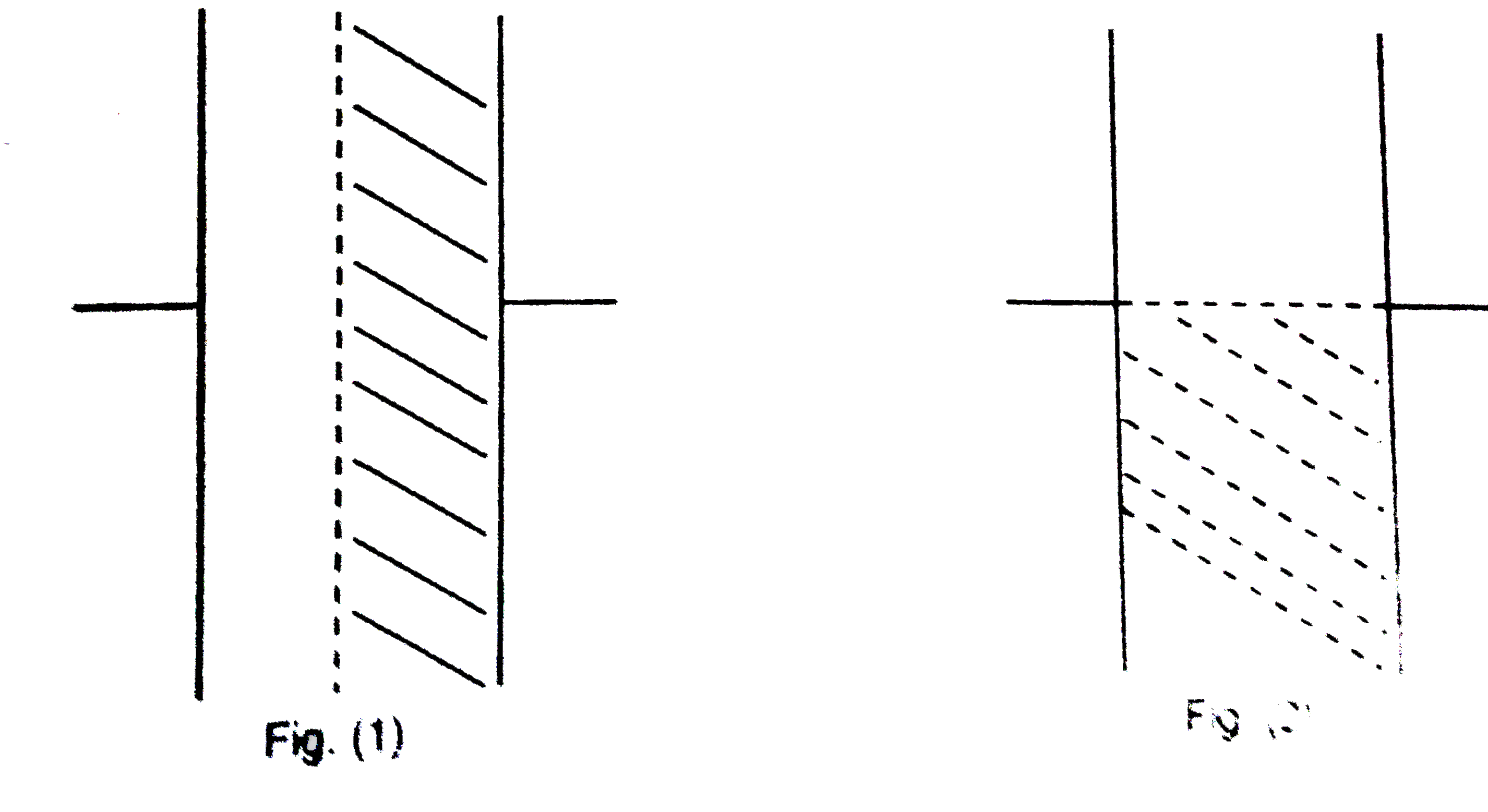
View Solution
