Question

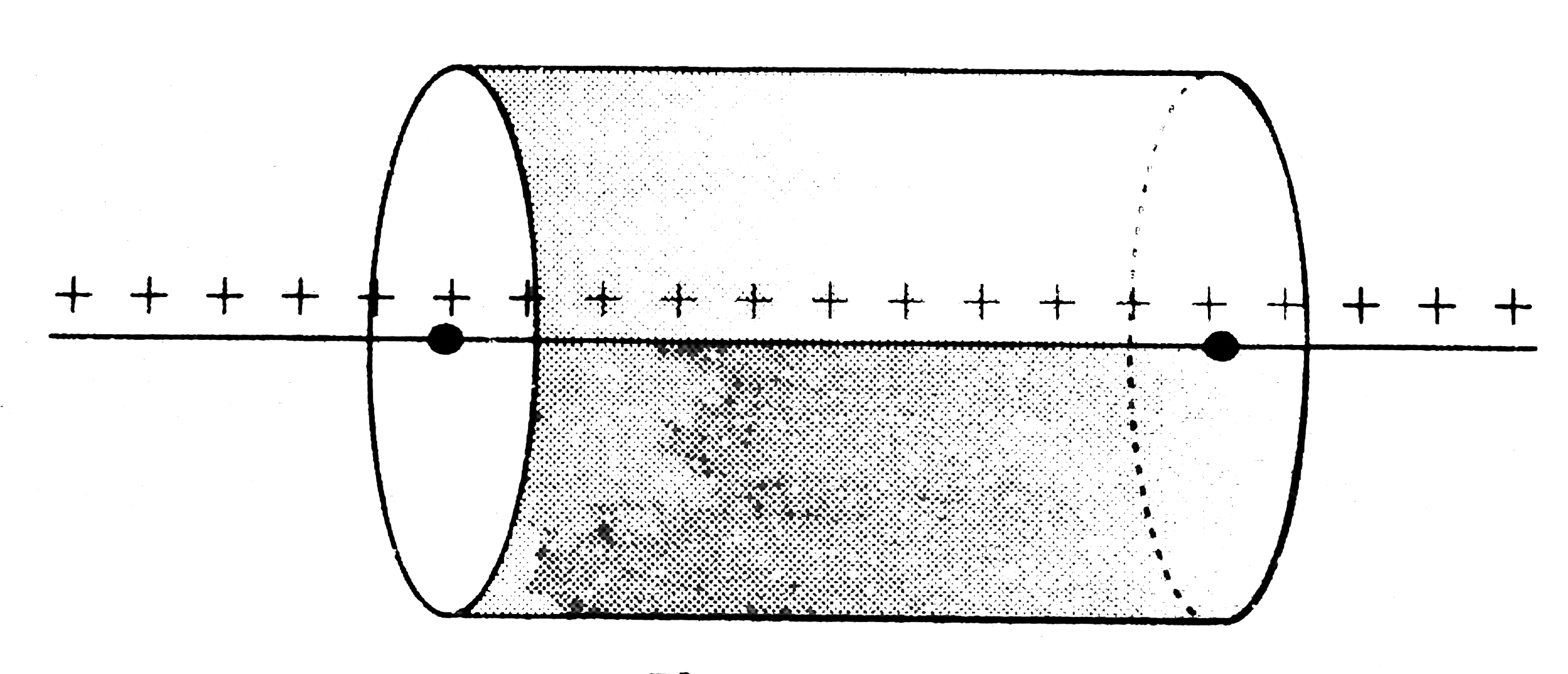
An infinite cylinder of radius $r_o,$ carrying linear charge density $\lambda .$ The equation of the equipotential surface for the cylinder is

Options

A. `r=r_(0)e^(pi epsi_(0)[V(r)+V(r_(0))]lamda)`
B. `r=r_(0)e^(2piepsi_(0)[V(r)-V(r_(0))]lamda^(2))`
C. `r=r_(0)e^(-2piepsi_(0)[V(r)=V(r_(0))]lamda)`
D. `r=r_(0)e^(-2piepsi_(0)[V(r)-V(r_(0))]lamda)`

Answer

C

Explanation

To find the equation of the equipotential surface for an infinite cylinder of radius $$R_0$$ carrying a linear charge density $$\lambda$$, we can follow these steps:

Step 1: Understand the setup
We have an infinite cylinder with radius \( R_0 \) and a linear charge density \( \lambda \). The electric field around a charged cylinder can be derived using Gauss's law.

Step 2: Apply Gauss's Law
Consider a cylindrical Gaussian surface of radius \( r \) (where \( r > R_0 \)) and length \( L \). According to Gauss's law:
\( \Phi_E = \frac{Q_{\text{enc}}}{\epsilon_0} \)
where \( \Phi_E \) is the electric flux through the Gaussian surface and \( Q_{\text{enc}} \) is the charge enclosed by the Gaussian surface.

Step 3: Calculate the charge enclosed
The charge enclosed by the Gaussian surface is:
\( Q_{\text{enc}} = L \lambda \)

Step 4: Calculate the electric field
The electric field \( E \) at a distance \( r \) from the axis of the cylinder can be expressed as:
\( E \cdot (2 \pi r L) = \frac{L \lambda}{\epsilon_0} \)
From this, we can solve for \( E \):
\( E = \frac{\lambda}{2 \pi \epsilon_0 r} \)

Step 5: Relate electric field to potential difference
The potential difference \( V \) between two points in an electric field is given by:
\( V = -\int E \, dl \)
For our case, we want to find the potential difference between the surface of the cylinder (at \( R_0 \)) and a point at distance \( r \):
\( V_r - V_{R_0} = -\int_{R_0}^{r} E \, dl \)

Step 6: Substitute the expression for \( E \)
Substituting the expression for \( E \):
\( V_r - V_{R_0} = -\int_{R_0}^{r} \frac{\lambda}{2 \pi \epsilon_0 l} \, dl \)

Step 7: Evaluate the integral
Evaluating the integral:
\( V_r - V_{R_0} = -\left( \frac{\lambda}{2 \pi \epsilon_0} \ln l \right)_{R_0}^{r} \)
This gives:
\( V_r - V_{R_0} = -\frac{\lambda}{2 \pi \epsilon_0} \left( \ln r - \ln R_0 \right) \)
\( = -\frac{\lambda}{2 \pi \epsilon_0} \ln \left( \frac{r}{R_0} \right) \)

Step 8: Rearranging the equation
Rearranging gives us:
\( \ln \left( \frac{R_0}{r} \right) = -\frac{2 \pi \epsilon_0}{\lambda} (V_r - V_{R_0}) \)

Step 9: Final equation for the equipotential surface
Exponentiating both sides leads to:
\( r = R_0 e^{-\frac{2 \pi \epsilon_0}{\lambda} (V_r - V_{R_0})} \)

This is the equation of the equipotential surface for the infinite charged cylinder.