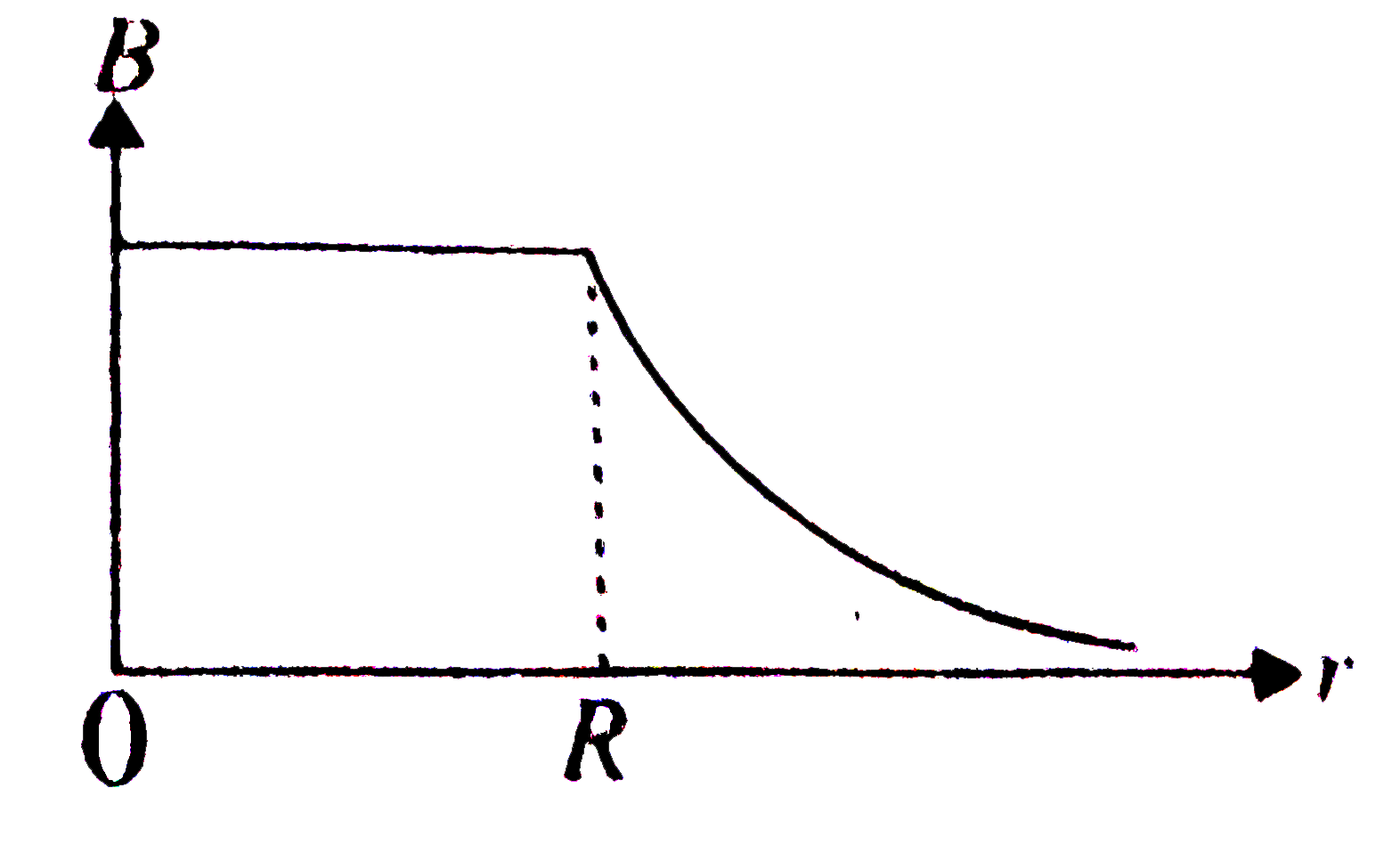
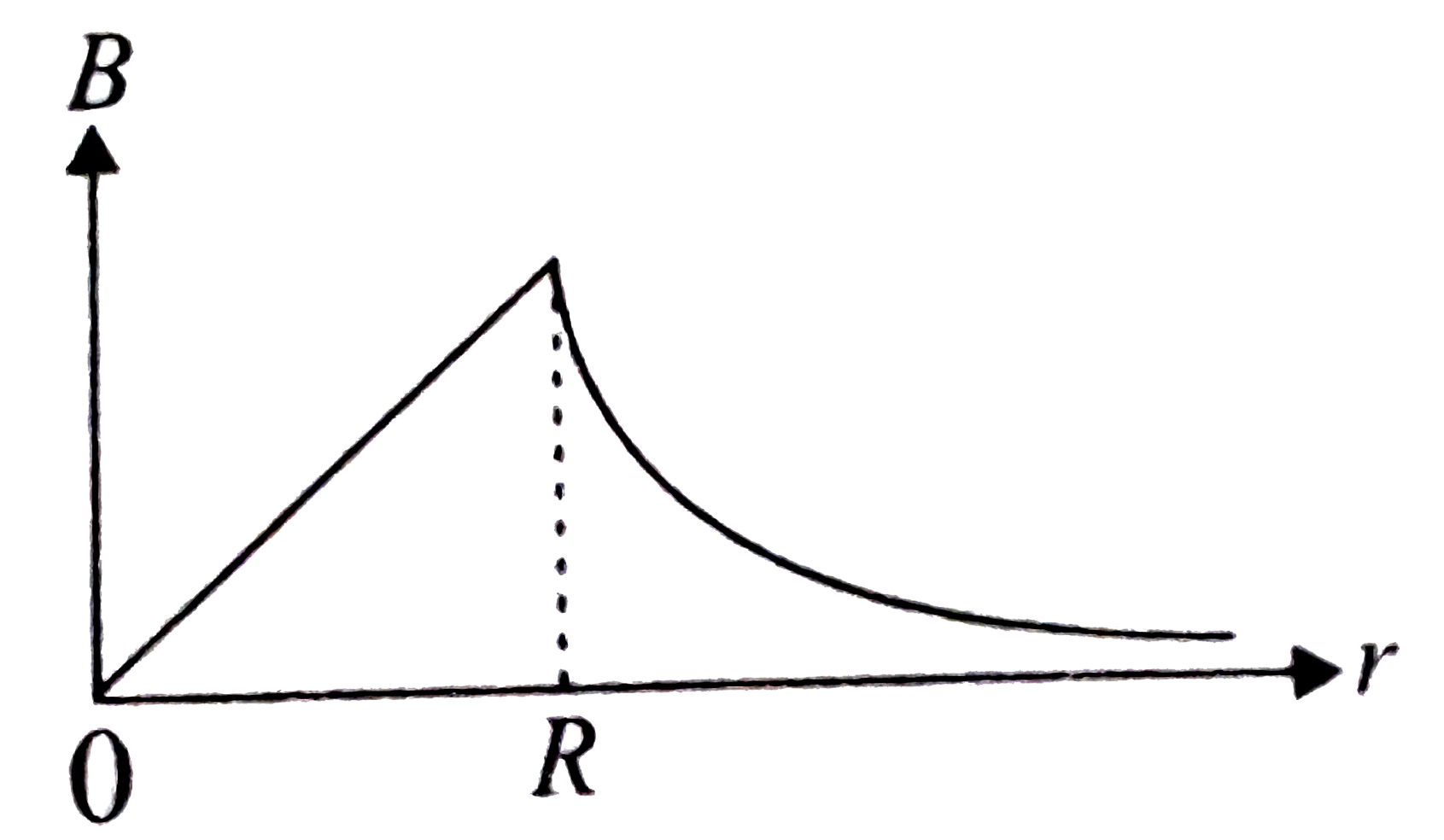
The correct plot of the magnitude of magnetic field →B vs distance r from centre of the wire is, if the radius of wire is R
The correct Answer is:B
To solve the problem of determining the correct plot of the magnitude of the magnetic field →B versus distance r from the center of a wire with radius R, we can follow these steps:
Step 1: Understand the Magnetic Field Inside and Outside the Wire
1. Inside the Wire (r<R): The magnetic field B inside a long straight wire carrying a current I is given by the formula:
B=μ0I2πR2r
where μ0 is the permeability of free space and r is the distance from the center of the wire. This indicates that the magnetic field B is directly proportional to r when r<R.
2. Outside the Wire (r>R): The magnetic field outside the wire is given by:
B=μ0I2πr
This shows that the magnetic field B is inversely proportional to r when r>R.
Step 2: Analyze the Behavior of B with Respect to r
- For r<R: As r increases from 0 to R, B increases linearly.
- For r>R: As r continues to increase beyond R, B decreases inversely.
Step 3: Sketch the Graph
- From r=0 to r=R: The graph will show a straight line with a positive slope (linear increase).
- From r=R onwards: The graph will show a curve that decreases, approaching the horizontal axis as r increases (inverse relationship).
Conclusion
The correct plot of the magnitude of the magnetic field B versus distance r from the center of the wire will show:
- A linear increase from the center of the wire to its surface (from r=0 to r=R).
- A decrease in the magnetic field as the distance increases beyond the radius of the wire (from r=R onwards).
Final Answer
The correct plot is one that shows a linear increase up to r=R and then a decrease for r>R.
---
1. Inside the Wire (r<R): The magnetic field B inside a long straight wire carrying a current I is given by the formula:
B=μ0I2πR2r
where μ0 is the permeability of free space and r is the distance from the center of the wire. This indicates that the magnetic field B is directly proportional to r when r<R.
B=μ0I2πr
This shows that the magnetic field B is inversely proportional to r when r>R.
- For r<R: As r increases from 0 to R, B increases linearly.
- For r>R: As r continues to increase beyond R, B decreases inversely.
- From r=0 to r=R: The graph will show a straight line with a positive slope (linear increase).
- From r=R onwards: The graph will show a curve that decreases, approaching the horizontal axis as r increases (inverse relationship).
The correct plot of the magnitude of the magnetic field B versus distance r from the center of the wire will show:
- A linear increase from the center of the wire to its surface (from r=0 to r=R).
- A decrease in the magnetic field as the distance increases beyond the radius of the wire (from r=R onwards).
The correct plot is one that shows a linear increase up to r=R and then a decrease for r>R.
Topper's Solved these Questions
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - HOTS
5 Videos
- MOVING CHARGES AND MAGNETISM BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISM EXERCISE - EXEMPLAR PROBLEMS
6 Videos
- MAGNETISM AND MATTER BOOK - NCERT FINGERTPS ENGLISHCHAPTER - MAGNETISM AND MATTER EXERCISE - NCERT Exemplar
5 Videos
- NUCLEIBOOK - NCERT FINGERTPS ENGLISHCHAPTER - NUCLEIEXERCISE - Assertion And Reason
15 Videos
Similar Questions
A cylinderical conductor of radius R is carrying constant current. The plot of the magnitude of the magnetic field, B with the distance, d from the centre of the conductor , is correctly represented by the figure:
View SolutionA sphere of radius R carries charge such that its volume charge density is proportional to the square of the distance from the centre. What is the ratio of the magnitude of the electric field at a distance 2 R from the centre to the magnitude of the electric field at a distance of R/2 from the centre?
View SolutionA cylindrical long wire of radius R carries a current I uniformly distributed over the cross sectional area of the wire. The magnetic field at a distance x from the surface inside the wire is
View SolutionThe field due to a magnet at a distance R~ from the centre of the magnet is proportional
View SolutionMagnetic field at a distance a from long current carrying wire is proportional to
View SolutionA uniform but time-varying magnetic field B(t) exists in a circular region of radius a and is directed into the plane of the paper, as shown. The magnitude of the induced electric field at point P at a distance r from the centre of the circular region
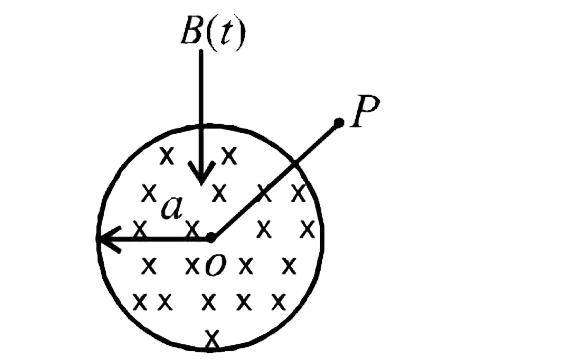
View SolutionTwo wires AO and OC carry equal currents i as shown in Fig. One end of both the wires extends to infinity. Angle AOC is α. The magnitude of magnetic field is point P on the bisector of these two wires at a distance r from point O is

View SolutionA circular coil of radius R carries a current i. The magnetic field at its centre is B. The distance from the centre on the axis of the coil where the magnetic field will be B/8 is
View SolutionA circular coil of radius R carries an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varies as
View SolutionA circular coil of radius R carries an electric current. The magnetic field due to the coil at a point on the axis of the coil located at a distance r from the centre of the coil, such that r>>R, varies as
View Solution
