Two particles move in a uniform gravitational field with an acceleration g. At the initial moment the particles were located at one point and moved with velocities u1=3.0ms−1 and u2=4.0ms−1 horizontally in opposite directions. Find the distance betwen the particles at the moment when their velocity vectors become mutually perpendicular.
The situation is shown fig.

Let the velocity vectors become perpendicular after time t when both particles has fallen same vertical distance 12gt2 and have acquired same vertical velocites gt.
Let their resultant velocities make angle θ1 and θ2 with horizontal.
Then tanθ1=gtv1 and tanθ2=gtv2
Since velocity vectors are perpendicular
θ1+θ2=90∘, hence tanθ2=cotθ1
It makes tanθ1=gtv1 and cotθ1=gtv2
On multiplying we get t=√v1v2g and D=(u1+u2)t
Similar Questions
Two particles move in a uniform gravitational field with an acceleration g. At the initial moment the particles were located over a tower at one point and moved with velocities v1=3m/sandv2=4m/s horizontally in opposite directions. Find the distance between the particles at the moment when their velocity vectors become mutually perpendicular.
View SolutionTwo particles move in a uniform gravitational field with an acceleration g. At the initial moment the particles were located over a tower at one point and moved with velocities v1=3m/sandv2=4m/s horizontally in opposite directions. Find the distance between the particles at the moment when their velocity vectors become mutually perpendicular.
View Solution
Knowledge Check
Two particles move in a uniform gravitational field with an acceleration g.At the initial moment the particles were located at same point and moved with velocities u1=9ms−1 and u2=4ms−1 horizontally in opposite directions.The time between the particles at the moment when their velocity vectors are mutually perpendicular in s in (take g=10ms−2)
A0.36B3.6C0.6D6
Two particles move in a uniform gravitational field with an acceleration g. At the intial moment the particles were located at one point and moved with velocity v1=1 ms−1 and v2=4 ms−1 horizontally in opposite directions. Find the time interval after their velocity vectors become mutually perpendicular
View SolutionTwo particles move in a uniform gravitational field with an acceleration g. At the intial moment the particles were located at one point and moved with velocity v1=1 ms−1 and v2=4 ms−1 horizontally in opposite directions. Find the time interval after their velocity vectors become mutually perpendicular
View SolutionFrom the top of the tower, two balls are thrown horizontally in opposite directions with velocities u1 and u2. Find the distacne between the balls at the moment when their velocity vectors becomes mutually perpendicular. (Assume the height of tower very large)
View SolutionA particle moves in a straight line with a uniform acceleration a. Initial velocity of the particle is zero. Find the average velocity of the particle in first 's' distance.
View SolutionA particle moves in a straight line with a uniform acceleration a. Initial velocity of the particle is zero. Find the average velocity of the particle in first 's' distance.
View SolutionTwo particles are thrown simultaneously from points A and B with velocities u1=2ms−1andu2=14ms−1, respectively, as shown in figure.
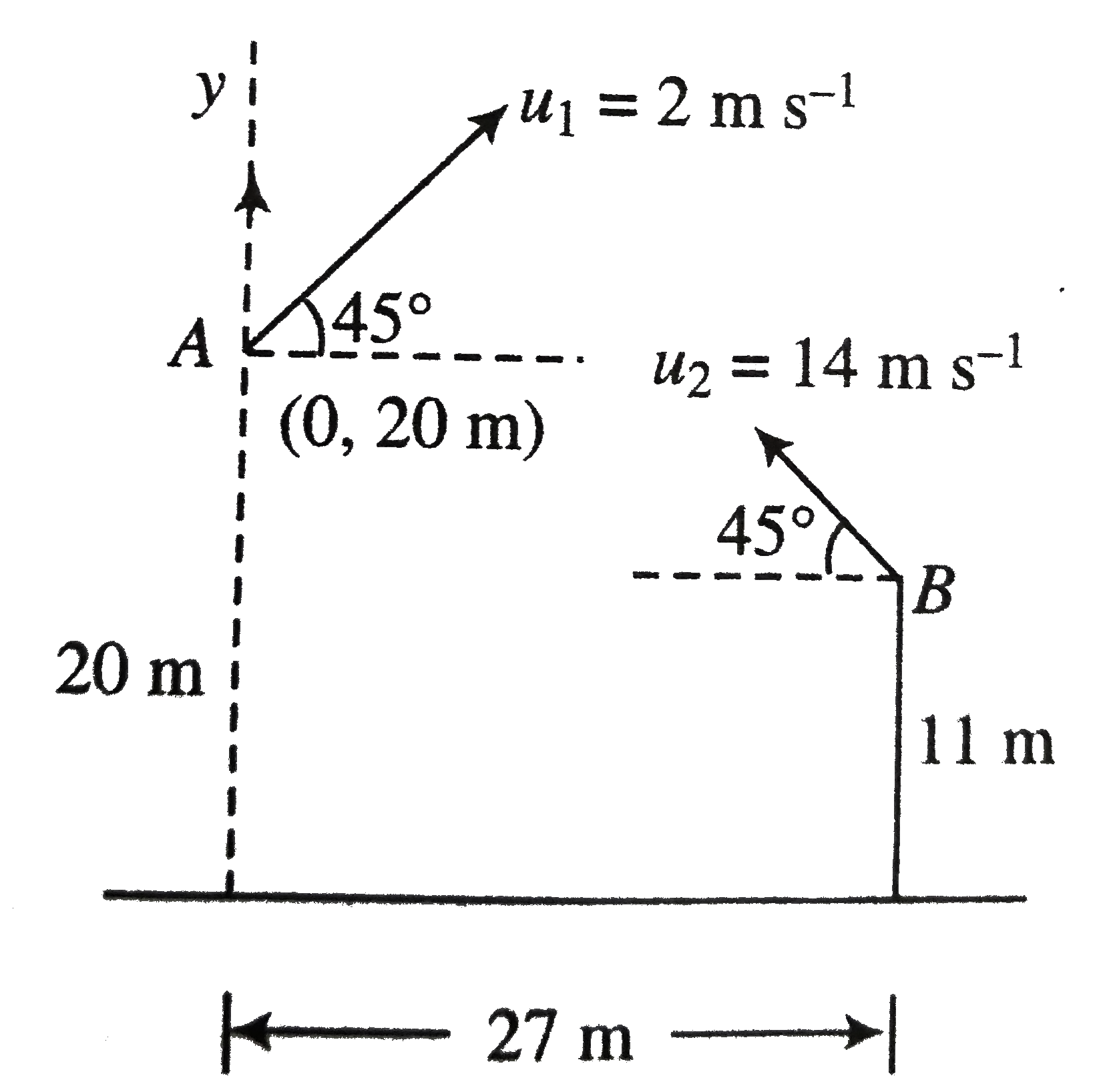
The direction (angle) with horizontal at which B will appear to move as seen from A is
View SolutionA particle is moving with initial velocity 1m/s and acceleration a=(4t+3)ms2. Find velocity of particle at t=2sec.
View Solution
