Figure given below shows the displace show ment versus time graph for two particles A and B executing simple harmonic motions. Find the ratio of their maximum velocities,
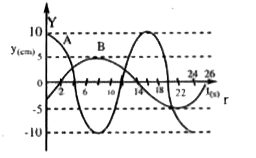
For A, time period TA = 16s (Distance between two adjacent crets)
for B, time period TB=(26−2)=24s [length between the crest and trough shown = 20s-8s 12s]
Also, amplitudes aA=10cm,aB=5cm
Ratio of maximum velocities
VAVB=aAωAaBωB=aATBaBTA=10×245×16=31
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE I
184 Videos
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE II
175 Videos
- OSCILLATIONSBOOK - AAKASH SERIESCHAPTER - OSCILLATIONSEXERCISE - EXERCISE-3
49 Videos
- OSCILLATIONBOOK - AAKASH SERIESCHAPTER - OSCILLATIONEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
12 Videos
- PHYSICAL WORLDBOOK - AAKASH SERIESCHAPTER - PHYSICAL WORLDEXERCISE - VERY SHORT ANSWER TYPE QUESTIONS
5 Videos
Similar Questions
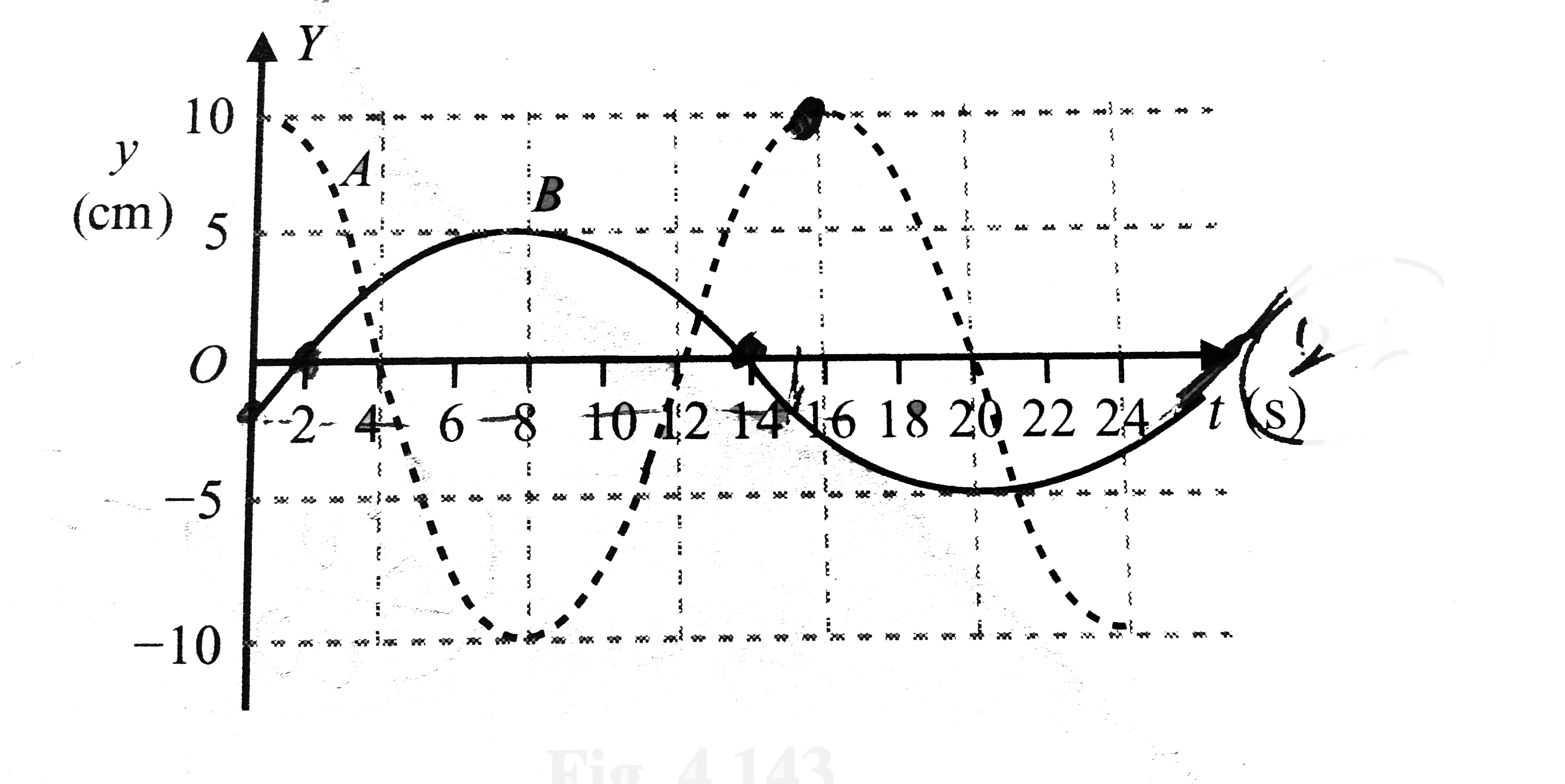
The following figure shows the displacement versus time graph for two particles A and B executing simple harmonic motions. The ratio of their maximum velocities is
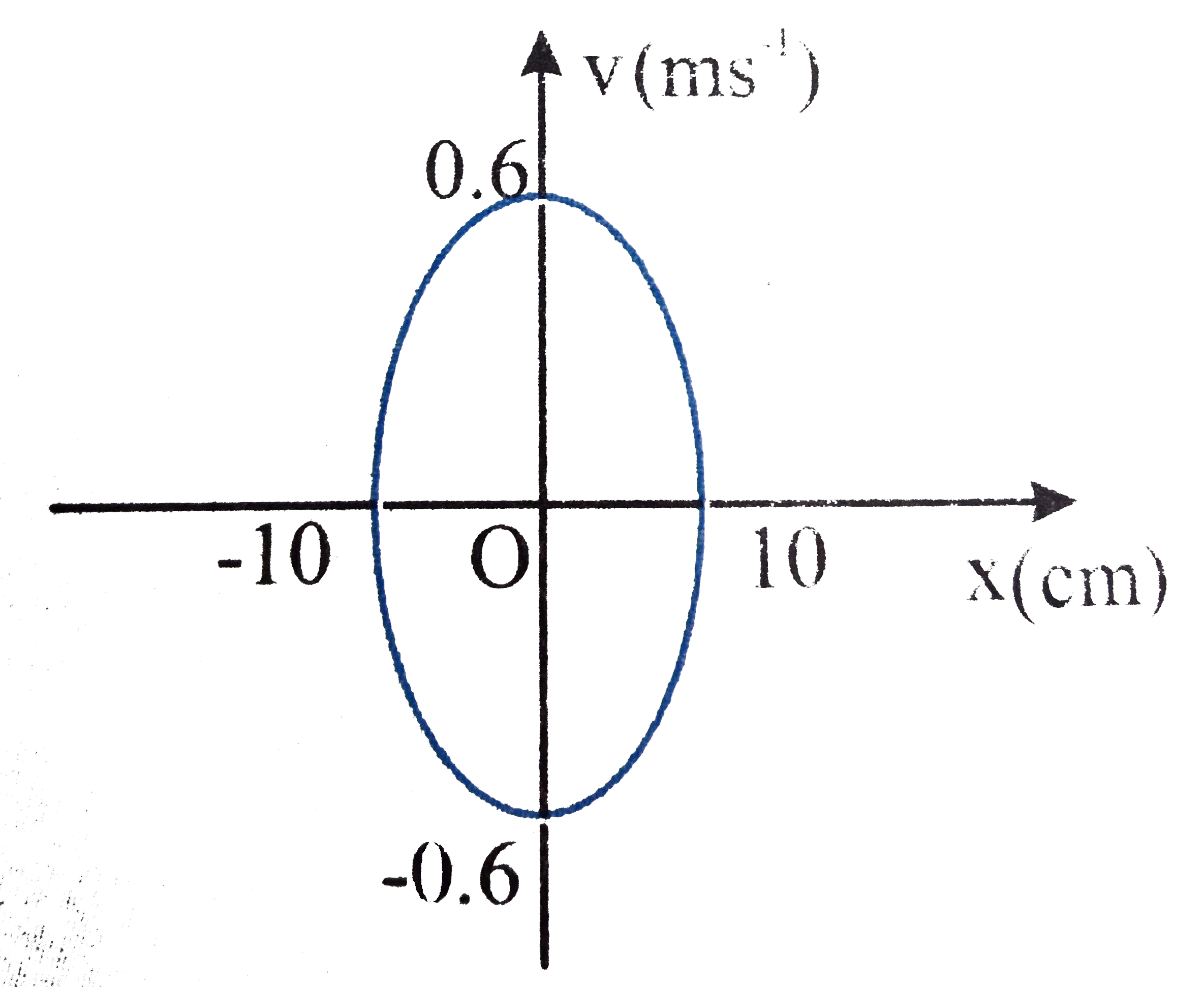
View SolutionFigure shows the graph of velocity versus displacement of a partciel executing simple harmonic motion. Find the period of oscillation of the particle.
View Solution
Knowledge Check
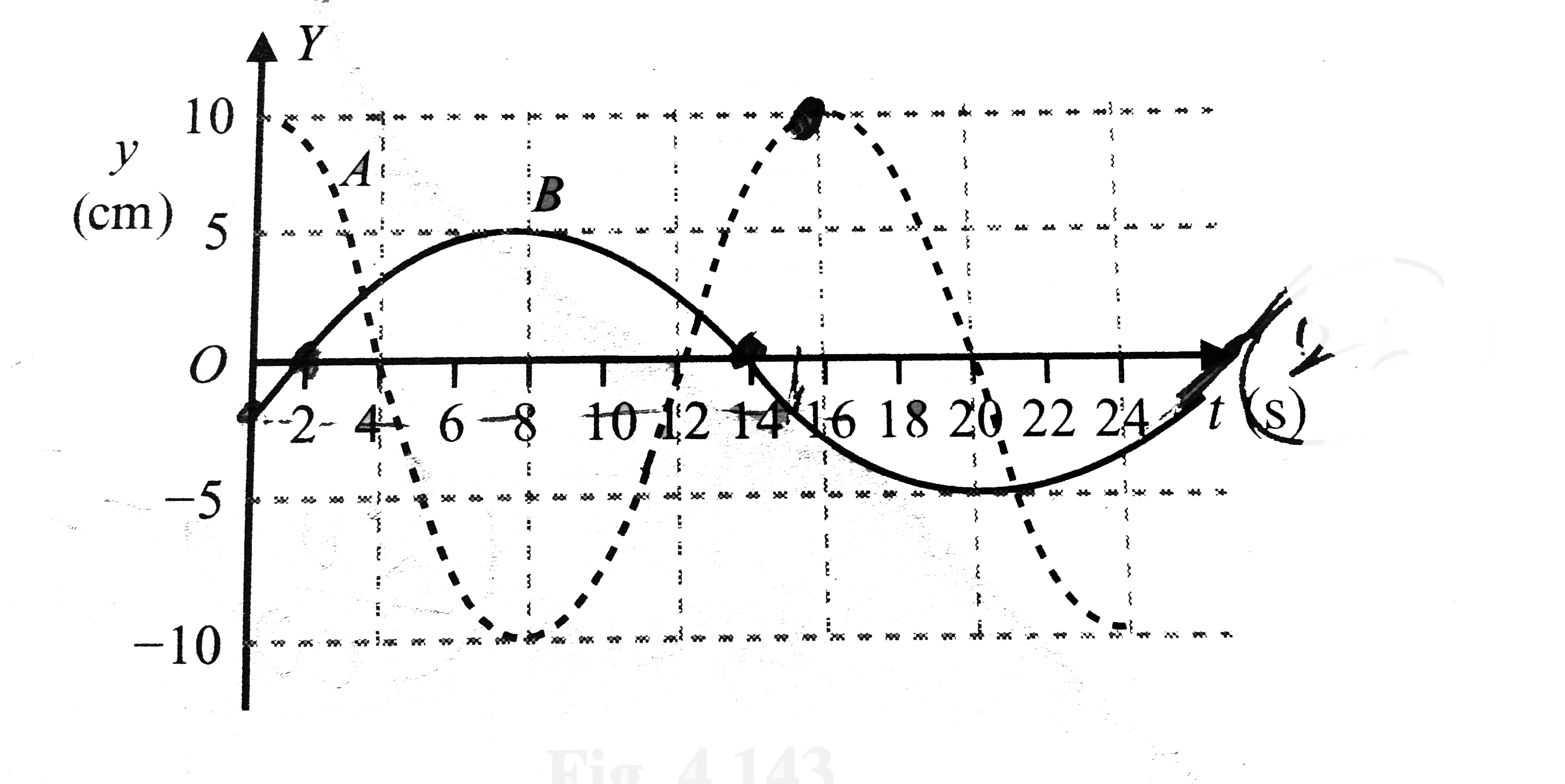
The following figure shows the displacement versus time graph for two particles A and B executing simple harmonic motions. The ratio of their maximum velocities isA3:1B1:3C1:9D9:1The velocity of a particle executing simple harmonic motion is
Aω2√A2+x2Bω√A2−x2Cω√A2+x2Dω2√A2−x2The total energy of a particle having a displacement x, executing simple harmonic motion is
A∝xB∝x2Cindependent of xD∝x1/2
The following figure shows displacement versus time curve for a particle executing simple harmonic motion :
Which one of the following statements is correct?
View SolutionThe total energy of a particle having a displacement x, executing simple harmonic motion is
View SolutionDraw displacement-time, velocity-time and acceleration-time graphs for a particle executing simple harmonic motion. Discuss their phase relationship.
View SolutionWhen the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is
View SolutionThe shape of graph plotted between velocity and position of a particle executing simple harmonic motion is
View Solution
