A regular polygon of 'n sides is formed by bending a wire of length $2 π R$ which carries a current i. Find the magnetic field at the centre of the polygon?

Text Solution

Verified by Experts

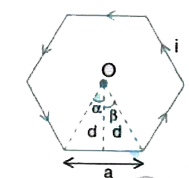
One side of polygon $a = \frac{2 π r}{n}$
$α = β = (\frac{2 π}{n}) / 2 = \frac{π}{n}, cot α = \frac{d}{a / 2}$
$d = \frac{a}{2} cot α = \frac{π R}{n} cot (\frac{π}{n})$
All sides of polygen produce magnetic field at O in same direction.
so,, B = n (magnetic field due to one side)
$= n \frac{μ_{0} i}{4 π} \frac{n}{π R cot \frac{π}{n}} (sin \frac{π}{n} + sin \frac{π}{n})$
$= \frac{μ_{0} i}{4 R} \frac{n^{2}}{π^{2}} \frac{2 sin π / n}{cos π / n} . sin \frac{π}{n}$
$B = \frac{μ_{0} i}{2 R} \times \frac{{sin π / n /_{π / n}}_{π / n}^{2}}{cos π / n}$

|

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

If a thin wire of length L is given the shape of a regular polygon of n sides and current I flows through it, what will be the net magnetic field at the centre of the polygon if n is infinity?
A $\frac{μ_{0} n i}{2 L}$
B $\frac{μ_{0} n i}{2 π L}$
C $\frac{μ_{0} π i}{L}$
D $\frac{μ_{0} n π i}{L}$

AAKASH SERIES-ELECTROMAGNETICS-ADDITIONAL EXERCISE

Home
Ask Doubt
Profile