A square current carrying loop made of thin wire and having a mass m =10g can rotate without friction with respect to the vertical axis $O O_{1}$ , passing through the centre of the loop at right angles to two opposite sides of the loop. The loop is placed in a homogeneous magnetic field with an induction $B = 10^{- 1} T$ directed at right angles to the plane of the drawing. A current I = 2A is flowing in the loop. Find the period of small oscillations that the loop performs about its position of stable equilibrium.

Text Solution

Verified by Experts

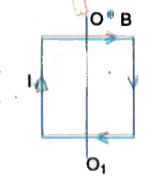
$T_{0} = 2 π \frac{\sqrt{m}}{6 I B} = 0.57 s$
[Hint : Restoring torque equation :
$I A B sin θ = - I_{0} θ,$ where $A = a^{2}$
and $I_{0} = \frac{m}{4} [\frac{a^{2}}{12} \times 2 + {(\frac{a}{2})}^{2} \times 2]$
$= \frac{m}{4} \times \frac{2}{3} a^{2}$
$\to (\frac{m}{6} a^{2}) . . θ = - a^{2} B θ \Rightarrow . . = - (\frac{6 I B}{M}) θ]$ .

Updated on:21/07/2023

shows a square current carrying loop $A B C D$ of side $10 c m$ and current $I = 10 A$ The magnetic moment $- \to M$ of the loop is
.
A $(0.05) (ˆ i - \sqrt{3} ˆ k) A - m^{2}$
B $(0.05) (ˆ j - \sqrt{3} ˆ k) A - m^{2}$
C $(0.05) (\sqrt{3} ˆ i + ˆ k) A - m^{2}$
D $(ˆ i + ˆ k) A - m^{2}$
A triangular loop of side 1 carries a current I. It is placed in a magnetic field B such that the plane of the loop is in the direction of B. The torque on the loop is
AlBl
Bzero
C $l^{2} B l$
Dinfinity
A triangular loop of side $l$ carries a current $I$ . It is placed in a magnetic field $B$ such that the plane of the loop is in the direction of $B$ . The torque on the loop is
AZero
BIBl
C $\frac{\sqrt{3}}{2} I I^{2} B^{2}$
D $\frac{\sqrt{3}}{4} I B l^{2}$