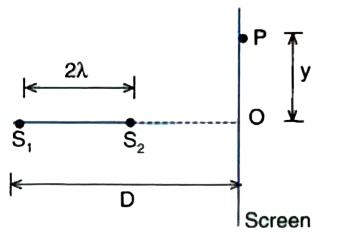
Two coherent narrow slits emitting sound of wavelength λ in the same phase are placed parallel to each other at a small separation of 2λ. The sound is detected by moving a detector on the screen at a distance D(>>λ) from the slit S1 as shown in figure. Find the distance y such that the intensity at P is equal to intensity at O.
 Solution in Telugu
Solution in Telugu
Let Δx=λ at angle θ (see fig) Path difference between the waves is Δx=2λcosθ
∴2λcosθ=λ(Δx=λ)orθ=60∘
Now, PO=S1Ocot30∘ory=√3D
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - EXERCISE (LONG ANSWER QUESTIONS)1videos
WAVE MOTION AND SOUND
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTION AND SOUNDEXERCISE - EXERCISE (VERY SHORT ANSWER QUESTIONS)25videos
WAVE MOTION AND SOUND
- BOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (INTEGER TYPE QUESTIONS)10videos
WAVE MOTION
- BOOK - AAKASH SERIESCHAPTER - WAVE OPTICSEXERCISE - PROBLEMS (LEVEL - II)20videos
WAVE OPTICS
Similar Questions
What is Doppler effect? Find an expression for the apparent frequency heard when the source is in motion and the listener is at rest. What is the limitation of Doppler effect?
View SolutionWhen sound travels from air into water, does the frequency of the wave change? The wavelength? The speed?
06:11View SolutionA source emitting sound of frequency 180 Hz is placed in front of a wall at a distance of 2m from it. a detector is also placed in front of the wall at the same distance from it. The minimum distance between the source and the detector is x mtrs for which the detector shows a maxima of sound. Speed of sound in air = 360 m/s
View SolutionIn Young's double slit experiment the y co-ordinates of central maxima and 10th maxima are 2 cm and 5 cm respectively. When the YDSE apparatus is immersed in a liquid of refractive index 1.5. Find the corresponding y co-ordinates.
03:33View SolutionTwo coherent narrow slits emitting sound of wavelength lamda in the same phase are placed parallel to each other at a small separation of 2lamda . The sound is detected by moving a detector on the screen sum at a distance D(gtgtlamda) from the slit S_1 as shown in figure. Find the distance x such that the intensity at P is equal to the intensity at O.
08:52View SolutionTwo coherent narrow slits emitting light of wavelength lambda in the same phase are placed parallel to each other at a small separation of 2lambda . The light is collected on a screen S which is placed at a distance D(gtgt lambda ) from the slit S_1 as shown in figure. Find the finite distance x such that the intensity at P is equal to intensity O. .
08:39View SolutionTwo coherent narrow slits emitting wavelength lambda in the same phase are placed parallel to each other at a small separation of 2lambda , the sound is detected by moving a detector on the screen S at a distance D (gtgt lambda) from the slit S_(1) as shown in Fig. 7.76. Find the distance x such that the intensity at P is equal to the intensity at O .
05:07View SolutionTwo coherent narrow slits emitting light of wavelength lamda in the same phase are placed parallel to each other at a small separation of 3lamda the light is collected on a screen S which is placed at a distance D(gt gt lamda) from the slits the smallest distance x such that the P is a maxima.
05:22View SolutionThree narrow slits A, B and C are illuminated by a parallel beam of light of wavelengthλ , P is a point on the screen exactly in front of point A. Slit plane is at a distance D from the screen(D>>λ). It is know that BP – AP =13
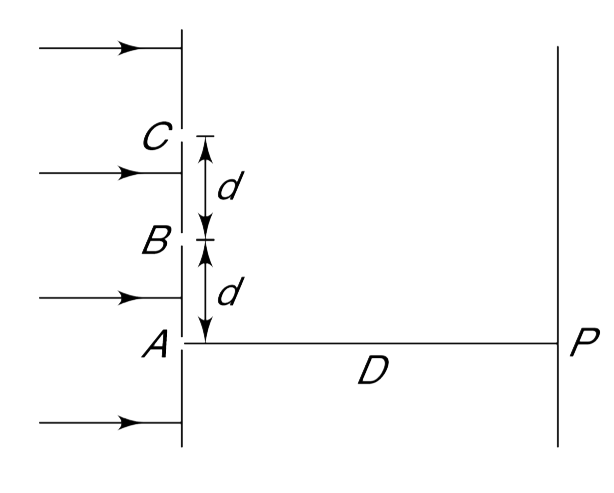
(a) Find d in terms of D andλ
(b) Write the phase difference between waves reaching at P from C and A.
(c) If intensity of P due to any of the three slits individu- ally is I0, find the resultant intensity at P.View SolutionTwo coherent sources Sf and S2 (in phase with ech other) are placed at a distance of 2λ as shown where λ is wavelength of sound. A detector moves on line AB parrallel to S1S2. If detector detects maximum intensity at finite distance from O at θ=±(πn). Find n
 04:21View Solution
04:21View SolutionTwo coherent sound sources A and B produce a sound of wavelength lambda. If at t=0 a detector starts moving with constant velocity v_(0) from point O along y - direction, then the minimum time, after which it detects the sound of maximum intensity, is (D gt gt lambda)
02:46View SolutionTwo coherent point sources S_1 and S_2 vibrating in phase emit light of wavelength lamda . The separation between them is 2lamda . The light is collected on a screen placed at a distance Dgt gt lamda from the slit S_1 as shown. The minimum distance, so that intensity at P is equal to the intensity at O
05:44View SolutionTwo coherant narrow slits emitting light of wavelength lambda in the same phase are placed parallel to each other at a small separation of 2lambda .The light is collected on a screen S which is placed at a distance D( gt gt A) from the slit S_(1) as shown in figure .Find teh finite distance x such that the intensity of P is equal to intensity at O .
06:09View SolutionConsider the situation shown in figure. The two slite S_1 and S_2 placed symmetrically around the centre line are illuminated by a monochromatic light of wavelength lambda. The separation between the slits is d. The light transmitted by the slits falls on a screen M_1 placed at a distance D from the slits. The slit S_3 is at the centre line and the slit S_4 is at a distance y form S_3 . Another screen M_2 is placed at a further distance D away from M_1 . Find the ration of the maximum to minimum intensity observed on M_2 if y is equal to (dltltD) .
View SolutionTwo coherent narrow slits emitting light of wavelength lambda in the same phase are placed parallel to each other at a small separation of 2lambda . The light is collected on a screen S which is placed at a distance D(gtgt lambda ) from the slit S_1 as shown in figure. Find the finite distance x such that the intensity at P is equal to intensity O. .
05:52View SolutionTwo coherent sources Sf and S2 (in phase with ech other) are placed at a distance of 2λ as shown where λ is wavelength of sound. A detector moves on line AB parrallel to S1S2. If detector detects maximum intensity at finite distance from O at θ=±(πn). Find n
 03:53View Solution
03:53View Solution

