A small mass m attached to one end of a spring with a negligible mass and an unstretched length L, executes vertical oscillations with angular frequency ω0 When the mass is rotated with an angular speed ω by holding the other end of the spring at a fixed point, the mass moves uniformly in a circular path in a horizontal plane. Then the increase in length of the spring during this rotation is
ω2Lω20−ω2
ω20Lω2−ω20
ω2Lω20
ω20Lω2
The correct Answer is:A
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBook:MTG-WBJEEChapter:OSCILLATIONS AND WAVESExercise:WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 3: ONE OR MORE THAN ONE OPTION CORRECT TYPE (2 MARKS))
Explore 2 Videos
- OSCILLATIONS AND WAVESBook:MTG-WBJEEChapter:OSCILLATIONS AND WAVESExercise:WB JEE PREVIOUS YEARS QUESTIONS (CATEGORY 1: SINGLE OPTION CORRECT TYPE (1 MARK))
Explore 16 Videos
- NUCLEAR PHYSICBook:MTG-WBJEEChapter:NUCLEAR PHYSICExercise:WB JEE PREVIOUS YEARS QUESTIONS
Explore 5 Videos
- PARTICLE NATURE OF LIGHT AND WAVE PARTICLE DUALISMBook:MTG-WBJEEChapter:PARTICLE NATURE OF LIGHT AND WAVE PARTICLE DUALISMExercise:WB JEE PREVIOUS YEARS QUESTIONS
Explore 15 Videos
Similar Questions
"A mass is attached to one end of a spring and by holding the other end in hand, the spring is whirled in a horizontal circle" What happens to the length of the spring ?
View SolutionA particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
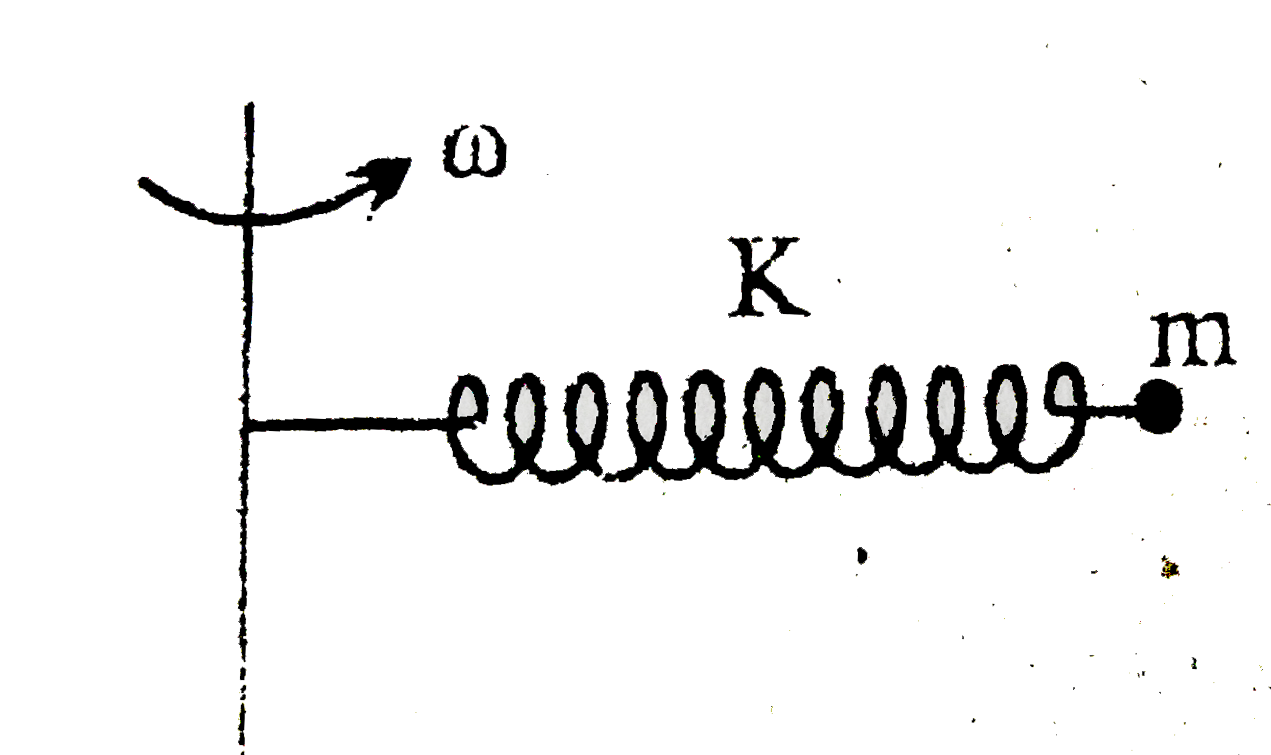
View Solution
Knowledge Check
A particles of mass m is fixed to one end of a light spring of force constant k and unstreatched length l. the system is rotated about the other end of the spring with an angular velocity ω in gravity free space. The increase in length of the spring is
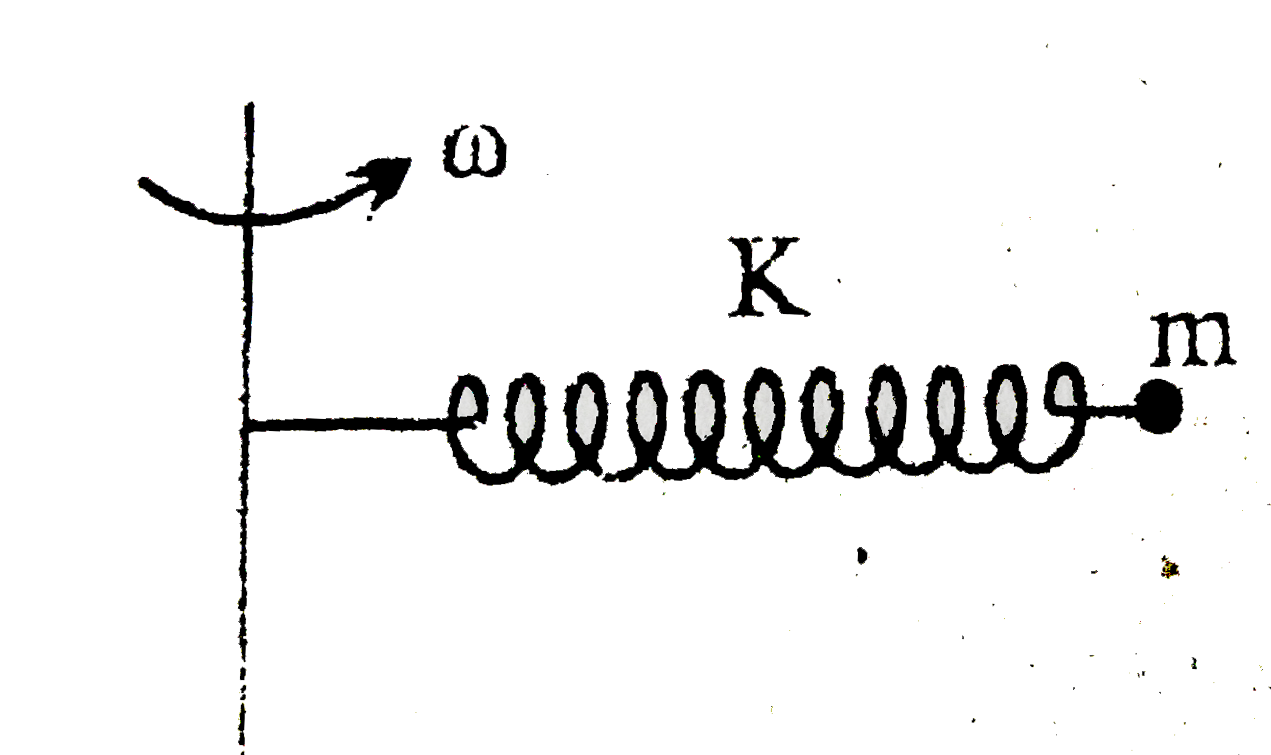 Amω2lkBmω2lk−mω2Cmω2lk+mω2Dnone of these
Amω2lkBmω2lk−mω2Cmω2lk+mω2Dnone of theseA particle of mass m is fixed to one end of a light spring of force constant k and unstretched length l. The other end of the spring is fixed on a vertical axis which is rotaing with an angular velocity ω. The increase in length of th espring will be
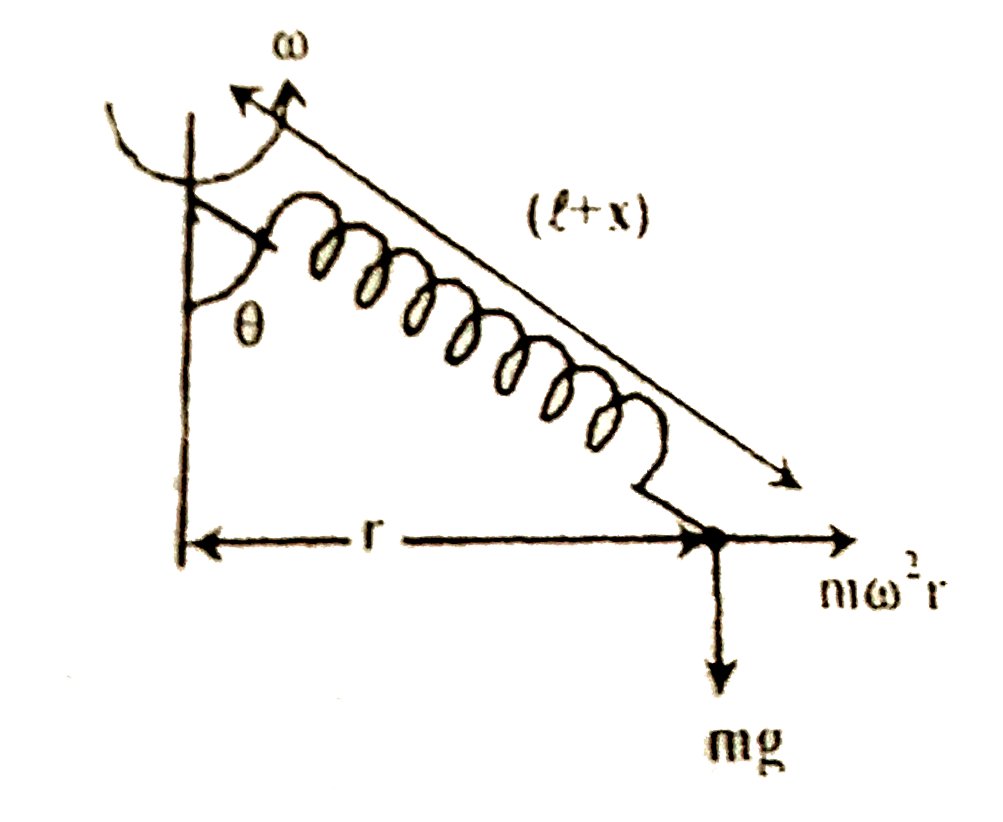 Amω2lkBmω2lk−mω2Cmω2lk+mω2DNone of these
Amω2lkBmω2lk−mω2Cmω2lk+mω2DNone of theseA uniform chain of mass M and length L is rotated in a horizontal circle about its one end at an angular frequency ω. The tension at its axis is
AMω2LBMω2L8CMω2L4DMω2L2
A block of mass m is connected at one end of spring fixed at other end having natural length l0 and spring constant K. The block is rotated with constant angular speed (ω) in gravity free space. Find the elongation in spring.
View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionA block of mass m is connected at one end of spring fixed at other end having natural length l0 and spring constant K. The block is rotated with constant angular speed (ω) in gravity free space. Find the elongation in spring.
View SolutionA mass m attached to spring of natural length l0 and spring constant k. One end of string is attached to centre of disc in horizontal plane which is being rotated by constant angular speed ω. Find extension per unit length in spring (given k>>mω2 ) :
View SolutionIn a spring- mass system , the length of the spring is L, and it has a mass M attached to it and oscillates with an angular frequency ω. The spring is then cut into two parts, one (i) with relaxed length αL and the other (ii) with relaxed length (1−α) L. The force constants of the two spring A and B are
View Solution
