त्रिभुज का आधार तीन समान भागों में विभाजित किया गया है। यदि इन भागों का सम्मुख शीर्ष पर अन्तरित कोणों की स्पर्श t1(,t2,t3 है, तो सिद्ध कीजिए. (1t1+1t2)(1t2+1t3)=4(1+1t22)
The correct Answer is:(ii)6
Step by step video, text & image solution for त्रिभुज का आधार तीन समान भागों में विभाजित किया गया है। यदि इन भागों का सम्मुख शीर्ष पर अन्तरित कोणों की स्पर्श t_(1)(,t_(2),t_(3) है, तो सिद्ध कीजिए. ((1)/(t_(1))+(1)/(t_(2)))((1)/(t_(2))+(1)/(t_(3)))=4(1+(1)/(t_(2)^(2))) by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Topper's Solved these Questions
- BOOK - ALLEN HINDICHAPTER - त्रिभुज के हल EXERCISE - EXERCISE-01(सही विकल्प चुनिए (केवल एक सही उत्तर है ))22videos
त्रिभुज के हल
- BOOK - ALLEN HINDICHAPTER - त्रिभुज के हल EXERCISE - EXERCISE-01(सही विकल्प चुनिए (कएक या एक से अधिक सही उत्तर है ) )4videos
त्रिभुज के हल
- BOOK - ALLEN HINDICHAPTER - त्रिकोणमीतीय समीकरणEXERCISE - EXERCISE-05 [B] JEE-[ADVANCED] : PREVIOUS YEAR QUESTIONS7videos
त्रिकोणमीतीय समीकरण
- BOOK - ALLEN HINDICHAPTER - द्विघात समीकरण EXERCISE - EXERCISE-4 B11videos
द्विघात समीकरण
Similar Questions
एक त्रिभुज में ∠A=55∘ तथा ∠B=15∘, तब c2−a2ab बराबर होगा
05:29View Solution△ABC में, A=π3 तथा b: c=2: 3 है। यदि tanα=√35,0<α<π2 -हो, तो .
12:25View Solutionk के उन पूर्णांक मानों की संख्या , जिनके लिए समीकरण 7cosx+5sinx=2k+1 का एक हल होगा , है
03:54View Solutionयदि द्विघात समीकरण ax2+bx+c=0 का एक मूल दूसरे मूल का n वीं घात के बराबर है, तो दिखाइए की
(acn)1n+1+(anc)1n+1+b=004:22View SolutionThe base of a triangle is divided into three equal parts. If t1,t2,t3 are the tangents of the angles subtended by these parts at the opposite vertex, prove that (1t1+1t2)(1t2+1t3)=4(1+1t22).
05:45View SolutionIf the normals at points t1andt2 meet on the parabola, then t1t2=1 (b) t2=−t1−2t1 t1t2=2 (d) none of these
02:11View Solution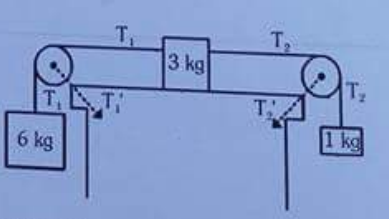
Calculate a, T1,T2,T1'&T2'.01:07View Solutionयदि एक वृत्त किसी समकोणीय अतिपरवलय से चार बिन्दुओं t1,t2,t3 तथा t4 में मिलता है, तो सिद्ध कीजिए कि t1,t2,t3,t4=1.
View Solutionदर्शाइए कि परवलय y2=4ax पर बिंदुओं t1,t2 और t3 से बने त्रिभुज के क्षेत्रफल का परिमाण a2(t1−t2)(t2−t3)(t3−t1) है।
05:40View SolutionIf the tangents at t1andt2 to a parabola y2=4ax are perpendicular then (t1+t2)2−(t1−t2)2=
06:44View SolutionThe area of triangle formed by the points points and t1,t2 and t3 on y3 =4ax is k|(t1−t2)(t2−t3)(t3−t1)| then K =
06:27View SolutionSuppose
(x+a)n=T0+T1+T2+…+Tn
Then (T0−T2+T4−…)2+(T1−T3+T5−….)2 is equal to07:09View Solutionयदि (x+a)n के विस्तार में, T0,T1,T2,…. पद प्राप्त होते है, तो (T0−T2+T4−…)2+(T1−T3+T5−….)2 बराबर होगा
04:24View SolutionIf t_(1)+t_(2)+t_(3)=-t_(1)t_(2)t_(3) , Then points [at_(1)t_(2),a(t_(1)+t_(2))] , [at_(2)t_(3),a(t_(2)+t_(3))] And [at_(3)t_(1),a(t_(3)+t_(1))] The perpendicular of the triangle with vertices will be
04:05View SolutionCoordinates of vertices of a triangle [a t_(3)t_(1)a(t_(3) +t_(1))], [a t_(2)t_(3), a(t_(2) + t_(3))] and [a t_(3)t_(1)a(t_(3) + t_(1))] Huh. The perpendicular of the triangle will be
03:24View SolutionThe base of a triangle is divided into 3-equal part. If t1,t2,t3 be the tangents of the angles subtended by these parts at opposite vertex then of (1t1+1t2)(1t2+1t3)(1+1t22)=
View Solution

