A particle starts with an initial velocity and passes successively over the two halves of a given distance with constant accelerations a1anda2 respectively. Show that the final velocity is the same as if the whole distance is covered with a uniform acceleration a1+a22.
The correct Answer is:A
To solve the problem, we need to analyze the motion of the particle in two segments, each with different accelerations, and then show that the final velocity can also be expressed using a uniform acceleration over the entire distance.
1. Define the Variables:
- Let the initial velocity of the particle be u.
- The total distance traveled by the particle is 2s (where s is the distance for each half).
- The first half of the distance s is traveled with acceleration a1.
- The second half of the distance s is traveled with acceleration a2.
2. Equation for the First Half:
- For the first half of the distance, we can use the kinematic equation:
v21=u2+2a1s
- Here, v1 is the final velocity after traveling the first half.
3. Equation for the Second Half:
- The initial velocity for the second half is v1. The kinematic equation for the second half is:
v22=v21+2a2s
- Here, v2 is the final velocity after traveling the second half.
4. Substituting v21 into the Second Equation:
- Substitute the expression for v21 from the first equation into the second equation:
v22=(u2+2a1s)+2a2s
- Simplifying this gives:
v22=u2+2a1s+2a2s=u2+2(a1+a2)s
5. Combine the Two Segments:
- The total distance is 2s. If we consider the entire distance covered with a uniform acceleration a, where a=a1+a22, we can use the kinematic equation:
v2=u2+2as
- Substituting a and the total distance 2s:
v2=u2+2(a1+a22)(2s)
- This simplifies to:
v2=u2+2(a1+a2)s
6. Conclusion:
- From both approaches, we have:
v22=u2+2(a1+a2)s
v2=u2+2(a1+a2)s
- Therefore, we conclude that:
v2=v
- This shows that the final velocity v2 is the same as if the whole distance is covered with uniform acceleration a1+a22.
- Let the initial velocity of the particle be u.
- The total distance traveled by the particle is 2s (where s is the distance for each half).
- The first half of the distance s is traveled with acceleration a1.
- The second half of the distance s is traveled with acceleration a2.
- For the first half of the distance, we can use the kinematic equation:
v21=u2+2a1s
- Here, v1 is the final velocity after traveling the first half.
- The initial velocity for the second half is v1. The kinematic equation for the second half is:
v22=v21+2a2s
- Here, v2 is the final velocity after traveling the second half.
- Substitute the expression for v21 from the first equation into the second equation:
v22=(u2+2a1s)+2a2s
- Simplifying this gives:
v22=u2+2a1s+2a2s=u2+2(a1+a2)s
- The total distance is 2s. If we consider the entire distance covered with a uniform acceleration a, where a=a1+a22, we can use the kinematic equation:
v2=u2+2as
- Substituting a and the total distance 2s:
v2=u2+2(a1+a22)(2s)
- This simplifies to:
v2=u2+2(a1+a2)s
- From both approaches, we have:
v22=u2+2(a1+a2)s
v2=u2+2(a1+a2)s
- Therefore, we conclude that:
v2=v
- This shows that the final velocity v2 is the same as if the whole distance is covered with uniform acceleration a1+a22.
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.1
2 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise 6.2
3 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Example Type 7
1 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
Starting from rest a particle is first accelerated for time t1 with constant acceleration a1 and then stops in time t2 with constant retardation a2. Let v1 be the average velocity in this case and s1 the total displacement. In the second case it is accelerating for the same time t1 with constant acceleration 2a1 and come to rest with constant retardation a2 in time t3. If v2 is the average velocity in this case and s2 the total displacement, then
View SolutionStarting from rest a particle is first accelerated for time t1 with constant acceleration a1 and then stops in time t2 with constant retardation a2. Let v1 be the average velocity in this case and s1 the total displacement. In the second case it is accelerating for the same time t1 with constant acceleration 2a1 and come to rest with constant retardation a2 in time t3. If v2 is the average velocity in this case and s2 the total displacement, then
View Solution
Knowledge Check
A body A starts from rest with an acceleration a1. After 2 seconds, another body B starts from rest with an acceleration a2. If they travel equal distances in the 5th second, after the start of A, then the ratio a1:a2 is equal to :
A0.21458333333333B0.21319444444444C0.37847222222222D0.37986111111111
A and B participate in a race with acceleration a1anda2 , respectively . A reaches t times earlier than B at finish line and their velocities at finish line are v1andv2 , respectively. If difference between their velocities is v , then find the value of v
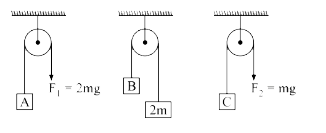
View SolutionIn the figure, blocks A, B and C of mass m each have acceleration a1, a2 and a3 respectively. F1 and F2 are external forces of magnitudes 2mg and mg respectively, then :
View SolutionIn a car race, car A takes a time t less than car B at the finish and passes the finishing point with speed v more than that of the car B. Assuming that both the cars start from rest and travel with constant acceleration a1anda2 respectively. Show that v=√a1a2t.
View SolutionIn a car race, car A takes a time t less than car B at the finish and passes the finishing point with speed v more than that of the car B. Assuming that both the cars start from rest and travel with constant acceleration a1anda2 respectively. Show that v=√a1a2t.
View SolutionThe capacitance of capacitor of plate areas A1 and A2 (A1<A2 at a distance d is

View SolutionTwo concentric conducting spherical shells of radii a1anda2(a2>a1) are charged to potentials ϕ1 and ϕ2, respectively. Find the charge on the inner shell.
View SolutionIf the area of the circle is A1 and the area of the regular pentagon inscribed in the circle is A2, then find the ratio A1A2.
View Solution
