An aeroplane has to go from a point P to another point Q, 1000 km away due north. Wind is blowing due east at a speed of 200km/h. The air speed of plane is 500km/h.
(a) Find the direction in which the pilot should head the plane to reach the point Q.
(b) Find the time taken by the plane to go from P to Q.
The correct Answer is:A, B, D
To solve the problem, we will break it down into two parts: (a) finding the direction in which the pilot should head the plane, and (b) calculating the time taken by the plane to travel from point P to point Q.
Part (a): Finding the Direction
1. Understanding the Problem:
- The plane needs to travel due north to reach point Q, which is 1000 km away.
- The wind is blowing due east at a speed of 200 km/h.
- The airspeed of the plane (the speed of the plane relative to the air) is 500 km/h.
2. Setting Up the Coordinate System:
- Let the north direction be the positive y-axis and the east direction be the positive x-axis.
3. Identifying the Velocity Components:
- The velocity of the wind (V_wind) is 200 km/h towards the east (x-direction).
- The velocity of the plane (V_plane) is 500 km/h, which we need to resolve into components.
4. Using Trigonometry:
- Let θ be the angle the plane makes with the north direction (positive y-axis).
- The northward component of the plane's velocity is V_plane * cos(θ).
- The eastward component of the plane's velocity is V_plane * sin(θ).
5. Setting Up the Equation:
- To reach point Q directly north, the eastward component of the plane's velocity must equal the wind's velocity:
Vplane⋅sin(θ)=Vwind
- Substituting the known values:
500⋅sin(θ)=200
6. Solving for sin(θ):
- Rearranging gives:
sin(θ)=200500=0.4
7. Finding θ:
- Taking the inverse sine:
θ=sin−1(0.4)
- This gives θ ≈ 23.58°.
8. Direction of the Plane:
- Since the wind is blowing east, the pilot should head the plane at an angle of approximately 23.58° west of north.
Part (b): Finding the Time Taken
1. Calculating the Effective Velocity:
- The effective velocity of the plane in the northward direction can be calculated using the Pythagorean theorem:
Veffective=√(Vplane⋅cos(θ))2+(Vwind)2
- First, calculate the northward component:
Vnorth=500⋅cos(23.58°)≈500⋅0.9239≈461.95 km/h
- The effective velocity is:
Veffective=√(461.95)2+(200)2≈√213,000+40,000≈√253,000≈503 km/h
2. Calculating Time:
- The time taken to travel the distance of 1000 km is given by:
Time=DistanceVelocity=1000 km503 km/h≈1.987 hours
Final Answers:
- (a) The pilot should head approximately 23.58° west of north.
- (b) The time taken by the plane to go from P to Q is approximately 1.99 hours.
- The plane needs to travel due north to reach point Q, which is 1000 km away.
- The wind is blowing due east at a speed of 200 km/h.
- The airspeed of the plane (the speed of the plane relative to the air) is 500 km/h.
- Let the north direction be the positive y-axis and the east direction be the positive x-axis.
- The velocity of the wind (V_wind) is 200 km/h towards the east (x-direction).
- The velocity of the plane (V_plane) is 500 km/h, which we need to resolve into components.
- Let θ be the angle the plane makes with the north direction (positive y-axis).
- The northward component of the plane's velocity is V_plane * cos(θ).
- The eastward component of the plane's velocity is V_plane * sin(θ).
- To reach point Q directly north, the eastward component of the plane's velocity must equal the wind's velocity:
Vplane⋅sin(θ)=Vwind
- Substituting the known values:
500⋅sin(θ)=200
- Rearranging gives:
sin(θ)=200500=0.4
- Taking the inverse sine:
θ=sin−1(0.4)
- This gives θ ≈ 23.58°.
- Since the wind is blowing east, the pilot should head the plane at an angle of approximately 23.58° west of north.
- The effective velocity of the plane in the northward direction can be calculated using the Pythagorean theorem:
Veffective=√(Vplane⋅cos(θ))2+(Vwind)2
- First, calculate the northward component:
Vnorth=500⋅cos(23.58°)≈500⋅0.9239≈461.95 km/h
- The effective velocity is:
Veffective=√(461.95)2+(200)2≈√213,000+40,000≈√253,000≈503 km/h
- The time taken to travel the distance of 1000 km is given by:
Time=DistanceVelocity=1000 km503 km/h≈1.987 hours
- (a) The pilot should head approximately 23.58° west of north.
- (b) The time taken by the plane to go from P to Q is approximately 1.99 hours.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - More Than One Correct6videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension7videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Objective45videos
KINEMATICS
- BOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises45videos
GRAVITATION
- BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE15videos
KINEMATICS 1
Similar Questions
An aeroplane has to go from a point A to another point B, 500km away due 30∘ east of north. Wind is blowing due north at a speed of 20m/s. The air-speed of the plane is 150m/s. (a) Find the direction in which the pilot should head the plane to reach the point B. (b) Find the time taken by the plane to go from A to B.
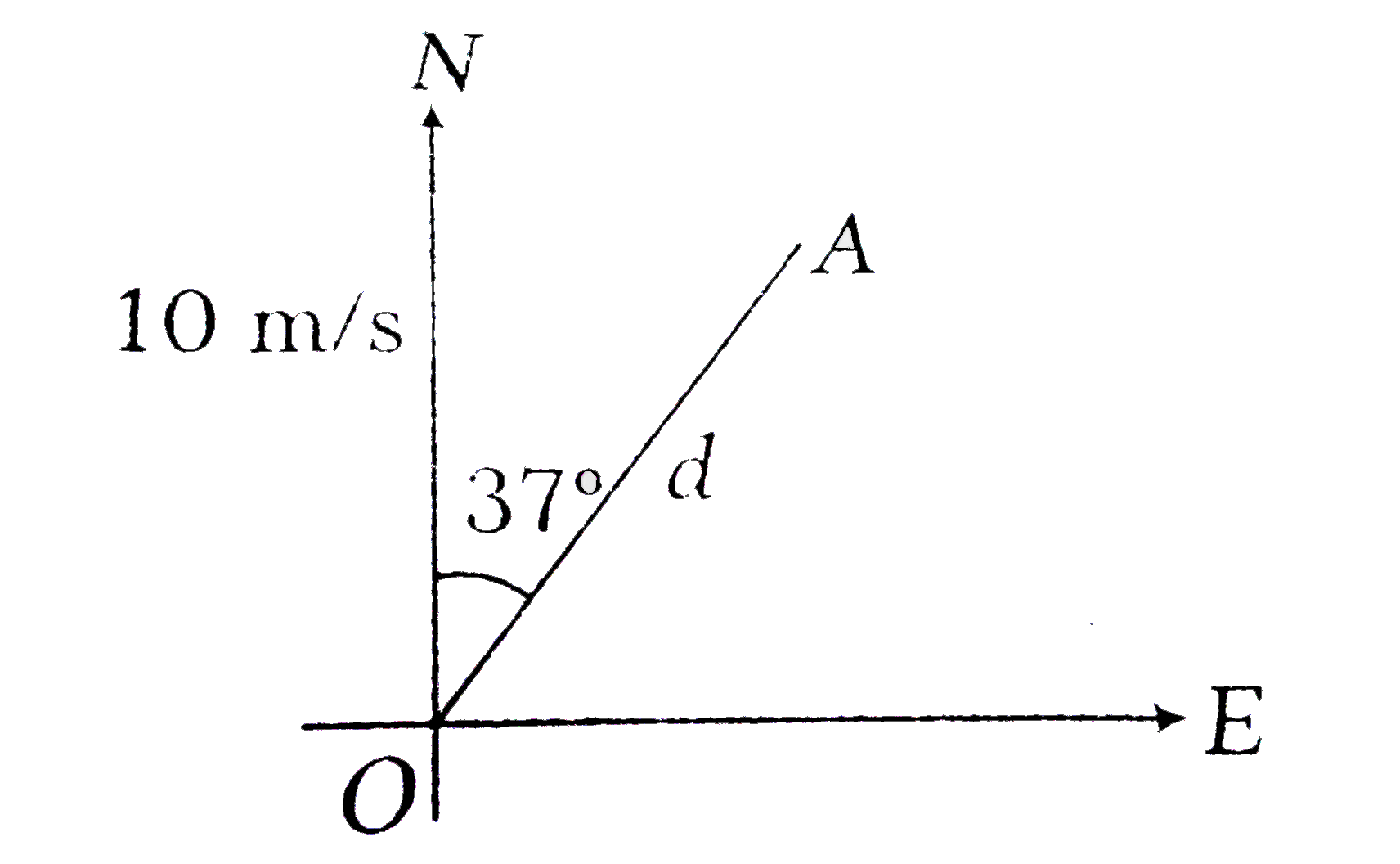
View SolutionAn aeroplane has to go from a point O to another point A, at distance d due 37∘ East of North. A wind is blowing due North at a speed of 10ms−1. The air speed of the plane is v. (i) Find the direction in which the pilot should head the plane to reach the point A. (ii) Find the time taken by the plane to go from O to A.
 View Solution
View SolutionA ship is travelling due east at a speed of 15km/h. Find the speed of a boat heading 30∘ east of north if it appears always due north from the ship.
View SolutionAn aeroplane pilot wishes to fly due west A wind of 100kmh−1 is blowing towards south.
(a) If the speed of the plane (its speed in still air) is 300kmh−1 in which direction should the pilot head ?
(b) What is the speed of the plane with respect tom ground ? Illustrate with a vector diagram.View SolutionFind the ratio in which the line segment , joining the points P(2,3,4)andQ(−3,5,−4) is divided by yz plane . Also find the point of contact .
View SolutionA man is moving due east with a speed 1 km/hr and rain is falling vertically with a speed √3 km/hr. At what angle from vertical the man has to hold his umbrella to keep the rain away. Also find the speed of rain drops w.r.t. man.
View SolutionFind the ratio in which yz-plane divides the line segment joining the points P(-1, 3,2) and Q(3, -4,5). Also find the co-ordinates of point of division.
View SolutionA political party has to start its procession in an area where wind is blowing at a speed of 30√2kmh−1 and party flags on the cars are fluttering along north - east direction. If the procession starts with a speed of 40kmh−1 towards north, find the direction of flags on the cars.
View SolutionA plane is flying at a speed of 720kmh−1 with respect to air. The wind is blowing at a speed of 54kmh−1 from west to east. With respect to ground the plane is found to be movig northwards. In which direction is the plane heading?
View SolutionFrom a light-house an observer two ships A and B. Ship A proceeding towards north at a speed 20√2 km//h and ship B proceeding towards north-east at a speed of 20 km//h. Find in which direction and at what speed the ship B would appear to move to an observer standing on the deck of the ship A.
View Solution

