A particle moves along a straight line and its velocity depends on time as v=3t−t2. Here, v is in m/s and t in second. Find
(a) average velocity and
(b) average speed for first five seconds.
The correct Answer is:A, B, C
To solve the problem, we need to find the average velocity and average speed of a particle whose velocity is given by the equation v=3t−t2 over the time interval from t=0 to t=5 seconds.
Step 1: Find the displacement over the time interval.
The displacement s can be found by integrating the velocity function with respect to time:
s=∫50vdt=∫50(3t−t2)dt
Step 2: Perform the integration.
Calculating the integral:
s=∫(3t−t2)dt=3t22−t33+C
Now, we evaluate this from t=0 to t=5:
s=(3(5)22−(5)33)−(3(0)22−(0)33)
Calculating the values:
s=(3⋅252−1253)
Calculating 3⋅252=37.5 and 1253≈41.67:
s=37.5−41.67=−4.17meters
Step 3: Calculate the average velocity.
The average velocity vavg is given by:
vavg=Total DisplacementTotal Time=st
Substituting the values:
vavg=−4.175=−0.834m/s
Step 4: Find the total distance traveled.
To find the average speed, we need to calculate the total distance traveled. First, we need to find when the particle changes direction by setting the velocity to zero:
3t−t2=0⟹t(3−t)=0
This gives us t=0 and t=3 seconds. The particle changes direction at t=3 seconds.
Step 5: Calculate the distance from t=0 to t=3.
d1=∫30(3t−t2)dt
Calculating this integral:
d1=(3(3)22−(3)33)=(272−9)=(13.5−9)=4.5meters
Step 6: Calculate the distance from t=3 to t=5.
For t=3 to t=5, the velocity is negative, so we need to calculate the distance using the absolute value of the velocity:
d2=∫53|v|dt=∫53(t2−3t)dt
Calculating this integral:
d2=(t33−3t22)53
Calculating the values:
d2=((5)33−3(5)22)−((3)33−3(3)22)
Calculating:
d2=(1253−752)−(9−272)
Calculating 1253≈41.67 and 752=37.5:
d2=41.67−37.5−(9−13.5)=4.17+4.5=8.67meters
Step 7: Calculate the total distance and average speed.
Total distance D:
D=d1+d2=4.5+8.67=13.17meters
Average speed vavg,speed:
vavg,speed=Dt=13.175=2.634m/s
Final Answers
- Average Velocity: −0.834m/s
- Average Speed: 2.634m/s
- Average Velocity: −0.834m/s
- Average Speed: 2.634m/s
Topper's Solved these Questions
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - SCQ_TYPE
39 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - MCQ_TYPE
23 Videos
- KINEMATICSBOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICSEXERCISE - Comprehension
7 Videos
- GRAVITATIONBOOK - DC PANDEY ENGLISHCHAPTER - GRAVITATIONEXERCISE - (C) Chapter Exercises
45 Videos
- KINEMATICS 1BOOK - DC PANDEY ENGLISHCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
Similar Questions
A particle moves along a straight line its velocity dipends on time as v=4t−t2. Then for first 5s:
View SolutionIf the particle is moving along a straight line given by the relation x=2−3t+4t3 where s is in cms., and t in sec. Its average velocity during the third sec is
View SolutionA particle is moving along x-axis. Its X-coordinate varies with time as, X=2t2+4t−6 Here, X is in metres and t in seconds. Find average velocity between the time interval t=0→t=2s.
View SolutionA particle is rotates in a circular path of radius 54m with varying speed v=4t2 . Here v is in m/s and t inn second . Find angle between velocity and accelearation at t=3s .
View SolutionThe motion of a particle along a straight line is described by the function x=(2t−3)2, where x is in metres and t is in seconds. Find
(a) the position, velocity and acceleration at t=2s.
(b) the velocity of the particle at origin.
View SolutionThe motion of a particle along a straight line is described by the function x=(2t−3)2, where x is in metres and t is in seconds. Find
(a) the position, velocity and acceleration at t=2s.
(b) the velocity of the particle at origin.
View SolutionA car moves along a straight line whose equation of motion is given by
s=12t+3t2−2t3
where s is in metres and t is in seconds. The velocity of the car at start will be :-
View SolutionThe velocity of an object moving rectilinearly is given as a function of time by v=4t−3t2 where v is in m/s and t is in seconds. The average velocity if particle between t=0 to t=2 seconds is
View SolutionThe velocity 'v' of a particle moving along straight line is given in terms of time t as v=3(t2−t) where t is in seconds and v is in m/s.
The distance travelled by particle from t=0 to t=2seconds is :
View SolutionVelocity-time graph of a particle moving in a straight line is shown in figure. In the time interval from t=0 to t=14s, find
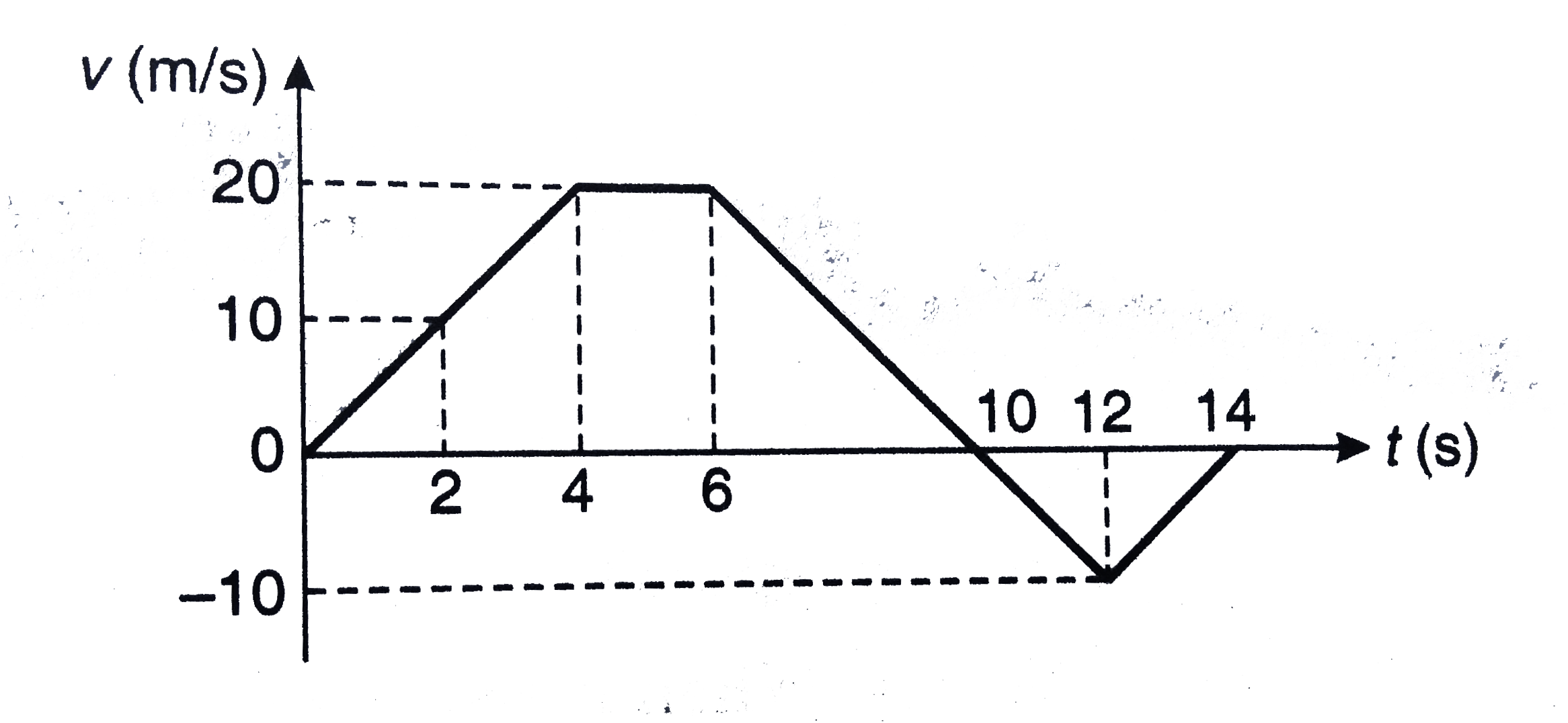
(a) average velocity and
(b) average speed of the particle.
View Solution
